MM3

Question 1

What is the general Fourier series for period 2L and what are the formulas for coefficients?

Answer 1

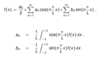
Question 2

What is Parseval’s identity for Fourier expansion between -π and +π?

Answer 2

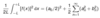
Question 3

What is meant by a regular singular point?

Answer 3

• p₀ and q₀ are both finite at x₀
  
  • what’s x₀

Question 4

How to find the inverse of matrix A?

Answer 4

• A^-1 = C^T/|A|
• C^T is the cofactor of A
  
  • like finding determinant AND with the +-+- stuff

Question 5

What is the Fourier transform f(k) of a function f(x)?

Answer 5

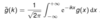
Question 6

What is the Parseval’s identity for Fourier transform?

Answer 6

Question 7

How to determine polynomial solutions?

Answer 7

• Find when series terminates
  
  • expand the series soln up to the point where the series terminates

Question 8

How to use dummy variables?

Answer 8

• Sub the power needed e.g. j in the original series e.g. j-1, replace variable in original series with j’ so j’-1 = j
• set j’=0 (or whatever the start point of the original series was) to get the new start point of the series e.g. j=-1

Question 9

What is the flux through a surface (assume closed)?

Answer 9

• n vector for any closed surface always points outwards
• e.g. magnetic flux

Question 10

How to determine if the field F through a point is a source or a sink?

Answer 10

• depends on div of F
  
  • ∇.F > 0 => source
  • ∇.F < 0 => sink

Question 11

What is the divergence theorem?

Answer 11

• outward flux of a vector field of a closed surface = volume integral of the divergence over the region inside the surface

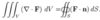
Question 12

How to evaluate line integral?

Answer 12

• see if F is parallel or perp. to dr
  
  • (dr = dr e_r)
  • if perp. then F.dr = 0
  • if parallel then F.dr = F dr
• can predict line integral using Stoke’s theorem
  
  • so for a conservative field, the integral of a closed loop is 0

Question 13

What is Stokes theorem?

Answer 13

• line integral of a loop enclosing surface Σ = surface integral of the curl of the field