Reading 27 Risk Management Applications of Option Strategies

Question 1

Call option: value at expiration, profit, maximum profit/loss, breakeven

Answer 1

For the option buyer:

c_T = max(0,S_T – X)

Value at expiration = c_T

Profit: Π = c_T – c₀

Maximum profit = ∞

Maximum loss = c₀

Breakeven: S_T* = X + c₀

Question 2

Put option: value at expiration, profit, maximum profit/loss, breakeven

Answer 2

Buying a put we have:

p_T = max(0,X – S_T)

Value at expiration = p_T

Profit: Π = p_T – p₀

Maximum profit = X – p₀

Maximum loss = p₀

Breakeven: S_T* = X – p₀

Question 3

Covered Call

Answer 3

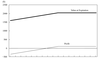
An option strategy involving the holding of an asset and sale of a call in the asset.

Value at expiration: V_T = S_T – max(0,S_T – X)

Profit: Π = V_T – S₀ + c₀

Maximum profit = X – S₀ + c₀

Maximum loss = S₀ – c₀

Breakeven: S_T* = S₀ – c₀

Question 4

Protective Put

Answer 4

Holding an asset and a put on the asset is a strategy known as a protective put.

Value at expiration: V_T = S_T + max(0,X – S_T)

Profit: Π = V_T – S₀ – p₀

Maximum profit = ∞

Maximum loss = S₀ + p₀ – X

Breakeven: S_T* = S₀ + p₀

Question 5

Money Spread option strategies

Answer 5

A spread is a strategy in which you buy one option and sell another option that is identical to the first in all respects except either exercise price or time to expiration. If the options differ by time to expiration, the spread is called a time spread.

Question 6

Bull Spreads

Answer 6

A bull spread is designed to make money when the market goes up. In this strategy we combine a long position in a call with one exercise price and a short position in a call with a higher exercise price.

To summarize the bull spread, we have:

• Value at expiration: V_T = max(0,S_T – X₁) – max(0,S_T – X₂)
• Profit: Π = V_T – c₁ + c₂
• Maximum profit = X₂ – X₁ – c₁ + c₂
• Maximum loss = c₁ – c₂
• Breakeven: S_T* = X₁ + c₁ – c_{<span>2</span>}

Bull spreads are used by investors who think the underlying price is going up.

Question 7

Bear Spreads

Answer 7

If one uses the opposite strategy, selling a call with the lower exercise price and buying a call with the higher exercise price, the opposite results occur. The graph is completely reversed: The gain is on the downside and the loss is on the upside. This strategy is called a bear spread. The more intuitive way of executing a bear spread, however, is to use puts. Specifically, we would buy the put with the higher exercise price and sell the put with the lower exercise price.

To summarize the bear spread, we have

• Value at expiration: V_T = max(0,X₂ – S_T) – max(0,X₁ – S_T)
• Profit: Π = V_T – p₂ + p₁
• Maximum profit = X₂ – X₁ – p₂ + p₁
• Maximum loss = p₂ – p₁
• Breakeven: S_T* = X₂ – p₂ + p₁

​The bear spread with calls involves selling the call with the lower exercise price and buying the one with the higher exercise price. Because the call with the lower exercise price will be more expensive, there will be a cash inflow at initiation of the position and hence a profit if the calls expire worthless.

Question 8

Butterfly Spreads

Answer 8

In both the bull and bear spread, we used options with two different exercise prices.

butterfly spread combines a bull and bear spread.

In summary, for the butterfly spread

• Value at expiration: V_T = max(0,S_T – X₁) – 2max(0,S_T – X₂) + max(0,S_T – X₃)
• Profit: Π = V_T – c₁ + 2c₂ – c₃
• Maximum profit = X₂ – X₁ – c₁ + 2c₂ – c₃
• Maximum loss = c₁ – 2c₂ + c₃
• Breakeven: S_T* = X₁ + c₁ – 2c₂ + c₃ and S_T* = 2X₂ – X₁ – c₁ + 2c₂ – c₃​

Question 9

Put–call parity

Answer 9

c = p + S – X/(1 + r)T

Question 10

Collars

Answer 10

In effect, the holder of the asset gains protection below a certain level, the exercise price of the put, and pays for it by giving up gains above a certain level, the exercise price of the call. This strategy is called a collar. When the premiums offset, it is sometimes called a zero-cost collar.

In summary, for the collar:

• Value at expiration: V_T = S_T + max(0,X₁ – S_T) – max(0,S_T – X₂)
• Profit: Π = V_T – S₀
• Maximum profit = X₂ – S₀
• Maximum loss = S₀ – X₁
• Breakeven: S_T* = S₀

Collars are virtually the same as bull spreads.

Question 11

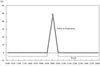
Straddle

Answer 11

Suppose the investor buys both a call and a put with the same exercise price on the same underlying with the same expiration.

Only when the investor believes the market will be more volatile than everyone else believes would a straddle be advised.

In summary, for a straddle

• Value at expiration: V_T = max(0,S_T – X) + max(0,X – S_T)
• Profit: Π = V_T – (c₀ + p₀)
• Maximum profit = ∞
• Maximum loss = c₀ + p₀
• Breakeven: S_T* = X ± (c₀ + p₀)

As we have noted, a straddle would tend to be used by an investor who is expecting the market to be volatile but does not have strong feelings one way or the other on the direction. An investor who leans one way or the other might consider adding a call or a put to the straddle. Adding a call to a straddle is a strategy called a strap, and adding a put to a straddle is called a strip. It is even more difficult to make a gain from these strategies than it is for a straddle, but if the hoped-for move does occur, the gains are leveraged. Another variation of the straddle is a strangle, in which the put and call have different exercise prices. This strategy creates a graph similar to a straddle but with a flat section instead of a point on the bottom.

Question 12

Box Spreads

Answer 12

So to summarize the box spread, we say that

• Value at expiration: V_T = X₂ – X₁
• Profit: Π = X₂ – X₁ – (c₁ – c₂ + p₂ – p₁)
• Maximum profit = (same as profit)
• Maximum loss = (no loss is possible, given fair option prices)
• Breakeven: no breakeven; the transaction always earns the risk-free rate, given fair option prices

Question 13

Interest rate options

Answer 13

The payoff of an interest rate call option is:

(Notional principal) x max (0,Underlying rate at expiration
− Exercise rate) x (Days in underlying rate/360)

If an interest rate option is used to hedge the interest paid over an m-day period, then “days in underlying” would be m.

The most important point, however, is that the rate is determined on one day, the option expiration, and payment is made m days later.

Question 14

Using an Interest Rate Cap with a Floating-Rate Loan

Answer 14

A combination of interest rate call options designed to align with the rates on a loan is called a cap. The component options are called caplets. Each caplet is distinct in having its own expiration date, but typically the exercise rate on each caplet is the same.

Question 15

Using an Interest Rate Floor with a Floating-Rate Loan

Answer 15

A combination of interest rate put options that expire on the various interest rate reset dates. This combination of puts is called a floor, and the component options are called floorlets.

Question 16

Using an Interest Rate Collar with a Floating-Rate Loan

Answer 16

Most interest rate collars, however, are initiated by borrowers.

Question 17

Delta hedge

Answer 17

• The option dealer would not want to hold a short call position for long. The ideal way to lay off the risk is to find someone else who would take the exact opposite position, but in most cases, the dealer will not be so lucky. Another ideal possibility is for the dealer to lay off the risk using put–call parity.
• Unfortunately, neither of these transactions can be commonly employed. The necessary options may not be available or may not be favorably priced. As the next best alternative, dealers delta hedge their positions using an available and attractively priced instrument. The dealer is short the call and will need an offsetting position in another instrument. An obvious offsetting instrument would be a long position of a certain number of units of the underlying. The size of that long position will be related to the option’s delta.

Delta = Change in option price / Change in underlying price

• The delta usually lies between 0.0 and 1.0. Delta will be 1.0 only at expiration and only if the option expires in-the-money. Delta will be 0.0 only at expiration and only if the option expires out-of-the-money. So most of the time, the delta will be between 0.0 and 1.0. Hence, 0.5 is often given as an “average” delta, but one must be careful because even before expiration the delta will tend to be higher than 0.5 if the option is in-the-money.
• The ratio of calls to shares has to be the negative of 1 over the delta.

N_c/N_S=−1/(Δc/ΔS)

• This illustration may make delta hedging sound simple: Buy (sell) delta shares for each option short (long). But there are three complicating issues. One is that delta is only an approximation of the change in the call price for a change in the underlying. A second issue is that the delta changes if anything else changes. Two factors that change are the price of the underlying and time. When the price of the underlying changes, delta changes, which affects the number of options required to hedge the underlying. Delta also changes as time changes; because time changes continuously, delta also changes continuously. Although a dealer can establish a delta-hedged position, as soon as anything happens—the underlying price changes or time elapses—the position is no longer delta hedged. In some cases, the position may not be terribly out of line with a delta hedge, but the more the underlying changes, the further the position moves away from being delta hedged. The third issue is that the number of units of the underlying per option must be rounded off, which leads to a small amount of imprecision in the balancing of the two opposing positions.
• A delta hedge should earn the risk-free rate. The position does not earn a “dividend” although it should increase in value gradually (at the risk-free rate). The upside potential is limited to the risk-free rate. The manager would have to constantly monitor and adjust the position to achieve the goal.
• Current exposure from selling/buying options = # contracts x spot price x option delta
• Numbers of shares for the hedge = # contracts x option delta !!!

Question 18

Delta Hedging an Option over Time

Answer 18

Two patterns become apparent:

1) The further away we move from the current price, the worse the delta-based approximation, and
2) the effects are asymmetric. A given move in one direction does not have the same effect on the option as the same move in the other direction. Specifically, for calls, the delta underestimates the effects of increases in the underlying and overestimates the effects of decreases in the underlying.

• The dealer would typically hold a position in the underlying to delta-hedge a position in the option. Trading in the underlying would not, however, always be the preferred hedge vehicle. In fact, we have stated quite strongly that trading in derivatives is often easier and more cost-effective than trading in the underlying.
• To delta hedge with options:

N₁/N₂=−Δc₂/Δc₁
* The negative sign simply means that a long position in one option will require a short position in the other. The desired quantity of Option 1 relative to the quantity of Option 2 is the ratio of the delta of Option 2 to the delta of Option 1.

Question 19

Formula for compounding a value at the risk-free rate for one day

Answer 19

Formula for compounding a value at the risk-free rate for one day is exp(r_c/365)

Question 20

Gamma and the Risk of Delta

Answer 20

A gamma is a measure of several effects. It reflects the deviation of the exact option price change from the price change as approximated by the delta. It also measures the sensitivity of delta to a change in the underlying. In effect, it is the delta of the delta. Specifically,

Gamma = Change in delta / Change in underlying price

If a delta-hedged position were risk free, its gamma would be zero. The larger the gamma, the more the delta-hedged position deviates from being risk free.

The largest moves for gamma occur when options are trading at-the-money or near expiration, when the deltas of at-the-money options move quickly toward 1.0 or 0.0. Under these conditions, the gammas tend to be largest and delta hedges are hardest to maintain.

Question 21

Vega and Volatility Risk

Answer 21

The sensitivity of the option price to the volatility is called the vega and is defined as

Vega = Change in option price / Change in volatility

Dealers try to measure the vega, monitor it, and in some cases hedge it by taking on a position in another option, using that option’s vega to offset the vega on the original option. Managing vega risk, however, cannot be done independently of managing delta and gamma risk. Thus, the dealer is required to jointly monitor and manage the risk associated with the delta, gamma, and vega.

Question 22

Differences between interest rate options and equity or bond options

Answer 22

• Bullish (bearish) equity investors buy calls (puts). In interest rate markets, bullish (bearish) investors buy puts (calls) on interest rates, because being bullish (bearish) on interest rates means that one thinks rates are going down (up).
• Interest rate options pay off as though they were interest payments. Equity or bond options pay off as though the holder were selling or buying stocks or bonds.
• Finally, interest rate options are very often combined into portfolios in the form of caps and floors for the purpose of hedging floating-rate loans. Standard option strategies such as straddles and spreads are just as applicable to interest rate options.