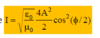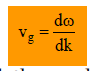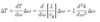Optical Fibres Flashcards
Features of ITU G.652 Single Mode Fibre
0.2db/km Loss @ 1550nm
Mode Diameter 9-10um
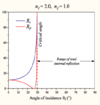
Cut Off Wavelength 1000 to 1280nm
Dispersion 0.17ps/(nm*km) @ 1550nm
Bend Loss @ 1550nm <1db for 100 turns at 75nm radius
Two General Steps In Fabrication Of Optical Fibres?
Preform Fabrication, then
Fibre Drawing
What Are the Primary Considerations In Preform Fabrication?
Low Water Content
High Purity
Precise Dimensions
Refractive Index Control.
What is the main method of preform Fabrication?
Silica gas Deposition
Other than silica gas deposition what other methods can be used for preform fabrication?
Liquid Melting,
Rod in Tube used for short device
Casing.
What are the three types of silica gas deposition?
Vapour Axial Deposition
Outside Vapour depostion
Modified Chemical Vapour Deposition
Describe Basic Chemistry of Silica Deposition (gas phase)
Volatile Halide Compounds formed from distillation of carrier gas through liquid halide are doped.
The mixture is Oxidised,
Deposition Occurs on to Substrate, Dopant Conc can be varied for graded index and step Index
Features of Vapour Axial Deposition (VAD)
Used Commonly for Transmission Fibres
Low Loss
Suitable for Multimode and Single mode Fibres
Large Preform so Suitable for Long Fibres
High-Speed Deposition
Continuos Production Possible
Describe VAD deposition
This is a process that uses end-on deposition onto a rotating fused silica target. The vaporized constituent substances are injected from burners and react to form silica soot via flame hydrolysis.
Silica soot is much less dense than silica and is deposited onto the end of the target in an axial direction to form a solid preform in a shape similar to a ball.
As the preform grows it is pulled upwards at a rate dependent on the growth rate.
It is first dehydrated using chloride and then sintered into a solid preform.
Important features of the VAD process
Soot is deposited as particles with a typical diameter of < 0.1um.
Soot is much less dense than silica by around a factor of ten,
Dopants are often not formed as particles in the flame, they condense onto silica particles
Particles impact the preform due to the thermophoretic force
Consolidation is the process of sintering soot into a solid
Consolidation can be performed online or offline in a furnace.
The atmosphere is controlled during consolidation, chlorine may be used to remove water via HCL and oxygen.
Define sintering
The process of compacting and forming a solid mass of a substance via pressure or heat, without heating to the point of liquefication.
Define the thermophoretic force
Thermophoresis is the force generated due to the temperature gradient between the hot gas and cold wall, in this case preform.
What diameter are preforms to be drawn to
100mm dia preforms are drawn to around 1/8mm dia fibres
Features of OVD
Batch Process, preform can be drawn for several kilometre fibres, suitable for core or claaing, Allows fine control of graded index for high bandwidth products, purity dependent of purity of feeding materials and OH impurity from flame.
In addition the mandrel when removed may lead to cracks in surface of the perform.
Process of MCVD?
- Pure oxygen and halide vapour flows though a high purity silica tube.
- A heat source (usually a H2/O2flame) heats a small section of the tube.
- In the hot region the halides are oxidised.
- Thermophoresis∗drives the silica particles to adhere to the tube.
- As the heat source passes over the soot it is consolidated.
- Multiple layers are deposited, each with carefully controlled dopant concentration giving accurate control of refractive index profile.
- The tube is collapsed to a solid rod by heating to more than 2000°C, such that the surface tension overcomes the viscosity and the tube collapses.
Features of MCVD
- Unlike VAD or OVD the process is conducted within a tube in the absence of hydrogen–thus allowing very low OH contamination without the lengthy drying
- Very versatile process giving highly precise control of the refractive index profile
- Widely used for speciality fibre production.
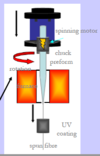
Describe Fibre Drawing
• ‘Drop’ to start the process
• Capstan speed controlled in a feedback loop from diameter gauge
• Fibre loss and strength can be affected by drawing
– Diameter fluctuation
• Draw temperature & tension
• Purge gas turbulence
– Flaws
• Contamination
• Damage
– Poor coating
• Coating –physical and chemical protection.
– Optical properties key for double-clad fibres
– Acrylate -UV crosslinked, high or low index
– Silicone –thermally cured, low index
• Can spin preform to reduce birefringence (up to 2000 rpm)
Concerns when Fibre Drawing
You must be mindful of potential contamination of the fibre, and in turn, introduce some losses.
Define free space velocity
Velocity of light in free space:
𝑐 =𝑓𝜆0 =1/ sqrt(𝜇0𝜖0) ≈ 3×108𝑚/𝑠
Define refractive index
𝑛 = sqrt(𝜖𝑟)
(remember that permittivity is a strong function of frequency)
Define Phase Velocity
The velocity of light in a medium of refractive index n: 𝑣𝑝=𝑓𝜆=1/sqrt(𝜇0𝜖0𝜖𝑟)
Define Angular Frequency
Angular frequency: ω (radians/second)
Define Wavenumber
Wavenumber: 𝑘=2𝜋/𝜆=𝜔 *sqrt(𝜇0𝜖0𝜖𝑟)
(if free space then εr= 1 and we use k0 and λ0)
Equation For Plane Wave
A plane wave can be expressed in the form
𝑬=𝑨𝑒𝑗𝜔t−𝑘z
How are the magnitudes of a plane wave and electric field related?
𝐸=|𝐻| sqrt(𝜇/𝜖)
The plane wave will also have a magnetic field orthogonal to both the E-field and z
Wave Impedance
Wave impedance:
𝑍=sqrt(𝜇/𝜖).
The wave impedance of free space is 𝑍0=sqrt(𝜇0𝜖0)=377Ω.
Intensity of Light
The intensity (irradiance) is:
𝐼=|𝐸0|2/2𝑍.
Where E0 is the peak electric field.
Describe Modulation Steps
- Sample time-varying analogue signal (at baseband sampling rate must be ≥ 2fmax)
- Quantisethese samples giving rise to quantisation noise
- Express each sample as a binary number
- Send these bits followed by the bits for the next sample down your channel
- Do the reverse of this at the end of the system, but there will be errors due to noise
Can a channel transmit DC easily? Why?
Channels often don’t transmit “DC”, in other words, a continuous train of “1”s or a continuous train of “0”s, very well.
What is coding?
Coding is the science of replacing the original sequence with another to match the data to the channel, often it includes adding bits for error detection and correction as well.
Considerations affect the receiver
Information on the timing of pulses
Error Detection and Correction
Why does the receiver need information on the timing of pulses?
So that it can align its clock and sample the received digital signal at the right points in time. Timing information is obtained by locking a clock oscillator at the receiving end to the transitions on the signal. If there are few transitions, the clock timing information is lost and sampling and reconstructing the signal is impossible. Appropriate coding ensures the probability of too few transitions is vanishingly small.
What modulation format is used by transmission systems?
Typically use OOK or on-off keying
From OOK, the system may use the return to zero(RZ) format, or the non-return to zero(NRZ) format.
What is the return to zero( RZ) Format?
In RZ, the signal returns to the value representing zero for the second half of the bit time slot.
The duty cycle of the signal may not be 0.5, i.e. divided into the second and first half. The division may be more like 1/3 and 2/3.
What is non-return to zero (NRZ)?
In NRZ, the signal keeps its value for the whole time slot.
Benefits of RZ
RZ has simpler clock recovery at the receiver as the data stream contains the clock frequency
Benefits of NRZ
NRZ uses less bandwidth and gives the detector more photons/bit -important for detectability
Is the clock transmitted with the data?
A clock is not transmitted with the data –it has to be recovered at the receiver.
Describe Power Spectrum of NRZ data stream
Peak at centre, smaller peaks surround it similar to normal function.
The envelope of Power Spectrum has a sine shape
- Matches Fourier transform of a square pulse
Extend to infinity
How are pulses transmitted in DWDM systems?
We don’t attempt to transmit square pulses, we pre-filter to reduce bandwidth and avoid cross-talk between channels in DWDM systems
Can modulated carriers be single frequency?
A carrier might be single-frequency (monochromatic in optics) but a modulated carrier cannot be single frequency.
What effect does dispersion have on transmission?
Dispersion will result in pulse-spreading and thus intersymbol interference, unless compensated
Why does signal dispersion lead to pulse spreading?
Dispersion results in pulse-spreading because refractive index and hence velocity varies with wavelength/frequency
A real data stream must contain a band of frequencies, so the pulses spread.
Define eye diagram
An eye pattern, also known as an eye diagram, is an oscilloscope display in which a digital signal from a receiver is repetitively sampled and applied to the vertical input, while the data rate is used to trigger the horizontal sweep. Wikipedia
Why are eye diagrams useful?
They sho pictorially and quantitatively, the constraints on deciding whether a received bit is 1 or 0 in the presence of attenuation, noise, jitter, pulse-spreading etc.
How are eye diagrams read?
A properly constructed eye should contain every possible bit sequence from simple alternate 1’s and 0’s to isolated 1’s after long runs of 0’s and all other patterns that may show up weaknesses in the design.
The larger the opening the more reliably a design in. And less affected by errors..
Describe eye diagram early in the channel
- Pulses are not square because that would need a lot of unnecessary bandwidth
- They are broad in the vertical (detector current) direction because of noise and the electronic response to different numbers of 1’s or 0’s in succession
- They are broad in the width (time) direction because of timing jitter
Describe eye diagram later in the channel
- Attenuation, noise, more jitter, and pulse-spreading have further closed the eye
- In presence of noise, the decision between 0 and 1 is more prone to an error (poor S/N)
- The timing of the decision is more critical
- Ultimately for a long channel or a high bit rate the eye closes and the system doesn’t work
What is jitter?
Jitter occurs when riding or falling edges occur at times that differ from the ideal time. Some edges occur early, some occur late. In a digital circuit, all signals are transmitted in reference to clock signals. The deviation of the digital signals as a result of reflections, intersymbol interference, crosstalk, PVT (process-voltage-temperature) variations, and other factors amounts to jitter. Some jitter is simply random.
What effect does jitter have?
“Jitter” causes errors in pulse timing with the clock, yet again increasing the probability of an error
What effect does attenuation have on the accuracy of transmission?
Attenuation (loss) reduces the distinction between the level of a “1” and a “0” so that receiver noise has an increased probability of causing an error
How do you deal with attenuation, dispersion and jitter?
These three phenomena need remediating in a repeater after some length of fibre–amplification, pulse reshaping and pulse retiming
Define interference in fibres
A range of phenomena associated with the superposition of waves
What is the equation for the intensity of two waves interfering with each other?
intensity will be
I = sqrt(ε0/μ) *(4A2 /2)cos(φ/2)
Describe what happens when two waves with different k and ω superpose?
Total E-field is :
E = Ae-j(k1z -ω1t) + Ae-j(k2z - ω2t)
Which may be rearranged to:
E = 2Ae-j(kz-ωt)cos(Δkz - Δωt)
where k =( k1+k2)/2 and Δk = (k1-k2)/2 same for ω and Δω
Thus becomes a plane wave with velocity ω/k modulated by a cos wave of frequency Δω.
Cos wave travels with a velocity of Δω/Δk
In turn, the group velocity is
Vg = dω/dk.
Define group velocity of the envelope of modulated carrier waves
Define equation for vg in terms of vp
Derive vg in terms of Vp
vg = d𝜔/dk,
1/vg = d/d𝜔[𝜔/c * n(𝜔)]
as k = 2π/𝜆 n and 𝜆 = (2πc)/𝜔 so k = (𝜔/c)*n
Differentiating with respect to 𝜔
1/vg = 1/c[n(𝜔) + 𝜔dn/d𝜔]
We recognise the first term as being equal to 1/vp and rearrange the 2nd term in terms of λ:
𝜔dn/d𝜔 = 2πc/𝜆[dn/d𝜆 * d𝜆/d𝜔] = -2πc/𝜆* 2πc/𝜔2* dn/d𝜆 = -[2πc/𝜔]2 * 1/𝜆 *dn/d𝜆 = -𝜆(dn/d𝜆)
Such that:
1/vg = 1/c[n(𝜆) - 𝜆dn/d𝜆] = 1/vp -(𝜆/c)dn/d𝜆
Give the equation for the refractive index in terms of relative permittivity
n = sqrt(εr)
How does vg vary in dispersive materials?
In dispersive materials (where velocity changes with wavelength) the dependence of the refractive index of the material leads to vg ≠ vp.
This means the modulation envelope does not travel at the same speed as the carrier
What effect does a difference in vg and vp have on the transmission?
Pulses travelling at a different velocity to vp doesn’t mean they spread, just that the delay in transmission (latency) is different.
Does a difference between vg and vp lead to pulse broadening?
Not by itself, however, if the group velocity is in a dispersive medium, i.e. frequency dependent, then pulse spreading will occur.
This is because each frequency component of a pulse travels at a different group velocity and so they disperse and arrive at different times at the end of the fibre.
What is the equation for pulse broadening due to a difference between vg and vp?
Give equation for latency
Latency is approximated using the equation (D = T * V) where D is the distance the fibre travels, T is the time it takes to travel, and V is the velocity of light.
How can vg and GVD be controlled?
Material dispersion and waveguide dispersion is used to do so.
What effect does pulse broadening have?
Pulse-broadening leads to inter-symbol interference, increasing the error rate
It occurs as modulated carriers have a non-zero bandwidth.
What is the equation for reflection in a dielectric interface?
The equation for reflection coefficient for E-field in the plane of incidence
n1 is the refractive index of the medium from which the wave is incident
n2 is the refractive index on the other side of the dielectric interface
α is the angle of incidence
The equation for reflection coefficient for E-field normal to the plane of incidence
n1 is the refractive index of the medium from which the wave is incident
n2 is the refractive index on the other side of the dielectric interface
α is the angle of incidence
How can different states of polarisation be expressed?
All states of polarisation can be expressed by superposing E// andE⊥ in different amplitudes and phases.
What conditions are required for total internal reflection where light is incident from a high index medium on a low index medium
Total internal reflection occurs (|ρ|=1) if α>αc
What is the core and cladding indices for a typical optical fibre?
A typical optical fibre has a core index of 1.4579 and a cladding index of 1.4469 at λ=1560nm
What does R represent in optical fibres?
R is the power reflection coefficient (R=|ρ|2) and Rp≡R// and Rs≡ R⊥
What happens when sinα < n2/n1?
We have reflection and transmission
What happens when sinα = n2/n1?
we get total reflection (for which n1 > n2)
What happens if sinα > n2/n1?
The angle of transmission is complex
The equation for cosβ in terms of refractive index
What happens to cosβ when then (n1/n2)2sin2α > 1
Then α>αc or sinα > n2/n1
This means cosβ is an imaginary angle. Which suggests exponentially decaying or possibly exponentially growing waves in the z-direction in the low-index medium.
What effect does an imaginary angle indicate?
Exponentially growing or decaying waves in the low index medium.
Phase of a transmitted Wave
φt = k2(xsinβ + zcosβ)
The phase of a transmitted wave in terms of incident angle and complex costs
The electric field in a lower-index medium
What are the terms in the exponent of the electric field?
- Phase as a function of time: tω
- Phase in the x-direction along the interface: kn1 xsinα
- Complex phase in the z-direction, representing a decaying wave if we choose –ve
The complex phase is the evanescent field.
What is the evanescent field?
The evanescent field is the complex phase in the z-direction of the electric field.
What is brewster’s angle?
Brewster’s angle is an angle of incidence at which light with a particular polarization is perfectly transmitted through a transparent dielectric surface, with no reflection.
How does the reflection coefficient vary as the angle of incidence changes, when light is incident from a low index medium on a high index medium.
As can be observed from the figure, the reflection coefficient for the Rs polarisation(perpendicular) increases exponentially as the angle of incidence increases.
Total internal reflection only occurs when the angle of incidence is 90 degrees.
What is the speed of light in a medium of permittivity ε and permeability μ?
Is vp = 1/sqrt(εμ)
where ε = ε0 εr similar rule for μ
What is Rayleigh scattering?
Rayleigh scattering is due to microscopic fluctuations in the density of the glass (in the case of glass fibre), frozen in place when the glass cools. These random fluctuations in the refractive index scatter light and it is found that the loss is related to a Rayleigh scattering coefficient by:
𝛼𝑅 = c/𝜆4
where C is found to be between 0.7 and 0.9 dB.km-1.μm4 depending upon the fibre composition.
What are the primary loss mechanisms for optical fibres
Rayleigh Scattering, Impurities such as OH group, and IR absorption
What is material absorption?
Material absorption refers to a process where photons are absorbed prematurely preventing light transmission.
Absorption can be described by Beer’s Law,
Pout/Pin = 10-εL
where ε is the extinction coefficient in units such as ppm-1 km-1 (per ppm per km)
c is the concentration of the impurity in ppm
L is the pathlength of the absorbing material in km
What causes material absorption?
Material absorption is due to impurities in the glass, and the fundamental absorption of the pure silica glass network.
Express absorption in terms of refractive index
We take an expression for a wave propagating in a medium of refractive index n in the +z direction
E(t, z) = E0 exp{jtωt - jk0nz}
Now we take n to be complex i.e. n’ + jn’’
E(t,z) = E0 exp{jωt - jk0n’z}exp{k0n’‘z}
How is the imaginary part of n (n”) related to the loss α(dB/km)?
The wave decays according to 𝐸(𝐿)=𝐸0exp{𝑘0𝑛”𝐿} 𝑜r 𝑃(𝐿)=𝑃(0)exp{2𝑘0𝑛”𝐿}
Loss in dB is given by
𝛼 =−10log10|P(0)/P(L)|
so that
𝛼= -10log10(e)2k0n”L
What is the V Number?
V is a form of normalised frequency
The equation is below:
What is the normalised propagation coefficient?
b must be between 0 and 1 because,
𝑛22eff212
β normally indicates propagation coefficient where 𝛽=𝑁𝑒ff𝑘0
What happens when b = 0?
When b = 0 the velocity is that of a wave in the cladding and the mode becomes cut-off. It doesn’t ever quite get there for the fundamental mode, so there is no cut-off for the fundamental mode.
What happens when b = 1
When b = 1 the mode travels with the velocity of a wave in the core material. There is always some evanescent field in the cladding, so b never quite gets to unity.
