Geometry (Circles Part. 1) Flashcards
Circle
The locus of all points equidistant from a central point.
Tangent
A straight line or plane that just touches a curve at one point.
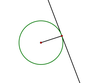
Secant
A line that intersects the curve in at least two (Distinct) points.
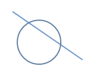
Chord
A line segment connecting two points on a curve.
Minor Arc
An arc of a circle whose measure is less than 180°.
*Usually represented by only two letters “Arc (AB)”
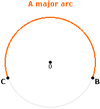
Major Arc
An arc of a circle whose measure is greater than 180°.
*Usually represented by three letters “Arc (ABC)”
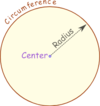
Radius
(Definition)
A straight line from the center to the circumference of a circle or sphere.
Diameter
(Definition)
Any straight line segment that passes through the center of the circle and whose endpoints lie on the circle.
Radius (From Diameter)
Equation
r = d/2
Diameter (From Radius)
Equation
d = 2r
The Diameter of a circle is 16 units.
What is the Radius of the circle?
r = (d)/2
r = (16)/2
r = 8
Radius = 8 Units
The Radius of a circle is 2 units.
What is the Diameter of the circle?
d = 2(r)
d = (2)2
d = 4
Diameter = 4 Units
What is the Radius and Diameter of the following circle?
Radius = 8 ft
d = 2(r)
d = 2(8)
d = 16
Diameter = 16 ft
What is the Diameter and Radius of the following circle?
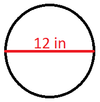
Diameter = 12 in
r = (d)/2
r = 12/2
r = 6
Radius = 6 in
Circumference
(Definition)
The distance around a circle.
π (PI)
(Definition)
The ratio of the Circumference of a circle to its Diameter (3.142)
π (From Circumference and Diameter)
(Equation)
π = c/d
A circle has a circumference of 907.46 units and a diameter of 289 Units.
What is the ratio of the Circumference to the diameter?
π = c/d
π = 907.46/289
π = 3.14 Units
π (From Circumference and Radius)
(Equation)
π = c/2r
A circle has a Circumference of 50.24 Units and a Radius of 8 units.
What is the ratio from the Circumference to the diameter?
π = c/2r
π = 50.24/16
π = 3.14
Circumference (From Diameter and π)
(Equation)
c = dπ
Suppose the diameter of a circle is 6 units.
What is the Circumference?
c = dπ
c = (6)π
c = 18.85
Circumference = 18.85 Units
Circumference (From Radius and π)
(Equation)
c = 2πr
Suppose the radius of a circle is 3 units.
What is the Circumference?
c = 2π(r)
c = 2π(3)
c = 6π
Circumference = 18.85 Units
Diameter (From π and Circumference)
(Equation)
d = c/π
What is the diameter of the circle below?
d = (c)/π
d = 10/π
d = 3.18
Diameter = 3.18m
Radius (From π and Circumference)
(Equation)
r = c/2π
A circle has the circumference of 153.86 units.
What is the radius of the circle?
r = (c)/2π
r = (153.86)/6.28
r = 24.5
Radius = 24.5 Units
Arc Measure
(Definition)
The measure of the central angle that intercepts an arc, measured in degrees.
What is the Arc Measure, in degrees, of arc (AC) on circle P below?
174°
In the figure below, line (AD) and line (CE) are diameters of circle P.
What is the measure of Arc (AEB) in degrees?
305°
What is the Arc Measure of (YZ) in degrees?
59°
In the figure below, line (AD) and line (BE) are diameters of circle P.
What is the Arc Measure of (CAD) in degrees?
296°
