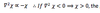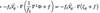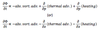chapter 4 Flashcards
The QG Thermodynamic Equation
This equation can be derived from
the thermodynamic energy equation in pressure coordinates
the following is the
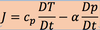
the thermodynamic energy equation in pressure coordinates
describe each term in the equation
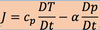
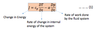
cp and a in the following equation means
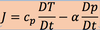
describe the following terms in the equation
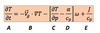
this is called
static stability parameter
is a positive number for a stable atmosphere, and a negative number for an unstable atmosphere.
the following is the
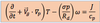
the quasi geostrophic (QG) thermodynamic energy equation in pressure coordinates
in this equation the …………………. is simply substituted for ……………………………..
geostrophic wind is simply substituted for the actual wind in the advection term
the following terms are
the above equation states that
temperature change at a particular location and height is a function of temperature advection by geostrophic wind and vertical motion.
Warm temperature advection result in
cold temperature advection result in
Ascent vertical motion result in
Decent vertical motion result in
Diabatic effects:
Diurnal (day and night) l heating/cooling plays a major role in temperature changes near the surface
(5) is the
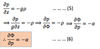
hydrostatic equation in height coordinates (x, y, z)
(6) is the
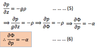
the hydrostatic equation in pressure coordinates
the geopotential tendency
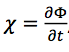
the following is the
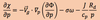
QG thermodynamic energy equation
Note that equation 5 and 7 are identical, which are written in different forms, because
in a hydrostatic atmosphere, do Φ⁄do p is proportional to the temperature (T) of the layer.
the following is the
QG vorticity equation
The geopotential tendency equation is derived from
The QG Thermodynamic Energy Eq.
QG Vorticity Eq.
For a sinusoidal disturbance having a zero mean value, the
horizontal Laplacian of a field is proportional to the negative of the field
……………………………………………………………., the horizontal Laplacian of a field is proportional to the negative of the field
For a sinusoidal disturbance having a zero mean value
geopotential tendency is positive
the following is the
absolute vorticity advection
PVA =
NVA
when heights are rising T-adv. increases with
pressure
when heights are rising T-adv. decreases with
height
when heights are falling T-adv. decreases with
pressure
when heights are falling T-adv. increases with
height
Strong CA over ………………. has the same effect as ……………… over ………………..
weak CA
weak WA
strong WA
It is the vertical derivative of the advection that matters. Strong CA over weak CA has the same effect as weak WA over strong WA, because in both cases the
derivative has the same value
The differential heating term (third term on RHS) behaves similarly to the
differential thermal advection term.
That is, the Term-C is proportional to
he vertical derivative of diabatic heating
when heights rise:
heating
cooling
Heating decreases with height
Cooling increases with height
when heights fall:
heating
cooling
Heating increases with height
Cooling decreases with height
Another useful way of writing the essence of the Q-G tendency equation is in qualitative form, as:
Thus, in quasi-geostrophic theory, there are only three ways for heights to
fall
Thus, in quasi-geostrophic theory, there are only three ways for heights to fall. These are through:
- Positive Vorticity Advection
- WA that increases with height
- Diabatic heating that increases with height
Le Chatelier’s Principle, states that
many natural systems will resist changes, and if forced to change, will react with process that try to restore the original state.
We can see Le Chatelier’s principle at work in
the differential thermal advection and diabatic heating terms of the Q-G tendency equation
For example, cold advection (or …………………….) over warm advection (or ………………………….) forces
diabatic cooling
diabatic heating
height rises at 500 mb, as well as height falls at 200 and 1000 mb
A
200 mb
B
500 mb
C
1000 mb
D
cold advection
E
Warm avection
A
200 mb
B
500 mb
C
1000 mb
D
E
these height rises and falls indicate that there must be a change in
the vorticity at these levels
However, these height rises and falls indicate that there must be a change in the vorticity at these levels:
- increased vorticity where there are height falls, and
- decreased vorticity where there are height rises
To accomplish this vorticity change in a …………………….. framework
quasi-geostrophic
To accomplish this vorticity change in a quasi-geostrophic framework, there must be
convergence where there are height falls, and divergence where there are height rises.
The convergence/divergence pattern leads to:
- upward motion and adiabatic cooling in the lower levels, and
- subsidence and adiabatic warming in the upper levels.
The adiabatic heating/cooling thus, opposes the
original temperature change due to advection.
Le Chatelier’s Principle doesn’t mean that
the effects of the differential heating (advection) will be completely cancelled by the adiabatic heating/cooling from the secondary circulation, but does illustrate that the atmosphere will resist the changes imposed by the thermal forcing, and will respond with a secondary circulation.
