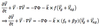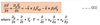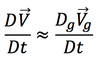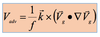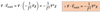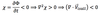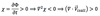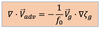chapter 3 Flashcards
The large scale flow in the atmosphere is nearly
geostrophic
the large scale flow in the atmosphere is nearly geostrophic
the ………………. and …………………. are …………………..
wind and mass fields are virtually in balance
the large scale flow in the atmosphere is nearly geostrophic
the wind and mass fields are virtually in balance
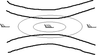
In such an atmosphere, the isobars are
straight and parallel and the density is a function of pressure alone (barotropic)
If geostrophy is assumed, there is
no vertical motion and there is no change in the spatial patterns of the height of isobaric surfaces.
If geostrophy is assumed, there is no vertical motion and there is no change in the spatial patterns of the height of isobaric surfaces.
Hence, geostrophy cannot be used to
understand the development of weather systems
Hence, geostrophy cannot be used to understand the development of weather systems, which requires
time changes in the spatial patterns of the height of isobaric surfaces
………………………………………. can be used to understand the development of weather systems in ……………………………….
Quasi-geostrophic theory
a baroclinic atmosphere
In a baroclinic atmosphere, the
surfaces of constant pressure and density intersect each other forming solenoids that leads to direct circulation and vertical motion.
In a baroclinic atmosphere, the surfaces of constant pressure and density intersect each other forming solenoids that leads to direct circulation and vertical motion.
In such an atmosphere,
the spatial pattern of height field changes with time.
Hence, the slight deviation of geostrophy (quasi-geostrophic) is of great importance to
understanding of atmospheric flow and weather systems
The Quasi-Geostrophic (QG) Momentum Equations
For this analysis, it is convenient to use the
isobaric coordinate system
For this analysis, it is convenient to use the isobaric coordinate system because
- meteorological measurements are generally referred to constant pressure surfaces and
- the dynamical equations are somewhat simpler in isobaric coordinates than in height coordinates.
The scalar and vector horizontal momentum equations in (x,y,z) coordinate system can be written as:
The scalar and vector horizontal momentum equations in (x,y,z) coordinate system can be written as:
where
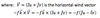
Equation (1) can be transferred to
x,y,p coordinates
Equation (1) can be transferred to (x,y,p) coordinate system as follows:
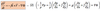
LHS of Eq.(2) can be expanded as follows:
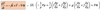
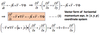
The QG Momentum Equations
Consider the momentum equation in vector form (Eq.3)
The QG Momentum Equations
after considering the momentum equation in vector form
now you should
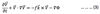
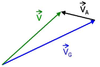
Split the horizontal wind into geostrophic and ageostrophic components
The QG Momentum Equations
after concidering the momentum equation in vector form
plitting the horizontal wind into geostrophic and ageostrophic components:
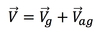
The QG Momentum Equations
- Consider the momentum equation in vector form (Eq.3):
- Splitting the horizontal wind into geostrophic and ageostrophic components:
the next step is
Substituting (4) in the coriolis term in (3)
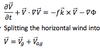
The QG Momentum Equations
- Consider the momentum equation in vector form (Eq.3):
- Splitting the horizontal wind into geostrophic and ageostrophic components:
Substituting (4) in the coriolis term in (3):
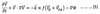
Midlatitude ß-plane approximation
To retain the dynamical effect of the variation of the Coriolis parameter with latitude, f can be approximated by expanding its latitudinal dependence in a Taylor series about a reference latitude O| o
The QG Momentum Equations
- Consider the momentum equation in vector form (Eq.3):
- Splitting the horizontal wind into geostrophic and ageostrophic components:
- Substituting (4) in the coriolis term in (3):
The next step is
Now, we replace the Coriolis parameter by f= f0 +ßy , so that the momentum equations (Eq.5) become:
The QG Momentum Equations
- Consider the momentum equation in vector form (Eq.3):
- Splitting the horizontal wind into geostrophic and ageostrophic components:
- Substituting (4) in the coriolis term in (3):
- Now, we replace the Coriolis parameter by f = f0 + ßy , so that the momentum equations (Eq.5) become:
the next step is
Expanding the RHS of Eq.(6)
The QG Momentum Equations
- Consider the momentum equation in vector form (Eq.3):
- Splitting the horizontal wind into geostrophic and ageostrophic components:
- Substituting (4) in the coriolis term in (3):
- Now, we replace the Coriolis parameter by f = f0 + ßy , so that the momentum equations (Eq.5) become:
- Expanding the RHS of Eq.(6)
the next step is
The last term in (7) is very small, and can be ignored, so we now have:
The QG Momentum Equations
- Consider the momentum equation in vector form (Eq.3):
- Splitting the horizontal wind into geostrophic and ageostrophic components:
- Substituting (4) in the coriolis term in (3):
- Now, we replace the Coriolis parameter by f = f0 + ßy , so that the momentum equations (Eq.5) become:
- Expanding the RHS of Eq.(6)
- The last term in (7) is very small, and can be ignored, so we now have
but the next step is
By the definition of the geostrophic wind
The QG Momentum Equations
- Consider the momentum equation in vector form (Eq.3):
- Splitting the horizontal wind into geostrophic and ageostrophic components:
- Substituting (4) in the coriolis term in (3):
- Now, we replace the Coriolis parameter by f = f0 + ßy , so that the momentum equations (Eq.5) become:
- Expanding the RHS of Eq.(6)
- The last term in (7) is very small, and can be ignored, so we now have
- By the definition of the geostrophic wind
the next step is
Substituting (9) in (8), the first two terms on the right-hand side of (8) cancel, resulting in
The approximate QG horizontal momentum equation thus has the form:
The rate of change of momentum following the total motion is approximately equal to
the rate of change of the geostrophic momentum following the geostrophic wind
The rate of change of momentum following the total motion is approximately equal to the rate of change of the geostrophic momentum following the geostrophic wind:
……………………………………………………………………… is approximately equal to the rate of change of the geostrophic momentum following the geostrophic wind
The rate of change of momentum following the total motion
As discussed earlier, though the atmosphere is close to being in geostrophic balance, the unbalanced component of the wind (the ageostrophic wind) is very important for
the dynamics of synoptic disturbances
Now, we derive an equation for the ageostrophic wind. For this, consider the
simplified horizontal momentum equations
Now, we derive an equation for the ageostrophic wind. For this, consider the simplified horizontal momentum equations:
Thus, the ageostrophic wind, which is the
deviation of the real wind from the geostrophic wind,
Thus, the ageostrophic wind, which is the deviation of the real wind from the geostrophic wind, is mathematically expressed as:
The ageostrophic wind is a measure of the
horizontal acceleration
As shown in figure, the ageostrophic wind points to the
left of the geostrophic acceleration (in NHS)
the ageostrophic wind figure
the bold line is representing
the height contour
the thin line represents
isotachs
the wind barbs represent
the geostrophic wind
the figure represents
a typical jet streak
ageostrophic wind is to the left of the geostrophic acceleration, the ageostrophic wind will be oriented as
the ageostrophic wind is divergent in
the right entrance and left-exit regions of the jet streak
the ageostrophic wind is convergent in
the other regions
the ageostrophic wind is divergent in the right entrance and left-exit regions of the jet streak, and convergent in the other regions
This leads to
upward motion in the right-entrance and left-exit regions and downward motion in the other two regions of the jet streak.
The Isallobaric Wind – Vector Form
step 1
Expanding the total derivative on the RHS of Eq.12:
Thus the forcing of the ageostrophic wind can be divided conveniently into the two parts, the
isallobaric wind and the advective wind.
the isallobaric wind is writen as
The scalar components of the isallobaric wind can be obtained as:
from the equation of isallobaric wind
is determined by the
gradient of the isolines of do p/do t
what are the isolines
These are the lines connecting the equal amounts of surface pressure change (isallobars)
These are the lines connecting the equal amounts of surface pressure change (isallobars). In figure, we have:
The direction of the isallobaric wind is
perpendicular to the isallobars
The direction of the isallobaric wind is perpendicular to the isallobars, always pointing
towards the falling pressure
The direction of the isallobaric wind is perpendicular to the isallobars, always pointing towards the falling pressure (i.e., pointing to
the minimum value where the strongest pressure decrease) in surface pressure is located.
the second term on the RHS of is the
advective term
The scalar components of the advective wind can be written as:
The advective wind arises when
the geostrophic wind is not uniform, as in diffluent or confluent flow pattern.
In diffluent flow pattern (fig), the geostrophic wind
decreases in positive x-direction
In diffluent flow pattern (fig), the geostrophic wind decreases in positive x-direction due to
the larger spacing between the isobars indicating smaller pressure gradient.
In the analogous case of a confluent flow the wind speed will
increase
The divergence of the isallobaric wind is:
Divergence of the isallobaric wind
When heights are falling:
the isallobaric wind is ……………………..
convergent
Divergence of the isallobaric wind
When heights are rising:
the isallobaric wind is ……………………….
divergent
The divergence of the advective wind can be obtained as:
where عg is the ………………………….. and Vg.-/عg is the …………………………….
where عg is the geostrophic vorticity and Vg.-/عg is the vorticity advection
When there is Positive Vorticity Advection (PVA):
the advective wind is ………………
divergent
When there is Negative Vorticity Advection (NVA):
the advective wind is ……………………..
convergent
