W6 - Circulation (2.6) Flashcards
What are the functions of the circulatory system?
transport of
- nutrition, O2, CO2, metabolites
- thermoregulation (= transport of heat)
- hormones (= endocrinological function)
- cells of immune system (= immunological function)
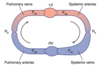
What is the two-pump system?
Explain.
simplified model for cardiovascular system
- 2 pumps in series: right, left ventricle
- 2 vascular beds in series: systemic, pulmonary vasculature
→ flow pumped in both systems virtually equal to each other
Elaborate on the following parameters of the aorta:
- diameter, thickness of wall
- amount of elastic tissue
- amount of smooth mm.
- number
- cross sectional area [cm2]
- percentage of blood volume
Elaborate on the following parameters of arteries:
- diameter, thickness of wall
- amount of elastic tissue
- amount of smooth mm.
- number
- cross sectional area [cm2]
- percentage of blood volume
Elaborate on the following parameters of arterioles:
- diameter, thickness of wall
- amount of elastic tissue
- amount of smooth mm.
- number
- cross sectional area [cm2]
- percentage of blood volume
Elaborate on the following parameters of capillaries:
- diameter, thickness of wall
- amount of elastic tissue
- amount of smooth mm.
- number
- cross sectional area [cm2]
- percentage of blood volume
Elaborate on the following parameters of venules:
- diameter, thickness of wall
- amount of elastic tissue
- amount of smooth mm.
- number
- cross sectional area [cm2]
- percentage of blood volume
Elaborate on the following parameters of veins:
- diameter, thickness of wall
- amount of elastic tissue
- amount of smooth mm.
- number
- cross sectional area [cm2]
- percentage of blood volume
Elaborate on the following parameters of the vv. cavae:
- diameter, thickness of wall
- amount of elastic tissue
- amount of smooth mm.
- number
- cross sectional area [cm2]
- percentage of blood volume
Elaborate on the following parameters of the vessels of the pulmonary circulation:
- amount of elastic tissue
- amount of smooth mm.
- percentage of blood volume
What percentage of blood volume can be found in the heart?
7%
→ approx. 350 - 450 ml
Although hemodynamics are a powerful tool to describe physics of blood/fluid flow through the circulatory system, they are not 100% exact.
Why is that?
bc in vessels
- flow is pulsatile: pressure P, flow Q are not constant
- wall is not rigid due to el. tissue: radius r, cross sectional area A can be changed
- blood is a non-newtonian fluid: velocity v is not always constant
Explain the relationship btw velocity and the cross-sectional area.
equation of continuity
conservation of mass causes fluid flow to be constant in every 2 sections of a tube
→ velocity inversely proportional to cross-sectional area
Q = A * v
A * v = constant
BUT: same applies if A2 is spread among many vessels
Relate the velocity-cross-sectional area relationship in a graph.
- velocity decreases in arterial system
- minimal value in capillaries
- velocity increases in venous system
What does the lowest point of the velocity graph indicate?
Value?
lowest v (= 0.3 mm/s) in capillaries → **longest time (1 - 3s) for diffusion btw circulatory system and peripheral tissues**
What are the types of pressure in a tube?
- static pressure Pstat: pressure if fluid is not moving or if object is moving w/ the fluid dynamic pressure Pdyn: pressure of a fluid that results from its motion
→ Pstat + Pdyn = Ptotal
lecture guy referred to static pressure as side pressure
How can dynamic pressure be calculated?
dynamic pressure is directly proportional to density and velocity
Pdyn = 1/2 ⍴ v2
- ⍴ = density
- v = velocity
BUT: usually negligible in most arterial locations
Describe the effect of velocity on static pressure in a tube.
Which law describes this relationship?
- *Bernoulli’s law** states that if the v is increased a decreased Pstat can be measured
- *↑v → ↓Pstat**
because:
- ↑v → ↑Pdyn
- Pstat + Pdyn = Ptotal
- conservation of E
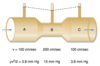
Which relationship is described by Hagen-Poiseuille’s law?
Formula.
describes flow of fluid in terms of
- ΔP (Pi - Po) = pressure gradient btw inlet and outlet
- r = radius of the tube
- ƞ = viscosity of the fluid
- l = length of the tube
NOTE: radius is the critical factor
(raised to the 4th power)
Give the formula for hydraulic resistance in fluid mechanics.
ability of vessels to fluid flow
R = ΔP/Q
- ΔP = pressure gradient btw inlet and outlet of tube
- Q = flow
⇒ resistance is inversely proportional to fluid flow
How can the resistance of vessels be calculated using Hagen-Poiseuille’s law?
Formula + unit.
Hagen’s Poiseuille’s law can be plugged in to formula for hydraulic resistance to substitute fluid flow, pressure gradient can be cancelled out
in [R unit] (instead of mmHg*sec/ml)
- ƞ = viscosity of fluid
- l = length of tube
- r = radius of tube
NOTE: radius is the critical factor
(raised to the 4th power)
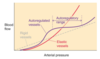
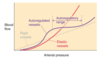
What is the consequence of Hagen-Poiseuille’s law w/r/t the resistance in various blood vessels?
depends mainly on vessel diameter
- highest resistance in capillaries
- diminishes as vessels incr. in diameter on art./ven. sides of capillaries
Name 2 mechanisms that can change the diameter of a vessel.
- contraction of circular smooth mm. in the vessel wall
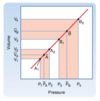
- transmural pressure = pressure difference btw int./ext. pressure, hence ↑Pint w/o incr. Pext → dilation of vessel
How can resistances in series and parallel be calculated?
in series: RT = R1 + R2 + R3 + …
in parallel: 1/RT = 1/R1 + 1/R2 + 1/R3 + …
⇒ total resistance = less than individual resistances bc additional pathways for fluid flow are provided
NOTE: RT = total peripheral resistance
Although capillaries are usually arranged in parallel with one another, there are 2 exceptions.
Which ones?
- renal vasculature: peritubular capillaries are in series w/ glomerular capillaries
- splanchnic vasculature: intestinal are in series w/ hepatic capillaries
What is total peripheral resistance?
Formula and unit.
What is the consequence?
ratio of arteriovenous pressure difference to cardiac output (according to ΔP = Q * R), reciprocal of 1/RT
TPR = Patrial/cardiac output
→ if CO constant, change in TPR can modify Patrial
What does the Reynold’s number describe?
Formula.
describes the tendency of flow to be laminar or turbulent
- NR > 2000 → laminar
- NR > 3000 → turbulent
NR = ⍴dv/ƞ
- ⍴ = density of fluid
- d = diameter of vessel
- v = mean velocity of fluid
- ƞ = viscosity
Describe how the velocity of laminar flow behaves.
most central layer moves most rapidly (double mean velocity of flow across entire cross section)
bc thin layer of fluid adhering to wall/slower layer → shear force, slowing down next, more central layer
What is the significance of turbulent flow?
How can it be caused?
greater pressure required to force a given flow through same tube (higher workload on heart)
+ causes sounds
- anemia → ↓ƞ → ↑NR
- stenosis → ↓A → ↑v → ↑NR
- BP measurement → ↓A → ↑v → ↑NR
AND: thrombi are more likely to develop in turbulent flow
What is shear stress?
Formula.
blood flow through a vessel causes a force on the wall parallel to the wall = shear stress
τ = 4ƞQ/πr3
- ƞ = viscosity of the fluid
- Q = fluid flow
- r = radius of the vessel
NOTE: radius is the critical factor
(raised to the 3th power)
Which properties of the vasculature are described by the Young-Laplace equation?
Why is it clinically relevant?
relates the wall tension to the shape of the surface
T = P*r/2x
- P = internal pressure
- r = radius of the vessel
- x = wall thickness
in case of aneurysm: incr. r → ↑T → ↑r → ↓x
→ ↑↑T → vessel eventually bursts
What does the distensibility of a vessel describe?
Formula.
ability of a vessel to change its volume under transmural pressure → elastic properties
D = (ΔV/V0)/P in [%]
- ΔV/V0 = ratio of volume change and initial volume
- P = transmural pressure
What is the difference btw compliance and distensibility of a vessel?
Formula.
Compare the compliance of veins and arteries.
- distensibility refers to volume change as a ratio to its initial volume
- compliance only describes the relative volume change (= slope of PV diagram)
C = ΔV/ΔP
- ΔV = volume change
- ΔP = pressure difference
Compare compliance and distensibility of systemic, pulmonary veins and arteries in a graph.
Explain.
compliance = slope of PV diagram
- Dveins = 8x Darteries
- Cveins = 25x Carteries
- Cpulm > Csyst
although arteries have much greater amount of elastic tissue than veins, veins are usually not fully distended
→ can alter shape from ellipsoid to circular
BUT: only in physiological range
(“true” D, Cveins < D, Carteries)
What are standard physiological pressure and volume changes in the venous and arterial system?
venous system:
- ΔP = 15 mmHg
- ΔV = 2500 ml
arterial system:
- ΔP = 100 mmHg
- ΔV = 500 ml
List and briefly explain different ways to measure the blood flow.
- bloody method: collecting venous outflow, timing the collection w/ a stopwatch
- electromagnetic flow meter: most frequently used, based on electromagnetic induction principle
- Doppler flow meter: measuring linear velocity
⇒ invasive
- Fick’s method: X added to blood, rate at which X passes a checkpoint measured + calculated
- dilution method: dyes used to measure organ’s circulation
⇒ non-invasive
List the 2 types of vessels w/r/t their conducted blood flow in response to changes in pressure.
Compare their characteristics in a graph.
- elastic vessels (= lung-type vessels) = “normal” vessel type
- autoregulated vessels (= kidney-type vessels) = able to adapt their behavior
Explain the behavior of elastic vessels referring to the graph below.
↑P → ↑r (dilation) → ↓R
⇒ exponential curve, tangent of each point would show decreased R
Explain the behavior of autoregulated vessels referring to the graph below.
Where can we find such vessels?
vascular smooth m. cells contract autonomously in response to mechanoreceptors to maintain local blood flow within a narrow range → active process
-
↑P → ↓r (contraction) → ↑R
avoids waste of perfusion into organs in which flow is already sufficient -
↓P → ↑r (relaxation) → ↓R
maintains capillary flow + capillary pressure
⇒ can be found in organs sensitive to ischemia, hypoxia (brain, kidney, heart)
(in physiological P range, 50 - 150 mmHg)
How is the radius of arterioles regulated?
- myogenic resting tone: caused by autoregulation
- sympathetic tone: caused by symp. innervation via α1 and β2 receptors
BUT: tissue dependent
Compare the hematocrit dependence of the relative viscosity of blood in vivo and in vitro.
Values for rel. viscosity of plasma at 0 and physiological Ht.
relative viscosity increases with increasing Ht level,
BUT: in vivo lower viscosity due to moving RBCs
(RBCs move faster than blood, hence lower proportion)
- blood plasma at 0% Ht, ƞ = 1.2ƞwater
- blood plasma at 45% Ht, ƞ = 3ƞwater
REMEMBER: bridge analogy
What is shear thinning?
Explain its effect on RBCs.
the greater the flow, the greater shear stress
(cf. shear stress)
→ RBCs accumulate in most central lamina, hence greatest velocity (only in vessels < 300μm)
⇒ enhances deformability of RBCs, able to fit through 3μm capillaries although 7μm in size
↑ [fibrinogen] incr. deformability of RBCs
How does the viscosity in the different types of vessels behave?
shear thinning in vessels (cf. own card)
→ lowest viscosity in smallest vessels
What is the function of the aorta and other large arteries?
Explain.
convert intermittent pulsatile cardiac output to a steady flow = Windkessel effect
- distend when the blood pressure rises during systole
- recoil when the blood pressure falls during diastole
since Qentering > Qleaving (due to the TPR)
⇒ net storage of blood during systole (40ml) which discharges during diastole
Draw and explain the pressure curve of the aortic system.
average heart rate = 75/min → heart beat = 0.8s
- systole = 0.27s (ends w/ incisura), phase of isometric contraction, rising P (80 → 120 mmHg)
- diastole = 0.53s, dropping P back to 80 mmHg
- incisura = small pressure drop due to closure of aortic valve
List the different types of blood pressure and explain.
Values.
- Psyst = 120 mmHg, highest point of curve
- Pdias = 80 mmHg, lowest point of curve
- Ppulse = 40 mmHg = ΔPdias, syst
-
Pmean = 93 mmHg = mean arterial pressure, in resting conditions Psyst/Pdias = 1:2,
BUT: heart-rate dependent: if ↑HR 1:1
List ways to measure the blood pressure.
- transducers
- sphygmomanometry
Explain the blood pressure measurement using a sphygmomanometer.
Another name.
**_Riva-Rocci method_** inflatable cuff (+ stethoscope)
- wrapped around arm, a. brachialis occluded
- pressure slowly declines
- 2 methods:
- palpatory method: pulse of a. radialis can be felt at wrist (= systolic pressure)
- auscultatory method: 2 Korotkoff sounds can be heard (1st = systolic, 2nd = diastolic)
Explain transducer measurements to determine the blood pressure.
catheter connected to a closed chamber w/ a diaphragm, amplifier, recorder
- catheter introduced into vessel
- diaphragm converts mech. strain into an electrical signal
- signal amplified + recorded
Distinguish btw parameters that determine arterial blood pressure.
physiological parameters
- cardiac output (stroke volume * HR)
- TPR
⇒ act through physical parameters
physical parameters
- blood volume
- compliance
What is the reason for the Windkessel effect in the aorta and large arteries?
Show how it changes during life using a graph.
high elastin content → high compliance
BUT: as people age elastin content reduced, replaced by collagen → reduced compliance
⇒ ↑ Psyst, ↓ Pdias, ↑↑Ppulse (cf. own card)
Describe the effect of changed stroke volume on the blood pressure, presumed that HR and arterial compliance remain constant.
Relate it in a graph.
volume that enters art. system exceeds volume that leaves system
- ↑↑ Psyst
- ↑ Pdias
- ↑ Ppulse
- ↑ Pmean
Describe the effect of changed TPR on the blood pressure, presumed that arterial compliance remains constant.
However since arterial compliance is only linear in young people, describe its effects in the elderly.
Relate it in a graph.
in young:
- ↑ Psyst
- ↑ Pdias (proportionally to ↑Psyst)
- constant Ppulse
- ↑ Pmean
in elderly: sim. effects to ↑ stroke volume
- ↑↑ Psyst
- ↑ Pdias (NOT proportional)
- ↑ Ppulse (bc improportional incr. of Psyst and Pdias)
- ↑ Pmean
left graph young, right graph elderly
Describe the effect of changed arterial compliance on the blood pressure.
When does it happen?
Relate it in a graph.
compliance decreases as people age, hence as elastin is successively replaced by collagen
→ incr. workload on left ventricle
- ↑ Psyst
- ↓ Pdias
- ↑↑ Ppulse
- constant Pmean
Describe the effect of changed blood volume on the blood pressure.
↑ blood volume → ↑ stroke volume (cf. own card)
Describe the effect of changed viscosity on the blood pressure.
↑ Ht → ↑ TPR (cf. own card)
Describe the effects of
- gender
- climate
on arterial blood pressure.
List other factors, too.
- gender: estrogen → vasodilation → ↓ TPR
- climate: heat → thermoregulation → ↓ TPR
- exercise
- sleep
- emotions
Describe the effect of gravity on large arteries, both in recumbent and upright position.
heart = reference level, zero height
95 mmHg in aorta
recumbent: no need to compensate hydrostatic pressure bc everything on heart level
- 90 mmHg in head + feet (ΔP drives blood flow)
upright: Δh heart-head = 50 cm, and 130cm (feet)
- 185 mmHg in feet (gravity → P added)
- 53 mmHg in head (gravity → P substracted)
red curves
Describe the effect of gravity on large veins, both in recumbent and upright position.
heart = reference level, zero height
2 mmHg in right atrium
recumbent: no need to compensate hydrostatic pressure bc everything on heart level
- 5 mmHg in head + feet (ΔP drives blood flow)
upright: Δh heart-head = 50 cm, and 130cm (feet)
- 100 mmHg in feet (gravity → P added)
- -32 mmHg in head (gravity → P substracted)
blue curves
Compare the effect of gravity on vascular beds in head and feet, both in recumbent and upright position.
different absolute pressures in head and feet
BUT: same ΔP driving pressure, 85 mmHg
