Regression Flashcards
(21 cards)
The formula for a Least Squares Regression Line (LSRL)
y = a + bx a is the y intercept b is the slope
Interpreting Correlation
Strength, Direction and Form
Explanatory Variable
x, input, independent
Response Variable
y, output, dependent
Scatterplot
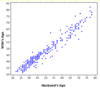
Regression Outlier
f
Independent Variable
One event has no effect on the other
Dependent Variable
Two events have effects on eachother
LSRL

Positive Association
As x increases, y increases
As x decreases, y decreases
Positive correlation

Negative Association
As x increases, y decreases
As x decreases, y increases
Negative correlation
Correlation
“r”
As r-value becomes closer to 1, the correlation becomes stronger

Coefficient of Determination
r²
written as a decimal/percentage (% of the change in y is explained by the change in x)
Regression Line
ŷ = a + bx
“line of best fit”
Residual
observed - expected (y-ŷ)
Slope
“b” value in ŷ=a+bx
y-intercept
“a” value in ŷ=a+bx
Residual Plot
A plot representing the x values and residual values (y-ŷ)
Population
Entire group of individuals to be studied
Sample
Subset of studied population
SRS
Simple Random Sample


