Enzyme Activity & Rate Equations

Question 1

What happens to the concentration of single substrate to single product reaction as it progresses?

Answer 1

substrate declines and products increase in a linear fashion

Question 2

define an equation for the general velocity of a single reactant to single product reaction

Answer 2

-∆[S]/∆t because substrate is decreasing, equals ∆[P]/∆t because product is increasing at the same rate

Question 3

What is the effect of additonal concentration of enzymes on product formation?

Answer 3

Linear increase, each enzyme of the same type reacts at the same pace

Question 4

As concentration of [S] increases with constant concentration of [E], how does the reaction proceed?

Answer 4

V changes in a hyperbolic (non-linear) fashion.

At low [S], velocity is linear as all enzymes are not yet bound

At high [S], activity levels off due to enzyme saturation. No additional [S] can be converted until existing enzymes turn over S into P

Question 5

V_max

Answer 5

V_max = k₂[E]_t

All enzymes are bound and turning over substrate to product (fully saturated)

Maximal rate of product formation

Direct effect on turnover number (k_cat = V_max/[E]_t)_, the number of S molecules converted to P molecules per enzyme per unit time

Because all enzymes are bound to substrate during V_max, [E]_t = [ES], so the first-order rate-limiting step k₂[ES] is equivilent to k₂[E]_t which is equivilent to V_max

Question 6

What is the mathematical expression for velocity of unimolecular reaction: A ⟶ B?

Answer 6

Rate of the decrease of [A] over time. Directly proportional to amount of reactant, rate constant ( k ) is first-order

Question 7

What is the mathematical expression for velocity of bimolecular reaction: A + B ⟶ C?

Answer 7

Rate of the decrease of [A] or [B] over time (equivalent because of the stoichiometry of the reaction, 1 A with 1 B). Directly proportional to amount of reactant, rate constant ( k ) is second-order because velocity depends on two reactants

Question 8

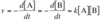
What are the assumptions made in Michaelis-Menten kinetics?

Answer 8

Stoichiometry of S and P are 1:1

k₂ is irreversable and the rate limiting step

Steady-state conditions in which the enzyme is saturated with substrate, so that once ES ⟶ E + P, the free enzyme is immediately re-bound to substrate reforming the ES complex, so that there is essentially no free enzyme in solution.

Question 9

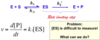
What is the mathematical expression for velocity of a Michaelis-Menten reaction: E +S ⇌ ES ⟶ E + P?

Answer 9

Velocity is the rate at which we increase the concentration of product over time

Question 10

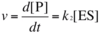
What is the limitation in measurement that Michaelis-Menten kinetics attempts to overcome?

Answer 10

We can measure total amount of substrate [E]_t, but [ES] concentration is difficult to measure in the lab, so determining d[P]/dt = k₂[ES] for the reaction E + S ⇌ ES ⟶ E + P is not possible. Because we assume that k₂ is irreversible, we can measure [ES] indirectly from the formation of ES ( rate₁ = k₁ [E][S] ) less the depletion of ES ( rate_-1 = k_-1 [ES] and rate₂ = k₂ [ES] ).

Question 11

Explain how Michaelis-Menten kinetics determines the velocity of the reaction

E + S ⇌ ES ⟶ E + P

Answer 11

Velocity of the reaction equals change in product over change in time. We have already determined that our reaction is a first-order reaction dependent on k₂ and concentration of ES. But if we cannot measure ES, how can we determine rate of reaction?

v = d[P]/dt = k₂[ES] ?

Because we assume that k₂ is irreversible, we can measure [ES] indirectly from the formation of ES ( rate₁ = k₁ [E][S] ) less the depletion of ES ( rate_-1 = k_-1 [ES] and rate₂ = k₂ [ES] ).

Steady-state conditions are those in which the enzyme is saturated with substrate, so that once ES ⟶ E + P, the free enzyme is immediately re-bound to substrate reforming the ES complex. There is essentially no free enzyme in solution, so

d[ES]/dt = 0

meaning that ES concentration does not change. Because the concentration of ES does not change over time due to the steady-state assumption, k₁ [E][S] - k_-1[ES] - k₂[ES] = 0.

The concentration of unbound enzyme must be the remainder of the total amound of enzyme less that bound in ES complex, so [E]_t-[ES] = [E].

• k*₁ ([E]_t-[ES])[S] - k_-1[ES] - k₂[ES] = 0.
• k₁ ([E]_t-[ES])[S] = [ES] (k*_-1 + k₂)

(k_-1 + k₂)/k₁ = K_M

[E]_t[S] - [ES][S] = [ES]K_M

[E]_t[S] = [ES][S] + [ES]K_M

[E]_t[S] = [ES] ([S] + K_M)

([E]_t[S])/([S] + K_M) = [ES]

We now have a formula for the concentration of ES, which allows us to determine the velocity of our first-order reaction with respect to our rate-limiting step, k₂[ES]

Because all enzymes are bound to substrate during V_max, [E]_t = [ES], so the first-order rate-limiting step k₂[ES] is equivilent to k₂[E]_t which, being our rate-limiting step, is equivilent to V_max

Question 12

Explain how [E], [ES], [S], and [P] change graphically over time in a steady-state reaction.

Answer 12

Question 13

When plotting initial velocity vs. substrate concentration what kind of plot must you get?

Answer 13

A hyperbola

Question 14

Explain the meaning of K_M in relation to substrate affinity

Answer 14

K_M is a ratio between the rate ES disappears and the rate ES forms.

If an enzyme has a high affinity for its substrate, k₁ will increase, lowering K_M.

Low K_M = high affinity

High K_M = low affinity

The faster the velocity of the reaction, the sooner 1/2 V_max is reached, and the smaller the value of K_M

Question 15

Turnover number

Answer 15

k_cat = V_max/[E]_t

Turnover number is the number of S molecules converted to P molecules per enzyme per unit time (measured in s^-1). A normalised number that allows for comparison between different enzymes at any concentration.

Question 16

Explain the meaning of K_M beyond the dissociation constant

Answer 16

K_M = (k_-1 + k₂)/k₁ = k_-1/k₁ + k₂/k₁ = K_d + k₂/k₁

Because of the additional term k₂/k₁, K_M is not simply a measure of dissociation constant (substrate affinity).

Question 17

Describe the relationship between K_M and V_max

Answer 17

When substrate concentration is equal to the Michaleis-Menten ES dissociation equilibrium ( [S] = K_M ), velocity of the reaction is half-maximal ( 1/2 V_max )

Question 18

What is the Michaelis-Menten dervied expression for velocity (V_o) of a enzyme reaction E + S ⇌ ES ⟶ E + P?

Answer 18

Question 19

Explain K_M in relation to substrate affinity

Answer 19

If an enzyme has low K_M, it will have high substrate affinity and bind its target even at low substrate concentrations.

On a high level, K_M is a measure of the amount of substrate required for significant catalysis to occur. If substrate concentration drops below K_M, not much product over time.

Often, K_M provides an approximation of substrate concentration in vivo ( [S] ≤ K_M )

Question 20

k_cat

Answer 20

k_cat = V_max/[E]_t

Turnover number is the number of S molecules converted to P molecules per enzyme per unit time (measured in s-1). A normalised number that allows for comparison between different enzymes at any concentration.

Question 21

k_cat/K_M

Answer 21

A measure of catalytic efficiency, as k_cat (k₂ or rate-limiting step at very high [S]) increases or K_M (ES complex binding disociation constant) decreases, efficiency increases k_cat/K_M becomes greater

Question 22

Lineweaver-Burk equation as it relates to graphing

Answer 22

1/V_o vs. 1/[S]

Double reciprocal of Michaelis-Menten equation

y-intercept is inverse of V_max (1/V_max)

x-intercept is negative inverse of K_M (-1/K_M)

slope is K_M/V_max

Question 23

Lineweaver-Burk y-intercept is…

Answer 23

inverse of maximum velocity (1/V_max)

Question 24

Lineweaver-Burk x-intercept is…

Answer 24

negative inverse of ES complex dissociation constant (-1/K_M)

Question 25

Lineweaver-Burk slope is…

Answer 25

ES complex dissociation constant over maximum velocity (K_M/V_max)

Question 26

Limitations of the Lineweaver-Burk plot

Answer 26

Data points not evenly distributed, because the graph measures inverse substrate concentration, the smallest measurement (lowest concentration, so also least accurate) is assigned the highest value and potential cause of error.