EK Physics Ch3 Fluids Flashcards
(86 cards)
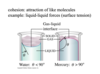
density
p= mass/ volume
so it is defined as how much mass the fluid contains in a specifid volume V
SI units are kg/m3
unless otherwise stated, assume that all liquids and solids are totally incompressible adn for this reason have constant density, in reality gases are much mroe ocmpressible than liquids and liquids are far more compressible than solids.
-gases change volume and therefore density as ideal gas law describes, PV=nRT
density formula
=how much mass per volume
= p (row) = mass/volume so density is kg per meter 3

specific gravity
SG = psubstace/p water
a specific gravity of a substance is the density of that substance divided by density of water
-less than one indciates a substace lighter than water, one indicates a substance equally as heavy was water, greater than one indicates a substance heavier than water
density of water
p= 1000 Kg/m3
p= 1 g/cm^3
fluid pressure
p= F (force)/ A (area)
fluid pressure
pressure experienced by object as a result of the impulse of molecular collisions
- it is the average of the magnitudes of the change in momentum of these collisions divided by the time duration of the collisions and the area over which these collisions occur
si unit = pascal, Pa
PRESSURE IS SCALAR, it has no direction!
- it exists in a fluid whether or not an object is immersed in that fluid
- its a type of stored energy per unit volume, units of pressure are equivalent to energy per unit volume, according to this definition, pressure is a measure of the energy due to the random velocities of molcules within a fluid distributed over the volume of the fluid
pressure in kidneys
- kidneys are responsible for keeping blood pressure within a narrow range, to maintain this delicate balance, the kidneys modify glomerular filtration rate (GFR) through renin-angiotensin-aldosterone system RAAS
- released renin converts angiotensinogen to angiotensin I and angiotensin converting enzyme ACE converts angiotensin I to angeiotensin II
- angiotensin II signals the contraction of the efferent arterioles leading away from teh glomerulus
- when teh efferent arterioles contract, blood pressure in the glomerular capillary increases because resistance in these vessels is increased
fluid at rest equation
p= pgy
p=density of the fluid
g= gravity 9.8
y= depth of the fluid or height
fluid at rest
- experiences only forces perpendicular to its surface
- at any given depth hte pressure is equal to teh weight of hte fluid above a disk with area A divided by teh deare of the disk
- pressure is the same regardless of what area is chosen!
total pressure at rest
ptotal= pgy1+p2gy2+p3gy3+p4gy4
additional fluids on top of the first fluid inc the total pressure by adding their weight, so sum the pressures
air is fluid so must add atm pressure if any fluid open to the atm, pressure can be found by p=pgy + patm
atmospheric pressure
patm= 101,000 Pa
liquids boil when vapor pressure equals atm pressure** since atm pressure is inversely proportional to altiude, it is easier for a solution to boil at higher altitudes***
pascal’s principle
- pressure applied anywhere to an enclosed incompressible fluid will be distributed equally/ undiminished throughout that fluid
- this is true becuase if pressure gradient exists btw two points with the same vertical distance from teh surface, fluid flows in the direction that relieves the differential
- since fluid pressure is a function of depth, the shape of the container does not affect it
- the pressure everywhere at a given depth in the same resting fluid will be constant
ex air pressure
think of atm as a sea of air, as mov closer to the top and climb a mountain, your depth decreases
near the top there are fewer molecyules abov eyou, which means less weight and lower pressure
air pressure up here is low, making it tough to breathe
gauge pressure
- refers to amount by which a system’s pressure deviates from atm
- P abs= p gauge + p atm
- pabsolute is hte pressure of a system relative to a vacuum
- pressure in chest for ex is lower than atm pressure
- the higher pressure of the atm pushes air into your lungs, same thing happens when suck straw, you create a partial vacuum inside the straw
- when you suck, vacuum doesnt really suck anything into it. The atm pressure pushes down on the fluid outside the straw pushing up the fluid inside the straw
- without atm pressure, a straw would not work!= remember in real life, physic never sucks
gauge pressure 2
difference btw atm pressure and absolute pressure
hydraulic lift

hydraulic lift 2
- two pistons and a container enclose a standing incompressible fluid, a force on piston 1 applies a pressure on the fluid
- presure is transferred undimished to piston 2
- piston 2 has a greater area (look page 67) than piston 1, and force and area are directly proportional when pressure is constant, the force on piston 2 is proportionally greater than the force on piston 1, even though the presure is the same
- pressure applied on a fluid must be the same throughout
- a machine does not change work, if piston 2 moed the same distance as piston 1 while also experiencing greater force, the work done by piston 2 would be greater than the work done by piston 1
- instead hte force of piston 2 applied through a proportionally smaller distance, keeping work the same
hydraulic lift 3
- notice that piston 1 has a much smaller area than does piston 2
- by pascal’s principle, both pistons must exert the same pressure at the point of contact with the liquid
- even if my pedal applies a relatively small force, its being applied to a simiarly small area
- the ratio of force to area will stay the same for piston 2, which with a larger area must be subject to a larger force
force on fluids at rest
buyoant force
buoyant force
net force acting upwards = weight of water beign displaced is archimedes principle
net upward force due to fact more pressure on bototm then top is buoyant force! what makes things float!
- on any object is floarting or sunk in teh fluid
- pressure inc as a function of depth within a fluid at rest, p=pgy, so pressure is a measure of force per unit area, P= F/A
- so for a given cross sectional area A, an inc in pressure must be associated with an inc in force
- both pressure and force inc with depth, causing an object in teh fluid to experience both greater pressure and greater force at points farther from the fluid’s surface.
- As a result, the object experiences an upward force!
- upward force is called buoyant force becuase it has potential to buoy the object - to cause it to float
Fb equation
Fb= p fluid X V fluid X g
Fb analysis
- magnitude of the buoyant force experienced by an object, whether floating, submerged or sunk is directly proportional to the volume of the fluid it displaces, where p is density of fluid and V is volumeo ffluid displaced, g is acceleration due to gravity
archimedes’ principle
- the upward buoyant force is equal in magnitude to the weight of the displaced fluid
- at depth along side of cube submerged in water, pressure will be the same on sides and offset each other; we know pressure is a functon of depth so pressure is greater on bottom of submerged cube, deeper into water, than presure on top of the cube
- FN = Force acting upwards on this obejct, the pressure at bottom of object X surface area at the bottom of object (surface are for cube is d2) moinus pressure top times surface area on top
- so FN= P(bottom)d2 - P(top)d2
- Force = volume of water displased X density of liquid X gravity
- volume X density is mass of liquid displace, so net force is also equal to mass of liquid displaced X gravity**
- OR can say net force acting on this object= weight of liquid displaced**
fraction of object submereged equation
V fluid/ V object
becuase pobject/ p fluid = m/v of object / m/v of fluid since m is same ends up with V fluid/ V object