EBM Tables and Equations Flashcards
Prevalence =
(total #sick)/(total population)
- how many people are sick
- a single time point
- Prevalence can be used to estimate the likelihood of a diagnosis before any “tests”
Cumulative incidence:
(new cases)/(total population at risk at study start)
- how many people are getting sick (NEW CASES)
- incidence is a rate, it happens over a time interval
Incidence equation in steady state:
prevalence / duration
Duration equation in steady state:
prevalence / incidence
Prevalence equation in steady state:
(incidence) X (average duration)
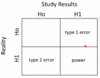
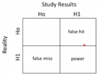
alpha =
- the probability you’ll make a false hit.
- Arbitrarily, p = 0.05
- false hit = type 1 error
- 5% of the time, we’ll make an error
beta =
- probability you won’t find a difference when one actually exists (false miss)
- false miss = type 2 error
- probability of missing a reality
power =
1 - beta
- power of study to pick up a study when it actually does exist
- Low power is a common reason for type II errors.
2 X 2 tables for hypothesis testing:
(alpha, beta, and power)
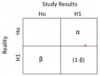
2 X 2 tables for hypothesis testing:
(type 1 and 2 errors)
2 X 2 tables for hypothesis testing:
(false hits and false misses)
95% CI equation:
95% CI = mean +/- 1.96(SEM)
- SEM = SD/ √n
Standard error of the mean (SEM) eqaution:
SEM = SD/ √n
- SD = standard deviation
- n = sample size
The three types of prevention:
- Primary: before exposure (PREVENT)
- Secondary: after exposure (SCREEN)
- Tertiary: after disease process occurs (TREAT)
Standard deviation:
+/- 3SD contains –% of observations
99.7%
Standard deviation:
+/- 1SD contains –% of observations
68%
Standard deviation:
+/- 2SD contains –% of observations
95%
The three types of variation:
-
Overall variation:
- measurement variation + biological variation
-
Measurement variation:
- instrument variation + observer variation
-
Biological variation:
- intra-individual and inter-individual
The dependent variable is on what axis?
Y-axis
The independent variable is on what axis?
X-axis
Regression r-value close to 0:
no association
Regression r-value close to 1:
strong association
Regression r-squared value tells you:
- the amount of variation in Y that is contributed by variation in X.
Three ways you can control for outliers:
- using non-parametric test
- dropping the outlier(s)
- log transformation
Variance =
measure of the spread/dispersion of values around the mean.
Standard deviation =
√v; (v = variance)
- decreases as sample size increases
Standard error of the mean (SEM) =
SD/ √n
Equation for 95% CI:
95% CI = mean +/- 1.96(SD/ √n)
- SD = standard deviation
- n = sample size
For correlation analyses, the confidence interval cannot contain:
0
0 = no correlation
For relative risk, hazard ratios, and odds ratios, the confidence interval cannot contain:
1
Sensitivity equation and table:
TP/(TP+FN)
SNOUT
Specificity equation and table:
TN/(TN+FP)
SPIN
Positive predictive value equation and table:
TP/(TP+FP)
Negative predictive value equation and table:
TN(TN+FN)
False positive rate =
1 - specificity
Positive Likelihood Ratio equation:
LR+ = Sn / (1-Sp)
Negative Likelihood Ratio equation:
LR- = (1-Sn) / Sp
Odds ratio definition and table:
- retrospective
- start with people who have disease, and go backwards to find risk factor
The probability of two mutually exclusive events, A or B, occurring =
(probability A) + (probability B)
Relative risk definition and table:
- prospective; follow
- start with the risk factor, follow them over time, see how many people get disease
- everyone starts with no disease
Probability:
- a proportion in which the frequency of both events are in the denominator:
A/(A+B)
The probability of two independent events, A and B, occurring together =
(probability A) X (probability B)
Absolute risk definition and table:
- attributable risk
- a difference of relative risks
- how much of getting the disease is attributable to having the risk factor
Number needed to treat (NNT) equation and table:
- takes everyone into account
- NNT = 1/ARR
Absolute risk reduction equation and table:
Number Needed to Harm (NNH) equation:
1/AR
