chapter 5 Flashcards
1
Q
what is a fluid?
A
- defined as a substance that can change its shape to correspond to that of the container (gases and liquids)
2
Q
what is density?
A
- denoted using rho (p) is defined as mass divided by volume
- p = m/v
3
Q
given the denisty of a material and its volume, you can easily calculate?
A
- how much mass it contains by multiplying density times volume
- pV = m
4
Q
if you need to find out how many moles you have of something given concentration and volume, you can just?
A
- multiply molarity times volume
5
Q
what are common units of density which are equivalent to eachother?
A
- g/mL
- g/cm3
- the actual SI unit for density is kg/m3
- 1000 kg/m3 = 1kg/L = 1g/mL = 1g/cm3 for the density of water at 4°C and 1 atm
- the actual SI unit for density is kg/m3
6
Q
what is specific gravity?
A
- how dense something is compared to water
- specific gravity = rho/ rhowater
7
Q
how is pressure defined?
A
- force divided by area
- P = F/A
- N/m2 and 1 N/m2 = 1 Pa
- P = F/A
8
Q
what is atmospheric pressure?
A
- refers to the baseline pressure exerted on us by all of the air present between our location and the point where the Earth’s atmosphere transitions to space
- varies with elevation, becoming lower as you go higher
- 1 atm = 101 kPa, 760 mmHg, 1 Torr, 14.7 psi
9
Q
the pressure ecerted on an object at any point submerged in a fluid is equal to the density of the fluid multiplied by g, multiplied by the depth of submersion:
A
- Psub = rho g h
- h indicates the depth of submersion (height of the fluid above the object)
10
Q
if we are specifically interested in the pressure exerted by a liquid on an object submerged in it, we use the term?
A
- hydrostatic pressure, which is defined as Psub = rho gh
11
Q
if we are interested in the total pressure exerted on such an object by both the hydrostatic pressure of the water and the atmosphere, we call this the?
A
- absolute pressure
- Psub = Patm + rho g h
12
Q
what is guage pressure?
A
- situtations where we are clear that a given pressure reading does not reflect atmospheric pressure
- Pguage = Psystem - Patm
13
Q
what is buoyancy?
A
- some objects float on the surface of water and others sink
- whether objects float or sink is a property of its density
- a low density object will will float on top of water
- higher density obkects sink
- the degree to which an object is submerged in water is proportional to its specific gravity
- whether objects float or sink is a property of its density
14
Q
if the specific gravity of an object is greater than 1, it is denser than water so it will?
if the specific gravity is equal to 1, it will be?
if the specific gravity is less than 1 it will?
A
- sink
- be completely submerged but at equilibrium
- will float, but will be partially submerged in the water
- in fact, the percentage it will be submerged is equal to its specific gravity, expressed as a percentage
15
Q
What if we’re not immersing the subject in water, or what if we’re using water with a non-standard density. In that case, we can use the following equation:
A
- % submerged = rhoobject/rholiquid x 100
- when an object is floating, the fluid it is floating in exerts an upward force on it. If the magnitude of this upward force, which is known as the buoyant force, exceeds that of the force of gravity that is pulling the object downward, then the object floats. If it does not, it sinks
16
Q
what is Archimede’s principle?
A
- weight = rho V g
- the buoyant force is therefore directly proportional to the volume of displaced fluid as modified by the density of the water
17
Q
what is Pascal’s law?
A
- states that a change in pressure made at any location within an enclosed fluid will be transmitted equally to all points throughout that fluid
- principle underlying hydraulic lift which ises this principle to generate mechanical advantage
- DeltaP = F1/A1 = F2/A2
- F2 = A2/A1F1
- DeltaP = F1/A1 = F2/A2
- principle underlying hydraulic lift which ises this principle to generate mechanical advantage
18
Q
Work is therefore conserved:
A
- W = F1d1 = F2d2
19
Q
what is surface tension?
A
- liquid is held together by the presence of intermolecular forces among molecules of the liquid
- each such molecule experiences attractive forces from all of the other water molecules around it, and we can assume they balance out. However, the intermolecular forces from other water molecules act unevenly on water molecules that are on the surface of the water. these molecules experience attractive forces from the water molecules deeper in the liquid, but virtually no compensating interactions with the air. this imbalance of forces acting on water molecules on the surface creates tension
20
Q
surface tension has units of?
A
- force divided by lnegth
- or energy divided by area so more surface tension means more energy, so ssytems will want to minimize surface tension and the best way to do this is to minimize surfcace area
21
Q
the effect of intermolecular forces within a given substance is known as?
A
- cohesion
22
Q
- It is possible for a liquid to interact with a substance that it is in contact with. such forces are known as?
A
- adhesion forces
- can be used to analyze how objects do or do not stick to each other
23
Q
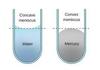
If the adhesive forces are stronger than the forces of surface tension, the liquid will crawl up the walls of the container to a slight extent. This results in the surface of the liquid becoming curved, which is knonw as a?
A
- meniscus
24
Q
the meniscus formed by water is a?
A
- concave meniscus
25
If the fluid interacts more strongly with itself than with the walls of the container, that is if the cohesive forces involed in surface tension outweigh the adhesive forves with the container, the fluid concentrates at the center and is known as?
* a concave meniscus
26
what is capillary action?
* the interplay of adhesion forces and surface tension can allow liquids to ride up the tube in opposition to gravity
27
what is flow?
* liquids can flow, but some do so more easily than others
28
The property of being rsistant to flow, or more technically, resistance to deformayion by shear stress is known as?
* viscosity
29
What is laminar flow?
used to describe scenarios in which a flowing fluid is composed of parallel layers that may be moving at different velocities
* corresponds to smooth, well-behanved flow that is easy to model
30
what is turbulent flow?
* the smoothly regulated layers of laminar flow break down. Pressure and flow can vary dramatically and unpredictably in turbulent flow
31
the onset of turbulence can be predicted to some extent using a value known as?
* Reynolds numer
* a dimensionless constant
* the higher the velocity, the more likely flow is to become turbulent
32
What is Poiseuille's law?
* used to describe Laminar flow of incompressible fluids through a long cylindrical tube
* accounts for viscosity
* Q = Πr4deltaP/8(viscosity)L
* Q is the flow rate
* deltaP is the change in pressure from one end of the tube to the other
* viscosity is the viscosity (n)
* L is the length of the tube
* r is the radius
33
relationships in Poiseuille's law:
* the flow rate (Q) and the pressure drop (deltaP) are proportional ti each other. A large pressure drop will cause flow rate to increase
* Length (L) is proportional to pressure drop (deltaP) and inversely proportional to flow rate
* Flow rate is proportional to the radius to the fourth power. this means that if we hold all other quantities equal, decreasing the radius will dramatically increase the amount of pressure necessary to push fluid through the pipe
34
What is Bernoulli's law?
* holds only for idealized fluids, for which we assume laminar flow, neglect viscosity, and neglect any interactions that may take place between the fluid and its container
* P1 + 1/2rhov12 + rhogh1 = P2 + 1/2rhov22 + rhogh2
* 1/2rhov12 correspond to kinetic energy
* rhogh1 corresponds to potential energy
* just conservation of energy applied in the contect of energy density of fluids
35
Bernoulli's equation tells us that a fluid contains 3 forms:
1. the pressure it exerts against the walls of its container
2. the kinetic energy that is proportional to its velocity squared
3. gravitational potential energy
* these 3 forms of energy can be interconverted
36
What is the continuity equation?
* states that within a closed system, the flow rate of a liquid is constant
* implies a relationship between the velocity of the fluid and the cross-sectional area that it is flowing through:
* v1A1 = v2A2
* increase cross-sectional area through which a liquid is flowing, we decrease its velocity, vice versa
37
linking together the Bernoulli equation and the continuity equation, there are some important relationships that we can observe for ideal liquids (if we neglect viscosity, turbulence, and interactions with the wall of the container)
* narrower tube → higher velocity
* narrower tube → lower pressure
* higher velocity → lower pressure
38
the fact that a narrower tube is associated with a lower pressure is known as the?
* Venturi effect
* means that measuring the pressure drop across 2 areas of pipe can be used to measure the velocity of fluid flow
39
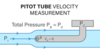
What is a Pitot tube?
* used to meausre fluid velocity in several important contexts
* rely on the same principle of conservation of energy that is expressed in Bernoulli's equation
* A Pitot tube is just a one-ended tube that is pointed into the flow of the fluid that one wants to measure so the fluid that enters cannot exit
* as fluids get stuck, it exertts a pressure on the back of the tube which is referred as the stagnation pressure
* A Pitot tube can also measure static pressure which is essentially the pressure along the sides of the tube
* the difference between the static pressure and the stagnation pressure reflects the contribution of the flowing fluid, and is known as the dynamic pressure (1/2rhov2)
* Pstag - Pstatic = 1/2rhov2
