Rates, Kinetics, Equilibrium

Question 1

For the generic chemical reaction:

aA + bB ⇒ cC + dD

where a, b, c, and d represent the coefficient of reactants A, B, C, and D respectively, what is the rate at which the reaction will proceed?

Answer 1

For the generic chemical reaction

aA + bB ⇒ cC + dD

the rate expression is:

rate = k_obs [A]^x [B]^y

where k_obs is the rate constant, and x and y are the reaction orders of A and B, respectively.

Question 2

How is the overall order of a chemical reaction calculated?

Answer 2

The overall order of a chemical reaction is the sum of the reaction orders of all the reactants.

Question 3

Define:

The rate constant of a chemical reaction

Answer 3

A chemical reaction’s rate constant, k_obs, is the rate at which the reaction proceeds when the concentration of all reactants is 1M or 1 atm.

Question 4

Define:

A zeroth order reaction

Answer 4

A zeroth order reaction is one whose overall reaction order is zero, and is independent of reactant concentration.

A zeroth order reaction’s rate is constant, and can be given by:

Rate = k_obs

Question 5

If a chemical reaction is zeroth order in [A], with A being one of the reactants, how does the reaction rate vary if [A] doubles?

Answer 5

The reaction rate is unchanged.

If the reaction is zeroth order in [A], that means that the rate can be described as:

rate = k_obs[A]^{<span>0</span>} = k_obs

Changing the value of [A] doesn’t change the reaction rate.

Question 6

Define:

A first order reaction

Answer 6

A first order reaction is one whose overall reaction order is 1, and whose rate depends on the concentration of only one reactant, in a linear fashion.

If A is the reactant on which the reaction rate depends, the rate will be:

Rate = k_obs[A]¹

Question 7

If a chemical reaction is first order in [A], how does the reaction rate vary if [A] doubles?

Answer 7

The reaction rate doubles.

First order in [A] means the rate law is:

rate = k_obs[A]¹

Let [A]_orig = x.
Then rate_orig = k_obs * x.
If [A] doubles, then

rate_new = k_obs (2x) = 2 * rate_orig

Question 8

Define:

A second order reaction

Answer 8

A second order reaction is one whose overall reaction order is 2, and whose rate either depends on one reactant to the second order, or two separate reactants to the first order.

If the reactants for the reaction are A and B, the rate will be one of:

Rate = k_obs[A]²
or Rate = k_obs [B]²
or Rate = k_obs [A]¹[B]¹

Question 9

If a chemical reaction is second order in [A], how does the reaction rate vary if [A] triples?

Answer 9

The reaction rate increases by a factor of 9.

Second order in [A] means the rate law is:

rate = k_obs[A]²

Let [A]_orig = x
Then rate_orig = k_obs * x².
If [A] triples, then

rate_new = k_obs (3x)² = 9 * rate_orig

Question 10

If a chemical reaction is first order in both [A] and [B], how does the reaction rate vary if [A] triples, while [B] is reduced by half?

Answer 10

The reaction rate increases by a factor of 1.5.

If the reaction is first order in [A] and [B] means the rate law is:

rate = k_obs[A]¹ [B]¹

Let [A]_orig = x and [B]_orig = y
then rate_orig = k_obs * x * y.
If [A] triples and [B] decreases by half, then

rate_new = k_obs * (3x) * (½y)
= 1.5 rate_orig

Question 11

For a reaction with chemical A as reactant, and kinetic data given below, what is the reaction’s overall rate law?

Answer 11

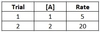
Rate = 5 [A]²

To determine the reaction order for A, see how varying [A] affects the rate. Between Trials 1 and 2, [A] increases by a factor of 2, and the rate increases by a factor of 4.

Since the rate increases by a factor of the concentration increase to the second power, the reaction must be second order in [A].

Once the reaction orders are solved, plug in the value of [A] for either trial to solve for k_obs.

Question 12

For a reaction with chemicals A and B as reactants, and kinetic data given below, what is the reaction’s overall rate law?

Answer 12

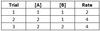
Rate = 2 [A]¹ [B]⁰

Between Trials 1 and 2, [A] doubles, while [B] stays constant, and the reaction rate doubles, so the reaction order for A is 1.

Between Trials 2 and 3, [B] doubles, while [A] stays constant, and the reaction rate is unchanged, so the reaction order for B is 0.

Plugging in the values [A] = 1 and [B] = 1 from Trial 1 delivers a final value of 2 for k_obs.

Question 13

For a reaction with chemicals A and B as reactants, and kinetic data given below, what is the reaction’s overall rate law?

Answer 13

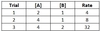
Rate = 2 [A]¹ [B]²

Between Trials 1 and 2, [A] doubles, while [B] stays constant, and the reaction rate doubles, so the reaction order for A is 1.

Between Trials 2 and 3, [B] doubles, while [A] stays constant, and the reaction rate increases by a factor of 4, so the reaction order for B is 2.

Plugging in the values [A] = 2 and [B] = 1 from Trial 1 delivers a final value of 2 for k_obs.

Question 14

What is a reaction’s rate-determining step?

Answer 14

A chemical reaction’s rate-determining step is the slowest sub-step of a reaction. As such, it limits how fast the overall reaction can proceed.

Ex: the reaction

NO₂ + CO ⇒ NO + CO₂

is actually two sub-reactions,

1) NO₂ + NO₂ ⇒ NO₃ + NO (slow)
2) NO₃ + CO ⇒ NO₂ + CO₂ (fast)

The slow first step, is the rate-determining step, and limits the overall reaction rate.

Question 15

How is a reaction’s rate-determining step identified?

Answer 15

The rate-determining step can be identified because the substances which appear in the rate law must be reactants in the rate-determining step.

Ex: If the overall reaction rate depends only on [NO₂] for the following steps:

1) NO₂ + NO₂ ⇒ NO₃ + NO
2) NO₃ + CO ⇒ NO₂ + CO₂

Then the first step, in which NO₂ appears as a reactant, must be the rate-determining step.

Question 16

Define:

An intermediate in a chemical reaction

Answer 16

A reaction intermediate is a species that plays a role in a reaction, but does not appear in the overall chemical equation.

An intermediate can be identified by the fact that it is a product of an early reaction step, and a reactant in a later one.

Question 17

Identify the intermediate in the reaction below:

Overall Reaction: 2 O₃ ⇒ 3 O₂
Step 1: O₃⇒O₂+ O
Step 2: O + O₃⇒ 2 O₂

Answer 17

O is the intermediate.

O is a product of Step 1, and a reactant of Step 2, and does not appear in the overall reaction. Therefore, it is an intermediate.

Question 18

What is the molecularity of an elemental chemical reaction?

Answer 18

Molecularity is the number of reactant molecules taking part in a single reaction step. Therefore, it is a concept that can only be applied to elementary reactions.

A reaction involving one molecule of reactant is unimolecular; two is bimolecular; and three is termolecular.

Question 19

What is the molecularity of the elementary reaction below?

NO + NO₃→ 2NO₂

Answer 19

NO + NO₃→ 2NO₂

The reaction has two individual molecules as reactants. Therefore, it is bimolecular.

Question 20

In the chemical reaction Energy Profile shown below, where are the reactants and the products located?

Answer 20

In Energy Profiles, the reactants are on the left, at the beginning of the reaction.

The products are on the right, at the end of the reaction.

Question 21

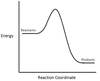
In the chemical reaction Energy Profile below, what is the quantity A?

Answer 21

A is the Activation Energy E_a.

The Activation Energy determines how much energy the reactants require to convert to the activated complex, or transition state. The higher the Activation Energy, the slower the reaction proceeds.

Question 22

In the chemical reaction Energy Profile below, what portion of the reaction is located at point C?

Answer 22

The reaction’s Transition State is located at the peak of the reaction profile.

The Transition State, also known as the Activated Complex, is the chemical intermediate halfway between the reactants and the products. Since it is the highest energy point in the reaction profile, it is the least stable part of the reaction, and the reaction rapidly proceeds to the products once the transition state is reached.

Question 23

In the chemical reaction Energy Profile below, what is the quantity B?

Answer 23

B is the Reaction Enthalpy, ΔH.

The Reaction Enthalpy is a measure of the difference in energy level between the reactants and the products.

If ΔH < 0, the reaction is exothermic.
If ΔH > 0, the reaction is endothermic.

Question 24

Is the chemical reaction being depicted below endothermic or exothermic?

Answer 24

It is exothermic.

The products’ enthalpy is lower than the reactants’, so ΔH < 0, meaning the reaction is exothermic.

Question 25

Is the chemical reaction being depicted below endothermic or exothermic?

Answer 25

It is endothermic.

The products’ enthalpy is higher than the reactants’, so ΔH > 0, meaning the reaction is endothermic.

Question 26

What is the Arrhenius equation?

Answer 26

The Arrhenius equation relates a reaction’s Activation Energy E_a, Rate Constant k_obs, the temperature of the chemical system T, and the ideal gas constant R.

Question 27

According to the Arrhenius equation, how does a reaction’s rate vary as temperature is increased?

Answer 27

As the temperature increases, the reaction’s rate increases as well.

As T increases, the term e^-E_a/RT, which starts out as a small fraction, moves closer and closer to 1, raising the calculated value of k_obs and speeding up the reaction.

In general, the Arrhenius equation predicts that a reaction’s rate will double for every 10ºK increase in temperature.

Question 28

Of the two reactions shown below, which is kinetically preferred?

Answer 28

Reaction B is kinetically preferred.

A reaction’s activation energy determines whether it is kinetically preferred or not. In this case, E_a is smaller for reaction B than it is for reaction A. So reaction B runs faster, and is kinetically preferred.

Question 29

If reactions A and B are run simultaneously at low temperature, which set of products will result?

Answer 29

At low temperature, reaction B’s products will dominate.

At low temperatures, the kinetically preferred reaction will be favored. Since B is kinetically preferred, it will dominate under these conditions.

Question 30

Of the two reactions shown below, which is thermodynamically preferred?

Answer 30

Reaction A is thermodynamically preferred.

A reaction’s enthalpy change determines whether it is thermodynamically preferred or not. In this case, ΔH_A is larger in magnitude than ΔH_B, so the products of A are more thermodynamically stable, and reaction A is thermodynamically preferred.

Question 31

If reactions A and B are run simultaneously at high temperature, which set of products will result?

Answer 31

At high temperature, reaction A’s products will dominate.

At high temperatures, the thermodynamically preferred reaction will be favored. Since A is thermodynamically preferred, it will dominate under these conditions.

Question 32

Define:

A chemical catalyst

Answer 32

A catalyst is a chemical which participates in a reaction and serves to increase the reaction’s rate. Catalysts are not themselves depleted by the reaction.

Question 33

How does a chemical catalyst change the energy profile of a chemical reaction?

Answer 33

Chemical catalysts decrease a reaction’s Activation Energy.

Ex: The below energy profile demonstrates positive catalysis. Note that E_a decreases, but ΔH is unaffected by the catalyst.

Question 34

What role do enzymes play in chemical reactions?

Answer 34

Enzymes serve as catalysts in chemical reactions.

Therefore, they speed up or slow down reactions, but they are not affected by the reaction, and they cannot change the thermodynamic favorability of a reaction.

Question 35

What is the difference between a homogeneous catalyst and heterogeneous catalyst?

Answer 35

• A homogeneous catalyst is in the same phase as the remaining reactants.
• A heterogeneous catalyst is in a different phase from the remaining reactants.

Question 36

Define:

Autocatalysis

Answer 36

A reaction undergoes autocatalysis if the product of the reaction further catalyzes the reaction.

Question 37

Define:

The Law of Mass Action

Answer 37

The law of mass action states that, at equilibrium, the composition of the reaction mixture can be expressed in terms of an ideal equilibrium constant, k_eq.

For the chemical reaction

aA (g) + bB (g) ⇔ cC (g)

if [C]_eq is the concentration of C at equilibrium,

Question 38

For the chemical reaction

aA (g) + bB (g) ⇔ cC (g)

what is the value of the reaction quotient Q?

Answer 38

The expression for Q is very similar to the expression for k_eq. The only difference is that Q can apply to a chemical system at any concentrations, while k_eq specifically refers to the concentrations at equilibrium.

Question 39

For the chemical reaction

A (g) ⇔ B (g)

what does it mean about the equilibrium concentrations of A and B if:

1. k_eq >> 1
2. k_eq = 1
3. k_eq >> 1

Answer 39

For this system,

k_eq = [B]_eq/[A]_eq

1. If k_eq >> 1, [B]_eq >> [A]_eq.
2. If k_eq = 1, [B]_eq = [A]_eq.
3. If k_eq << 1, [A]_eq >> [B]_eq.

Question 40

What is the equilibrium constant for the reaction:

NO + NO₃ ⇔ 2NO₂

Answer 40

The equilibrium constant is calculated from the concentrations of the products and reactants at equilibrium:

Question 41

What is the equilibrium constant k_eq for the following reaction:

CaCO₃(s)→CaO(s)+CO₂(g)

Answer 41

k_eq=[CO₂]

The value of the equilibrium constant (and reaction quotient) depends only on the concentration of reactants and products present in the aqueous or gaseous phases. Chemicals in the solid or liquid phase do not affect the equilibrium levels.

Question 42

What is true of the relationship between K_eq and Q for any chemical system which is at equilibrium?

Answer 42

Equilibrium occurs when the reactants and products are at concentrations such that the reaction quotient, Q, is equal to the equilibrium constant k_eq.

Question 43

What can be said about the rates of the forward and reverse reactions for any chemical system which is at equilibrium?

Answer 43

When the system is at equilibrium, by definition, the rates of the forward and reverse reactions are equal.

Question 44

If the chemical system

NO + NO₃ ⇔ 2NO₂

is at dynamic equilibrium, what can be said about the rates of the forward and reverse reactions?

Answer 44

The rates of the forward and reverse reactions are equal.

For every molecule of NO that comes together with a molecule of NO₃ to make 2 molecules of NO₂, 2 molecules of NO₂ will also come together to make a molecule of NO and NO₃.

Question 45

Define:

Le Chatelier’s Principle

Answer 45

Le Chatelier’s Principle states that when a system in equilibrium is placed under stress, the system adjusts to restore equilibrium.

There are three kinds of stress a chemical system can be placed under:

• Concentration
• Temperature
• Pressure

Question 46

If the chemical system

aA (g) + bB (g) ⇔ cC (g)

is in equilibrium, and additional A is added, what does Le Chatelier’s Principle predict will occur?

Answer 46

More C will be created.

According to Le Chatelier’s Principle, the system will respond to relieve any stress placed on one side of the system.

In this case, the reactant side is the one placed under stress by the addition. As such, the system will respond by shifting to the right, favoring the forward reaction, and more products will be created.

Question 47

If the chemical system

aA (g) + bB (g) ⇔ cC (g)

is in equilibrium, and additional A is added, what happens to the reaction quotient Q?

Answer 47

Q decreases.

This circumstance favors the forward reaction, or the creation of more products.

As a general rule of reactions: when Q < k_eq, the forward reaction is favored and more products are created.

Question 48

If the chemical system

aA (g) + bB (g) ⇔ cC (g)

is in equilibrium, and additional C is added, what does Le Chatelier’s Principle predict will occur?

Answer 48

More A and B will be created.

In this case, the product side is the one placed under stress by the addition. As such, the system will respond by shifting to the left, favoring the reverse reaction, and more products will be created.

Question 49

If the chemical system

aA (g) + bB (g) ⇔ cC (g)

is in equilibrium, and is endothermic, what happens if the temperature is increased?

Answer 49

More C will be created.

For an endothermic reaction, heat must be added; hence it can be thought of as a reactant. Increasing the temperature is akin to adding more reactant, therefore, and shifts the reaction to the right.

Question 50

If the chemical system

aA (g) + bB (g) ⇔ cC (g)

is in equilibrium, and is exothermic, what happens if the temperature is increased?

Answer 50

More A and B will be created.

For an exothermic reaction, heat is given off; hence it can be thought of as a product. Increasing the temperature is akin to adding more product, therefore, and shifts the reaction to the left.

Question 51

If the chemical system

A (g) + 2 B (g) ⇔ C (g)

is in equilibrium, what happens if the pressure is increased?

Answer 51

More C will be created.

Increasing the pressure will add stress to the side which has more moles of gas. In this case, that is the reactant side, with 3 moles of gas vs. 1 mole of gas on the product side.

So, when the pressure is increased, the system will shift right, creating more products.

Question 52

If the chemical system

A (g) + B (g) ⇔ 3 C (g)

is in equilibrium, what happens if the pressure is decreased?

Answer 52

More C will be created.

Decreasing the pressure lessens the inhibition for the side creating more moles of gas. In this case, the product side has 3 moles of gas vs. 2 total moles of gas on the reactant side.

So, when the pressure is decreased, the system will shift right, creating more products.

Question 53

What is the relationship between a substance’s Gibbs’ Free Energy of Formation and the equilibrium constant of the substance’s formation reaction?

Answer 53

ΔGº = -RT ln(k_eq)