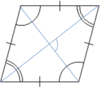
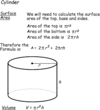
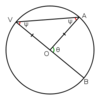
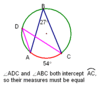
Math Review Flashcards
If an integer b is not a divisor of a, then the result can be viewed in 3 diff ways…
If an integer b is not a divisor of a, then the result can be viewed in 3 diff ways…
1) A fraction
2) A decimal
3) A quotient with remainder
The product of two positive integers is…
The product of two positive integers is a positive integer
The product of two negative integers is…
The product of two positive integers is a positive integer
The product of a positive integer and negative integer is…
The product of a positive integer and negative integer is a negative integer
Each of the multiplied integers that result in a product
Factor/Divisor
When an integer *a * is divided by an integer b, where *b * is a divisor of *a, *the result is always
When an integer a is divided by an integer b, where b is a divisor of a, the result is always
A divisor of a
EX: When 60 is divided by 6(one of its divisors), the result is 10, which is another divisor of 60
When a positive integer a is divided by a positive integer *b, you first find the first greatest multiple of b that is less than or equal to a. That multiple of b can be expressed as the product qb(where q is quotient). Then the remainder is equal a *minus that multiple of b, or r = a - qb, where *r *is the remainder.
The remainder is always greater than or equal 0 and less than b.
When a positive odd integer is divided by 2…
When a positive odd integer is divided by 2…
The remainder is always 1
To divide one fraction by another…
To divide one fraction by another…
First invert the second fraction(i.e. find it’s reciprocal), then multiply the first fraction by the inverted fraction.
17/8 ÷3/4 = (17/8)(4/3) = (4/8)(17/3) = (1/2)(17/3) = 17/6
Numbers of the form a/b, where either *a *or b is not an integer and b NE 0, are fractional expressions that can be manipulated just like fractions.
EX: Numbers π/2 and π/3 can be added as:
π/2 + π/3 = (π/2)(3/3) + (π/3)(2/2) = (3π/6)(2π/6) = 5π/6
Exponents are..
Exponents are..
Used to denote the repeated multiplication of a number by itself.
-Remember the base **and exponent
A negative number raised to an odd power…
A negative number raised to an odd power..
is always negative
NOTE: The exponent is applied before the negative sign when you have an expression with exponents
Without parentheses the expression -32 means “the negative of ‘3 squared.’” So (-3)2= 9,
but -32 = -9
All positive numbers have two square roots, one positive and one negative
The symbol √n is used to denote the nonnegative square root of the nonnegative number n.
Therefore, √100 = 10 and –√100 = –10
(– √a)2 =
(– √a)2 = a
(– √3)2= 3
√a2=
√a2 = a
√π2 = π
√a√b =
√a√b = √ab
√3√10 = √30
√a/√b =
√a/√b = √a/b
√18/√2 = √18/2 = √9 = 3
For odd-order roots
For odd-order roots, there is exactly one **root for every number n, even when n is negative.
EX: 8 has exactly one cube root, 3√8 = 2
and –8 has exactly one cube root, 3√–8 = –2
For even-order roots
For even-order roots, there are exactly two roots for every positive number n and **no roots **for any **negative **number n.
EX: 8 has two fourth roots, 4√8 and –4√8,
but -8 has no fourth root, since it is negative
All rational numbers and irrational numbers, including all integers, fractions and decimals.
Real number
If a + b = b + a, then
If a + b = b + a, then ab = ba
EX: 8 + 2 = 2 + 8 = 10
and (-3)(17) = (17)(-3) = -51
If (a + *b) + c = a + (b* + c), then
If (a + b) + c = a + (b + c), then (ab)c = *a(bc*)
EX: (7 + 3) + 8 = 7 + (3 + 8) = 18,
and (7√2) √2 = 7(√2√2) = (7)(2) = 14
If a(b +c) =
If a(b + c) = ab + ac
EX: 5(3 + 16) = (5)(3) + (5)(16) = 15 + 80 = 95
a + 0 = *a, (a)(0) = 0, and (a*)(1) = a
If ab = 0, then
If ab = 0, then a = 0 or b = 0 or both
EX: -2b = 0, then b = 0
|a + b| <= la| + lb|
The triangle inequality
EX: If a = 5 and *b *= –2,
then |5 + (–2)| = |5 - 2| = |3| = 3
and |5| + |–2| = 5 + 2 = 7
Therefore, |5 + (–2)| <= |5| + |–2|
If* a *> 1, then a2 > a. If 0 < b < 1, then b2 < b
EX: 52 = 25 > 5, but (1/5)2 = 1/25 < 1/5
Ratio is
Ratio is a way to express relative size, often in the form of a fraction.
Given the *percent *and *whole, *compute the percent
Given the percent and whole, compute the percent by dividing the *part * by the whole. Result will be decimal equivalent, so multiply result by 100
To find the part that is a certain * percent* of the whole,
To find the part that is a certain percent of the whole, you can:
1) either multiply the percent by the decimal equivalent of the percent.
EX: Find 30% of 350 by x = (350)(.3) = 105
2) or set up a proportion to find the part.
EX: Find the numer of parts of 350 that yields the same ratio as 30 out of 100 parts. x/350 = 30/100 and solve for x
Given the *percent *and the part, calculate the whole
Given the percent and the part, calculate the whole.
1) Either use the decimal equivalent* *of the percent
EX: 15 is 60% of what number?
- 6z = 15 and slove for z
2) or set up a proportion and solve
* part/whole* = 60/100 –> 15/*z = 60/100 *and cross multiply
The whole is _____ of the percent
base
The amount of change as a percent of the initial amount
percent change
When computing a percent increase the base..
When computing a percent increase the base is the smaller number.
NOTE: Base is initial number, before the change
When computing a percent decrease, the base is…
When computing a percent decrease, the base is the larger number.
NOTE: Base is initial number, before the change
Roots and exponents
**Roots ** are closely related to exponents. Exponents and roots can also undo each other
√52= 5 and (3√73) = 7
What to do?
2/x-1
Always get variables out of denominators.
Whenever you see an equation with a squared variable, you need to:
- Recognize that the equation may have two solutions
- Know how to find both solutions
x2 = 4
√x2 = √4
x = 2 or -2
Before factoring a quadratic expression…
you MUST make sure that the other side of the equation equals 0
x2 - y2 =
(x + y) (x - y)
x2 + 2xy + y2 =
(x + y) (x + y) = (x + y)2
x2 - 2xy +y2 =
(x - y) (x - y) = (x - y)2
Variables in absolute value
|y| = 3
When there is a variable inside an absolute value, you should look for the variable to have two possible values.
+(y) = 3 or -(y) = 3
y = 3 or -3
____________________
|2x+4| = - (2x+4) OR (2x+4)
When performing operations on compound inequalities
Perform operations on every term in the inequality:
x + 3 < y < x + 5
x < y - 3 < x + 2
______________________
c/2 < b - 3 < d/2
c < 2b - 6 < d
When dealing with optimization problems:
Problems involving optimization are related to extreme values: specifically, minimizing or maximizing. Focus on the largest and smallest possible values for each of the variables.
1/100
0.01 or 1%
1/50
0.02 or 2%
1/25
0.04 or 4%
1/20
0.05 or 5%
1/10
0.10 or 10%
1/9
0.11 ≈ 0.111 ≈ 11.1%
1/8
0.125 or 12.5%
1/6
0.16 ≈ 0.167 ≈ 16.7%
1/5
0.2 or 20%
Pythagorean Theorem
For any right triangle, the relationship that a2 + b2 = c2
Where a and b are the legs of a triangle and c is the hypotenuse.
Ignore the numbers: Rectangle is inscribed in a circle. If the circumference of the circle is 5π and the diameter is 4, what is the area of the rectangle?
Remember: Any line that passes through the center is a diameter, choose the best angle to solve.
Where b = 4 and d = diameter (hypotenuse)
C = 2πr ; C = 5π, so 5π = 2πr now find r.
5 = 2r; 2 = 5/2 = 2.5
d = 2r = 2(2.5) = 5
a2 + b2 = d2 -> a2 + 42 = 52
a2 + 16 = 25
a2 = 9 -> a = 3
a = 3 and b = 4
A(rectangle) = length*width = (4)(3) = 12
The sum of any two side lengths of a triangle will always be __________ than the third side length.
The sum of any two side lengths of a triangle will always be greater than the third side length. This is because the shortest distance between two points is a straight line.
The third side length will always be ________ than the difference of the other two side lengths.
The third side length will always be greater than the difference of the other two side lengths
isosceles triangle
A triangle that has two equal angles and two equal sides (opposite the equal angles)
equilateral triangle
A triangle that has three equal sides (all 60) and three equal sides
Sides of a triangle correspond to their opposite angles
The longest side is opposite the largest angle, and the smallest side is opposite the smallest angle.
Area of a triangle
A(tri) = 1/2 (base)(height)
- base and height MUST be perpendicular to each other, so that it forms a 90° angle
- Any side of the triangle could act as a base, only thing that matters is that base and height are perpendicular to each other.
Pythagorean triplet
A relationship of right triangles (the hypotenuse, or largest side, corresponds to the largest number in the triplet) where all three sides have lengths that are integer values.
45-45-90 tri
Isosceles right triangle has a 90° angle (opposite the hyp) and two 45° angles (opposite two equal legs) and has a specific ratio:
45° –> 45° –> 90°
leg –> leg –> hyp
1 : 1 : √2
x : x : x√2
30-60-90 tri
An equilateral triangle in which all three sides (and all three angles) are equal. Each angle is 60°. A close relative of the equilateral triangle is the 30-60-90 triangle, when put together, form an equilateral triangle
30°–>60°–>90°
short–>long–>hyp
1 : √3 : 2
x : x√3 : 2x
diagonal of square/face of cube
- d* = s√2, where s is a side of a square
- recall, any squre can be divided into two 45-45-90 triangles and can use the ration 1 : 1 : √2
main (space) diagonal of a cube
d = s√3, where s is the edge of the cube
Trapezoid
Quadrilateral, where one pair of opposite sides is parallel.
Parallelogram
Quadrilateral, where opposite sides and opposite angles are equal.
Rhombus
Quadrilateral, where opposite sides and opposite angles are equal. All sides are equal.
Surface Area
the SUM of the areas of ALL of the faces
2(LW + LH + WH)
Volume
Length x Width x Height
Of all quadrilaterals with a given perimeter, the _______ has the largest area
Of all quadrilaterals with a given perimeter, the SQUARE has the largest area
Arc length
portion of circumference remaining in a sector
central angle/360*Circumference of full circle
(n°/360°) * 2πr
central angle - An angle whose vertex lies at the center point of a circle (an arc and sector of a circle)
Sector area
(n°/360°) * Area of whole circle
(n°/360°) * πr2
Inscribed angle
has its vertex on the circle itself (rather than on the center of the circle).
