Key notes

Question 1

• How is the ESE approximated further?

Answer 1

• ESE still too complex to solve e.g. due to 2^nd differential in KE term
• Separate into a universal (F_e) and an external potential (V_ext)

Question 3

• (IMP) Outline briefly how the ESE is solved ab-intio via wavefunction theory
• (DFT is also ab initio just different appraoch)

Answer 3

• Exact ESE is solved through approximation of the ψ through variational or perturbation theory
• Uses HF of non-interacting electrons in a mean field
• Electron correlation captured through post HF methods
• E.g. CI, MP2 only differing in how they approximate ψ

Question 4

• (IMP) Why might it be more appropriate to use electron density, ρ(r) to solve the ESE

Answer 4

• Ψ depends on 3n coordinates for n electrons
• Storing this info for >100 electrons is unfeasible
• ρ(r) depends only on 3 coordinate (x,y,z), describing the probability distribution of electrons in space at that point
• much simpler as only depends on position you measure it.

Question 5

• What is the problem with expressing the universal function F in terms of density?

Answer 5

• Expansion of the original KE term contains a 2^nd derivative of ψ which cannot be formulated as a simple function of ρ
• We know that F[ρ] must exist but can’t write it down.

Question 6

• (IMP) Describe the details of this figure

Answer 6

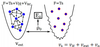
• Adiabatic connection of an artificial auxiliary system, v_s and a real many-body system, v_ext connected by the same electron density, ρ
• Real system defined by coulomb interaction of all particles (complex)
• Mapped into a non-interacting system encoded by overall potential describing indirect interaction between atoms.

Question 7

(IMP) Describe the key differences between Wave function and KS theory

Answer 7

• Wave function theory
  
  • Complexity in high dimensional ψ via exact ESE
  • Finite sum over slater determinants
  • Finite approximation of ψ àMP2,CCSD etc
  • Very slow convergence to get exact E­_corr but results in exact path via systematic improvements through variational theory
  • Very computationally expensive (limited to ~30 atoms)
• DFT
  
  • Complexity in v_XC (less as based on density alone)
  • FInite sum over KS equations describing orbital like states
  • Non-local potential in space (and time) connects all possible interactions
  • Local approximations to v_XC makes systematic path to true answer difficult
  • Good error calculations
  • Computationally very efficient

Question 8

• Every external potential can have only … density
• But the same density can connect to … … external potentials
• Therefore, we can calculate the GS density of …-… e­^-s (via Hartree theory) in an … … v_s that is known
• Use this … … potentials equivalent … to calculate the energy of the system described by …

Answer 8

• Every external potential can have only one density
• But the same density can connect to two different external potentials
• Therefore, we can calculate the GS density of non-interacting e­^-s (via Hartree theory) in an effective potential v_s that is known
• Use this made up potentials equivalent ρ to calculate the energy of the system described by v_ext

Question 9

• What is the basic assumption of Local-density approximations (LDA)?

Answer 9

• E_XC only depends on the value of the local electron density at one point

Question 10

• Discuss some other problems with LDA’s

Answer 10

• Binding energies are too negative i.e.overbinding
• Activation energies are unreliable
• Band gaps, ionization energies and electron affinities are strongly underestimated.

Question 11

• What are Generalized-gradient approximation (GGA’s) and how do they improve upon LDAs

Answer 11

• Similar form but a local gradient of electron density is included as well as the value of density
• This is to gain information on the local variation in neighbouring electron density at other positions

Question 13

• How does the bulk lattice constants and cohesive energies with GGA compare with LDA?

Answer 13

• Bulk lattice constants (unit cell vecotr between atoms): GGA increase due to more repulsive core-valence XC
• Cohesive energies (E released by binding atoms in to a solid): GGA reduction mostly due to valence effect, giving better description.

Question 14

• How do Energy barriers of GGAs compare to LDAs

Answer 14

• Free energy of molecule better described with GGAs, reducing the degree to which the barrier is underestimated
• LDAs underestimate to a large extent.

Question 15

Name an improvement GGAs make on LDAs

Answer 15

• GGA correct LDA overbinding, with less stiffness of tightly packed system

Question 16

• Where do GGAs still fall short

Answer 16

• Still no long-range description of vdW forces as local approximation (same as LDA)
• As GGA favours low coordination (large gradient), can now interpret different E sites on a surface (LDA could not distinguish). However can do so incorrectly.

Question 17

• What is the general idea of meta-GGAs?

Answer 17

• Expand the local function dependence to occupied KS orbitals.
• 2^nd derivative of KE added via T[ρ] of non-interacting electrons i.e. the product of the derivative of KS ψ of single particle space
• This leads to the function becoming explicitly orbital dependent.
• Still technically local derivative however accounts for many variations in different orbitals.

Question 18

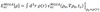
• For their simplicity, LDA/GGAs perform well for a large range of materials, marking ‘semi-…’ DFT as an important improvement in how we describe the …
• However major failures in the … of chemical reduction barriers and … … as well as the overestimation of … mark a need for further improvement.

Answer 18

• For their simplicity, LDA/GGAs perform well for a large range of materials, marking ‘semi-local’ DFT as an important improvement in how we describe the E_XC
• However major failures in the underestimation of chemical reduction barriers and band gaps as well as the overestimation of polarizabilities mark a need for further improvement.

Question 19

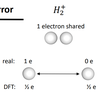
(IMP) Describe the band-gap problem in GGA’s

Answer 19

• Underestimation of difference in ionisation energy/electron affinity due to self-interaction error
• Contrary to HF, V_X and V_H + V_C don’t cancel for an H-atom
• Missing V_X causes half electrons to move apart from each other instead of contracting in to a positive and negative ion in dissociation.

Question 21

• How do meta-GGAs compare to LDA/GGA

Answer 21

• Better molecular binding E’s
• Better cohesive/structural description
• Band gaps still underestimated
• Long range vdW still not accounted for as still a local functional

Question 22

(IMP) How does the error in delocalization affect how the ionisation energy and electron affinity are related to the energies of the KS states at this stage? Use a sketch to support your answer

Answer 22

• Energies of the KS auxiliary states do not equal the electron affinity and ionisation energy of the system as they should
• This is due to the presence of half electrons in local description causing a bowing of the curve instead of clear definition
  
  • means half an electron in LUMO and HOMO instead of 1 in LUMO (I underest, A overest, E underest)
• Gradient represents energy levels and are incorrect

Question 24

(IMP) How do 4th-rung hybrid functionals solve the localization problem in meta-GGAs? Use a sketch to support your answer

Answer 24

• Includes HF exchange, which strongly over localizes/stabilizes electrons, making graph more convex.

Question 25

(IMP) What is the over localisation error also associated with the band gap problem?

Answer 25

• Electronic states are too localised as electrons do not sufficiently repel each other (Pauli repulsion missing)
• Electrons do not come close in contact unless they must (run away from themselves as orbitals more diffuse.

Question 26

(IMP) Describe the problem of missing long-range correlation in local functionals

Answer 26

• Electron correlation V_C treated incorrectly (due to local approximation)
• Leading to no long range interaction between non overlapping densities
• As a result, LDA/GGA/meta-GGA do not capture dispersion/vdW effects

Question 28

(IMP) What is the solution to the long-range correlation error?

Answer 28

Include explicit non-local correlation or long-range dispersion terms, describing sum of all pairwise interactions as a function as 1/r^6 e.g. Many body dispersion/ vdWsurf

Question 30

• How do hybrid functionals compare to local?

Answer 30

• Large improvements in barriers, band gaps and dissociation energies (≈0.1 eV expt)
• More accurate and consistent description of CO site adsorption.

Question 31

• What is the idea of 5^th rung RPA (Random Phase Approximation) functionals?

Answer 31

• Xc functionals become explicitly dependent on unoccupied orbitals as well as occupied orbitals previously
• Similar to CCSD in HF (mixing excitation to unoccupied states)