Intro to Geometry Flashcards
State the first 4 of Euclids Axioms
1) Through any two points there is a unique line 2) Its possible to draw a unique circle of any given radius around any given point 3)Its possible to extend any line segment continuously to a larger line segment 4) All right angles are equal
When are points collinear
When there is a straight line between all of them
Given 3 points ABC in the plane, how do we measure the angle

Lemma; Let a and b be a pair of intersecting lines. Then the two angles where we go counter clockwise from a to b are equal

alpha + beta = pi
alphaprine + beta = pi
therfore alpha = alphaprine
Define the angle ab of two intersecting lines a and b
L(ab) is the value of either of the two equal angles from a to b anti-clockwise
When are two lines a and b perpendicular
When L(ab) = L(ba) = pi/2
What is the perpendicular bisector of AB
The unique line which passes through the midpoint of AB and is perpendicular to AB
Give three examples of isometric maps and one which is not
Reflection, Rotation and Translation are
Dilation isnt
Define the notation for a rotation, translation and a dilation
Rotation - R(O, theta) where O is the central point
Tv: R to R ie (x,y) to (x + v1, y + v2)
D(O, alpha) dilates a map by alpha from centre O
Define an isometry
A map f:Plane to the Plane if for any two points A and B in the plane d(A,B) = d(f(A),f(B))
When is an isometry orientation preserving
L(ABC) = L(f(A)f(B)f(C))
Define Congrunecy

When are two line segments congruent
When they are of equal length, | AB | = | CD | and theres an isometry taking A to C and B to D
What is Axiom 7
Given a line l and a point P, there exists an isometry which leaves l fixed and moves P to the other side of l
When is a triangle ABC clockwise orientated
If moving from A to B to C takes you clockwise
Lemma; Isometries preserve internal angles of a triangle. More precisely, let ABC and A’B’C’ be such that ABC ~ A’B’C’ then alpha = alphaprine beta = betaprine gamma = gammaprine

Prove the SAS Congruence criterion, where | BA | = | B’A’ |, | BC | = | B’C’ | and beta=betaprine

Prove the ASA Congruence criterion, where | BC | = | B’C’ |, beta=betaprine and gamma=gammaprine

When is a triangle an isosceles
When it has two sides that are the same length
Lemma; Let ABC be a triangle in the plane. Then | AC | = | BC | if and only if alpha = beta
If alpha = beta. Then ABC is congruent to BAC by ASA so | AC | = | BC |
If | AC | = | BC | then ABC is congruent to BAC by SAS so alpha = beta
Prove the SSS congruence criterion

Lemma; For any two points A and B in the plane the perpendicular bisector of AB is the locus of points equidistant from A and B

Theorem; In any triangle ABC the perpendicular bisectors meet at a point O. The point O is the centre of the unique circle passing through A, B and C

Define a tangent
A line l is tangent to a circle C at point P if it meets C only at P
Lemma; Let l be a line and P a point not on l. Then there exists a unique line through P which is perpendicular to l, and the shortest distance from P to l is along this line

Lemma; Through any point P on a circle C there is a unique line tangent to C. It is the line perpendicular to OP where O is the centre of the circle

State the Parallel Postulate
If a straight line intersecting two straight lines makes the interior angles on one of the sides which are less than two right angles in total, then the two straight lines, if produced indefinitely, meet on that side
State Playfairs Axiom
Given a line l and a point P not on l, there is a unique line through P parallel to l
Theorem; Euclids 5th Axiom implies Playfairs Axiom

Let l1 and l2 be a pair of parallel lines. Let l3 be a line falling on l1 and l2. Then alpha = betaprine and beta = alphaprine

Lemma; The interior angles of a triangle sum to pi

Let ABCD be a parallelogram with AB||CD and AC||BD, then |AC| = |BD| and |AB| = |CD|

Give the three statement definitions of Area
1) The area of a rectangle is the product of the lengths of two adjacent sides
2) If two figures are disjoint or meet along their edges, then the area of their union is the sum of their areas
3) Congruent figures have equal areas
Lemma; The area of a parallelogram is base times height

Lemma; The area of a triangle is a half times the base times the height

When are two triangles similar
When are the angles are equal, denoted by ~
State and Prove Pythagorus’ Theorem
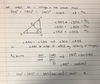
Define a great circle
A great circle, or a geodesic on a sphere is the intersection of the sphere with a plane passing through its origin
When are two points antipodal
When the straight line connecting them passes through the origin
Lemma; Through any two non-antipodal points on a sphere there passes a unique great circle

What is Ambient and Intrinsic notion?
Ambient - straight line connecting A and B
Intrinsic - Smallest arc of any great circle connecting A and B
Give 2 examples of spherical isometries
Rotation about an axis through the centre of the sphere
Any reflection in a plane passing through the centre of the sphere
Define the spherical angle BAC

State the sum of angles of a triangle ABC on a sphere of radius r
L(A) + L(B) + L(C) = pi + area(ABC)/r2


