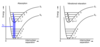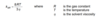Excited states and Photochemistry Flashcards
What is photochemistry and where does it take place?
Chemistry initiated by light
Takes place in the excikted state created by absorbtion of a photon with energy hv
When does an elevctronic transition take place?
- When an electron moves from one MO to another:
the electron density is redistributed
the bonding and structure chage
photochemical reactions can be quite different from thermal reactions of the same molecule
- Typically indiced by UV light
wavelengths in the range of λ = 200-800 nm
absorbers called chromophores
Equations for:
energy of one photon
energy of a mol of photons
wavenumber converting m - cm and m-1 - cm-1
Realtionship between energy, frequency, wavenumber and wavelength
Units and symbols of frequency, wavenumber, wavelength, energy (of one photon and a mol of photons), speed of light, planck’s constant, avogadro’s constant
What is the beer lambert law and what are the units of the components
Typical long-wavelength absorption properties of saturated and unsaturated systems and some typical transitions
saturated systems: usually absorb in the vacuum UV
unsaturated systems: increasing conjugation shifts the absorbtion to longer λ
σ—–>σ*
π—–>π*
n—–>π*
Transitions invloving σ* prbitals usually give higher bands (λ<200 nm)
Describe the states a molecule can be in
Ground state:
the lowest energy electronic state
electrons generally occupy bonding MOs and in some cases non bonding orbitals
Excited state:
Photon absorbtion induces an electronic transtion where an electron is excited to a higher energy level usually an antibonding MO
Explain and draw the different types of transition that can occur between MOs
When electrons occupy the same MOs but with different spins different excited states can be achieved.
What is multiplicity
Multiplicuty is used to describe the electron spin properties of a state
Defined as 2S+1
When an electron retains its spin on transtion multiplicity remains constant
When an excited state has a multiplicity of 1 it is called a singlet transition, when a transition has a multiplicity of 3 it is called a triplet transition
Give the labels for singlet and triplet transitions and the ground and excited state
How many triplet states are there?
Singlet = S
Triplet = T
Ground = 0
Excited = 1,2,3…
Each excited singlet state has an equivalent triplet state at lower energy (hunds rule states that subshells will preferentially fill singly beofre doubly).
There is no T0 state (Pauli exclusion principle says that 1 orbital cannot be doubly occupied with 2 electrons of the same spin).
Describe the rotational and vibrational degrees of freedom of atoms and molecules?
Atoms: no rotational or vibrational degrees of freedom
electronic energy levels are clearly defined
UV-visible spectra has sharp lines
Molecules: has rotational and vibrational degrees of freedom
eavh electronic state has many energy levels
UV-visible spectra has many lines or braod bands
Describe the Born-Oppenheimer approximation
states that the elctronic, vibrational and rotational properties are considered to be independent of each other
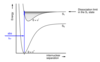
Where do electronic and vibrational transitions usually occur from and to?
Electronic trnsitions occur from the ground state (S0) to the first excited state (S1) when the longest wavelenght absorption arises
From v’’ = 0 in the S0 state (Boltzman states this is the most populated)
To: several v’ levels in the s1 state
Describe typical rotational energy transitions
Transition genrally occur from several J’’ rotational levels in the v’’ = 0 level (as Boltzman states that many J levels are populated) to several J’ leve;s in each v’ level in S1
Describe what typical spectra can look like
Rotational fine structure is seen from gases at high spectroscopic resolution
Vibrational structure seen from gases and liquids/solids in some cases but peak can be broad - particularly in polar solvents due to strong interations
What determines the required energy of a photon for a transition and the probability of the transition?
The energy of the photon (wavelength of light) is determined by the energy gap between the ground and excited states
The probability (stregth of absorption coeffiecient) is determined by selection rules
What is the overall selection rule for an electronuc transition?
The elctric field of the light must cause a displacement if charge giving a transition dipole moment (TM)
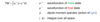
How do you apply the born oppenheimer approximation to the overall selection rule?
separate thr electronic anf vibrational wavefunction:
ψ = ψe . ψv
Separate ψe into electron spin (S) and orbital wavefuntions:
ψ = S . φ . ψv
Substitute into the original TM equation noting that the TM operator (μ)only acts on the orbital wavefunction
Tis gives three separate selection rukles: spin (S), orbital (φ) and vibrattion (ψv)
IF any of the integrals are 0 the TM is 0 and the transition if disallowed
What is the spin selection rule?
An electron has two possible spin states:
spin up (α) and spin down (β)
When the electron retains its spin on transition S’* and S’’ are both Sα or Sβ - The integral is normalised to and therefore = 1
If the spin changes on transition S’* = S’*α and S’’ = S’‘β or vice versa and the integral is orthogonal and therefore = 0
Rule: the electron must retain its spin on transition for the transition to be allowed (triplet excited state cannot be created efficiently by absorption.
Explain the orbital selection rule
The rbiotal selection rule has two parts:
symmetry and spatial
Symmetry rule: The orbital part of the transition can be split into three parts:
TMorbital = TMx + TMy + TMz
All three components must be 0 for the transition to be forbidden
Spatial: If the initial and final orbitals occupy a region of space that is similar the transition is allowed
Describe how to determine which transiotion are symmetry allowed
Deduce the point group
Deduce the symmetries (irreducibles) of the initial and final states (Γi and Γf)
Deduce the symmetries of the x, y and z components of the dipole moment operator (read from character table) (Γx, Γy, Γz)
Deduce three direct products
Γf x Γx x Γi - repeat for y and z
If any direct products gives totally symmetric represention (A1/Ag) the transition is symmetry allowed
Which transitions are allowed and forbidden under the spatial selection rule and what can affect the size of μ
π —–> π* have good spatial overlap and therefore are spatially allowed
n —-> π* occupy different regions of space and therefore are spatially forbidden
If there is a l;arge displacement of charge μ will be large
For a harmonic oscillator give the most probable geometries for v = 0 and v >> 0and give the most probable transitions
v = 0: most probably geometry (most probable point of finding particle) is at equilibrium geometry
When v >> 0 the most probably geometry is at the turning points
electronic transitions occur mainly from v’’ = 0 in the S0 state to v’ levels that give large vibrational overlap
What is the Franck-Codon Principal?
electrons move faster than nuclei because of their relative masses
There is no change in the position of atoms during a transition
Any change in geometry occurs after the transition.
Why is re (S1) often > re(S0)
an electron is promoted to an sntibonding orbital hence the excited state often has weaker, longer bond
What is the probability of a transition given by?
Oscillator strenght (f)
f is proprtional to the dipole moment (TM)
f is proportional to the integral of the absorbtion coefficient
oscillator strength for an electronic component:
f = fspin . fsymmetry . fspatial
What values of f are expected for allowed and disallowed transitions
if fully allowed: fx = 1
If disallowed:
fspin = 10-8 - 10-5
fsymmetry = 10-3 - 10-1
fspatial = 10-3 - 10-1
Allowed means high probability
Disallowed means lower probability
What is the breakdown of selection rules and explain the breakdown of the spin selection rule
The breakdown of selection rules is used to describe scenarios where the Born-Oppenheimer approximation does not hold well
The spin and orbital motions of electrons are not independant of each other
Spin orbit coupling magnitude is proportional to z4 (atomic number)
A spin flip transition is strongly forbidden but weakly allowed in the presence of heavy atoms
Internal - within a molecule
External - within other molecules (solvent)
Describe the breakdown of the symmetry selection rule
The vibrational and electronic motions are vibronic coupling
An electronic transition that is forbidden can be weakly allowed if the point group changes (symmetry lowers)due to an asymmetric vibration distorting the molecule
What is photo physics and what are the two processes of returning to ground state
Photophysics - the non radioactive decay of excited states
Excited states have a large excess of energy and are short lived
Losing ecess energy and returning to the ground state can accurr by two methods:
radiative
non radiative
Describe vibrational relaxation
Excited electronic states can be formed in a vibrationally excited state when excess energy is given to suurpunding molecules (sovent) in collisions - non radiative process
Rate constant (in liquids) = 1013 collisions s-1
Time scale = reciprocal of rate constant = 10-13 s
This usually occurs amoung the v’ levels as v’ =- 0 is the most populated
Describe luminesence and Fluorescence
Luminesence - a radiatuve transition (emission)
Fluoroesence - a radiative transition between states if the same multiplicity (S1 —-> S0)
What are the selection rules for decay processes?
The selection rules are the same as electronic absorption: spin, orbital and vibrational overlap integral
By definition fluoresence is spin allowed - typically the rate constant is high (kf = 106 - 109 s-1)
Genrrally is the decay process is allowed the rate constant is high
What does a gerneal absorbtion emission spetcrum look like
A vibrational progression from S1 (v’ = 0) to several S0 (v’’) levels
The probabilities are determines by the vibrational overlap integrals
The spectra are often mirror images
What are some general notes of spectra?
The spacing between individual peaks gives the spacing of vibrational energy levels in the final state
Absorbtion —-> v’ levels in S1 Emission —-> v’’ levels in S0
The spacing in absorption and emission spectra are slightly different because the vibrational energies are different in the S1 and S0 states - vibrational energies typically smaller in the S1 state because bonds are weaker
In gases the collision rate is lower in the S1 state so vinrational relaxation is slower - unrelaxed resonance fluoresence can be observed in higher v’ levels in gases
What is Stokes shift?
In solution the 0-0 band is typically shifted to a lightly lower energy in emission than absorption
This is because the solvent rearranges to stabalise the excited state after absorption but before emission
The extent of stabalisation varies with molecule and soolvent - polar systems generally give large shifts
What is internal conversion?
A non radiative isoenergetic transition between states of the same multiplicity - elevtron moved to s lower MO with dE = 0 and dS = 0
State formed is vibrationally excited
vibrational relaxation follows rapidly
What are the selection rules for non radiative transitions and what is vibrational overlap integral dependant on?
Similar rules to radiative transiotion: spin, orbital, vibrational overlap integral
By definition internal conversion is spin allowed
Vibrational overlap integral is based on energy and equilibirum geometry
Small energy gap = large overlap integral, very large rate constant
Large energy gap = small overlap integral, large rate constant
What is Kasha’s rule
Even if higher excited states are achieved internal conversion and vibrational relaxation occur very rapidly
fluoresence amd other processes usually occur exclusively from S1
In S1 state internal conversion and fluroesence are in competition
What is intersystem crossing
a non radiative, isoenergeitc transition between states of differing multiplicity - the molecule chnges state but may also change MO
vibrational relaxation follows rapidly
By definition is spin disallowed
For overlap ntegral Rate constants Kisc<<k>ic</k>
Small energy gap = large overlap integral, large rate constant
LArge energy gap = small overlap integral, very small rate constant
What do the relative rates of the different process depend on?
Depend of the rate constants
For many molecules intersystem conversion of an efficient way to form T1 states
T1 states arec relatively long loved
What is phosphoresence?
a radiative transition between states of different multiplicity (one step)
By definition spin disallowed - typically low rate constant
profile usually similar to fluoroesence spectra - vibrational overlap integrals are similar
Longer wavelength than in fluoroesence because the T1 state is lower energy tha fluoroesence
What are Jablonski diagrams?
Simple schematic diagrams taht summarise photophysical processes
Y axis = energy - usually qualitative but actual energies sometimes used
-v levels often shown butsome diagrams omit them
No parameter as x axis - states separated into stacks for ease
What is chemiluminescence?
Luminescence from an excited state formed asthe product of a chemical reaction
REleasing enough energy for this requires a strongly exothermic reaction
What is a quantum yield and what can it be applied to?
Quantum yield Φ: usually varies between 0 and 1
Φ = Number of molecules undergoing a process / number of photons absorbed
= rate of process / rate of absorption
What is Φf in terms of rate constants?
In the S1 state ; Φf = rate of S1 emission / Rate of S0 absorption
If S1 only decays by photophysics:
Φf + Φic + Φics = 1 and kf + kic + kics = ktot
Rate of absorption = Rabs, Rate of S1 emission = kf[S1] and Rate of S1 decay = (kf + kic + kics) [S1]
Under steady state conditions Rate of S0 absorption = Rate of S1 decay and Rabs = (kf + kic + kics )[S1)
Φf = kf [S1] / Rabs
Therefore: Φf = kf [S1] / (kf + kic + kisc) [S1]
= kf / (kf + kic + kisc)
What are quantum yields determined by and what steps need to be considered in a particular transition?
Determined by how the rate constant for a specific prpcess competes with the sum of the rate constants for all the decay processes
For a process from the T1 state the quantum yield of T1 formation (ΦT) and the fraction of molecules in the T1 state that decay by the process called a quantum efficiency θprocess
θ = No. molecules undergoing process / No. molecules undergoing all processes
= Rate of process / rate of all processes
What is the quantum yield of phosphorescence?
Φp = Rate of T1 emission / Rate of S0 absorption
Considering two steps: Φp = ΦT . θp
T1 is formed by isc so: ΦT = Φisc =
kisc / (kf + kic + kisc)
If T1 only decays by photophysics the θp = kp / kp + kisc
Φp = kisc / (kf + kic + kisc) . kp / kp + k’isc
How do you find the lifetime of a decay process
An excited state A* can decau by radiatuve processes, non radiative processes or other possible processes B and C
Rateof decay = ( kr + knr + kB + kC) [A*] = ΣK[A*]
Integrating gives: [A*]t = [A*]0e-Σkt
Lifetime τ = 1 / Σk
Lifetime if the time when [A*] falls to 1/e of its initial value
it enables conversion of rate constants to timescales
What are the types of lifetime?
Two types of lifetime:
- observed lifetime (actual) τobs
Can bemeasure in experiment τobs = 1/Σk = 1/kobs
- The radiative lifetime τ0
would be measured in experiment if decay was emission only - rarely measured as pther processes compete
Fluorescence and phosphorescence radiative lifetimes:
τf0 = 1/kf τp0 = 1/kp
Why are T1 states normally lopnger loived and what are typical observed lifetimes?
T1 states are usualluy longer lived because their photophysical decay routes are spin disallowed (low rate constant)
Typical observed lifetimes:
S1 τobs = pico seconds - nanoseconds
T1 τobs = micro seconds - seconds
Photophysical rate constants depend of the olecule as there may be competing non-photophysical processes
How does temperature affect rate constants?
generally has small effect on photophysical rate constants:
kf, kic, kisc, kp and k’isc
Can have a large effect on rate constants for other processes
when T decreases often kother decreases and Φphotophysical increases
Why can T1 states in the fluid solution decay faster than determined by kp and kisc
Non reactived decay can be caused by physical quenching
How do rate constants show that T1 lifetimes are shorter?
A typical quenchng rate constant = kq = 109 mol-1dm3s-1
even at low concentration [Q] = 10-9 mol dm-3 gives kq[Q] = 1s-1
This can out compete typical T1 state photophysical rate constant of kp = 0.05 s-1
Hence T1state lifetimes are shorter and photophysical quantum yields are smaller than determined by kp and k’isc
How is phosphorescence studied?
Generally physical quenching makes phosphorescence too weak to study in fluid solution
Instead it is studies in a rigid matrix when even if quens=chers are present they cannot diffuse
Typically studied at 77 K using a solvent that becomes a rigid c
glass (EtOH)
How do heavy atoms affect rate cinstants?
They incerase the rate cnstants for all spin flip processes due to spin-orbit couplinhg
kisc, kp, k’isc
What are steady state experimental techniques?
UV-visible absorption - gives spectrukm and aborption coefficients
UV-visible emission - gives spectrum and quantum yields
HWat are time-resolved techniques?
Excite with a light pulse and measure the time dependence of
wither emission intensity or absorbanvce
both are proportional to [A*]
Can give observed t
lifetime and rate constants or absorption spectra of excited states
Various designs with pulsed lasers and lamps: milli, nano … femtosecond time resolution possible
What is photochemistry?
The reactive decay of ezcited states
reactive processes compete with photophysical porcesses
Hiw can the reactivity of excited states differ from ground state
Electronic configuration: bonding, structure, redox ability
Excess energy: ability to overcome activation barriers
Lifetime: S1 states short lived
T1 states longer lived
What are some possibilities of reactions that can happen to an excited state
Reaction: subsitution, addition:
AB* + C —-> AC + B or ABC
Electron transfer:
AB* + C —-> AB.+ + C.- or AB<strong>.</strong>- + C<strong>.</strong>+
Energy transfer:
AB* + C —–> AB + C*
Isomerisation: AB* —-> AB’
What is unimolecular photochemistry and describe photodissociation.
Unimolecular photochemistry: an excited state elementary reaction involving one molecule as reactant
Photodissociation: on excitatiom the molecule dissociates
Above the dissociation limit the absorption band loses vibrational structure and becomes continuous
The fragments carry a continuous range of translational energies
What is photopredissociation?
a bound excited state is created initially but interval conversion to a dissociative state rapidly
due to the crossing of the potential energy curve from a dissciative state
the absorption band loses vibrational structure in the region of the crossing
How can sample conditions affect dissociation
In the gas phase the fragments mopve apart freely
In solution the solvent cage can keep fragments together long enough for geminate recombination to occur lowering the fragment yield
Examples of photodissociation
What is photoisomerisation?
On excitation the molecule undergoes a chang ein structure
In the ground state the barrier to isomerisation can be high
In the excited state the excess energy may overcome the barrier
A change in bonding may promote the change in structure
How does photoismoerisation affect alkenes?
In alkenes a π —> π* transition weakens the C=C bond so the most stable structure of the excited state is a twisted form
In general isomerisation of a trans or cis isomer gives a mixture of both.
Excitation of a sample of either isomer can give a mixture of bpth called a photostationary state
The composition depends on the relative absorbance of the isomers
If only one absorbs the conversion to the other can be 100%
What is photochromism?
A light induced reversible change in colour
This is often seen on switching between two isomers such as between cis and trans or ring opening
What is bimolecular photochemistry
An excited state elementary reaction involving two molecules
usually
A* + B ——–> products
What are excimers?
Systems hat form dimers in the excited state but usually not in the ground state
Excimer: an excited state dimer
Typically conjugated ring giving pi-stacking structures in the S1 state
Atb a low concentration of M the monomer fluorescence with vibrational structure
At high structure of M excimer fluorescemnce at longer wavelengthb without vibrational structure
What are Exiplexes?
Systems that form complexes in the excited state but usually not in the ground state
Exciplex: an excited state complex (MQ*)
At low concentrayion of Q monomer fluorescence with vibrational structure
At high concentration of Q exciplex fluorescence at longer wavelength without vibrational structure
Similarities and differences between eximers and exciplexes
Sim: fluorescence wavelength and vibrational structure characteristics
Potential energy curve characteristics
concentration and time dependence
Differences:
exciplexes are polar, charge transfer comlexes
significant degree of elevtron transfer between the two species
What is quenching?
The deactivation of an excited state
can be reactive or non reactive
the emission intensity (quantum yield ) is lowered
Scheme for biomolecular quenching
How do you fidn the Stern-Volmer equation
In the presemce of quencher Φfq = kf / (kf + knr + kq[Q])
In the absence of quencher: Φf0 = kf / (kf + knr)
Ratio = Φf0/Φfq = (kf + knr + kq[Q]) / (kf + knr)
Stern Volmer; Φf0/Φfq = 1 + (kq[Q]) / (kf + knr)
Can also be written: 1 + τobs0kq[Q]
τobs0 is the observed lifetime in the absence of quencher
What is the Stern-Volmer Equantion used for
determines quenching rate constants kq
- measure Φf at [Q] = 0 amd several [Q] concentrations
- plot Φf0/Φfq versus [Q]
- determine the gradient = τ0obskq
- using known value of τ0obskq cslculate kq
What is the diffusion controlled limit?
For many quenchers in non viscous solvents kq = 109-1010 sm3mol-1s-1 approachung the diffusion controlled limtit
the r.d.s is the reactants diffusing to meet
Can be estimated by the Debye equation
General trend Kdiff decreases as T decreases or as η increases
What are the thermodynamic and kinetics of quenching by electron transfer?
Between M* and Q and can be oxidative or reductive
Theremodynamics: ΔG = ΔG(M,Q –> M<strong>.</strong>+,Q<strong>.</strong>-) - E00 = ox
ΔG = ΔG(M,Q –> M<strong>.</strong>-, Q<strong>.</strong>+) - E00 = red
E00 = electronic excitation energy
The reaction is more favourable in the excited state due to E00
Kinetics: in general as ΔG = -ve, kq = kdiff
The back electron transfer can also be very fast reforming the reactants
When is the yield of electron transfer products the highest?
The ion yield is highest in polar solvents which can stabalise the products
What is an energy transfer?
The transfer of electronic excitation energy from an excited state donor molecule to an acceptor
D* + A ——> D + A*
The donor is deactivated to the griund state and the acceptor is promoted to anexcited state
Any emission or photochemistry of D* is replaced by that of A*
Processes occurring from A* are called sensitised
Describe the radiative mechanism of energy transfer
The donor emits a photon, the acceptor absorbs it
D* —-> D + hν emission
A + hν —–> A* absorption
For efficient energy transfer the emission of D* must iverlap with the absorption of A
The dominant energy transfer mechanism for molecules at large separation: solutions at low conc
Could occur over large distances - sun - earth
Describe long range coulombic mechanism of energy transfer
Energy transfer by dipole dipole interaction between D* and A without photon emission (non radiative) or contact
For efficient energy transfer the A/A* and D/D* energy gaps must match - energy release matches energy acceptance, emission overlaps with absorption
D* - A distance typically 2-10 nm (compared with molecular size this is a large distance)
Characteristics of long range coulombic energy transfer
Rate constant larger than diffusionm controlled limit because collision is not required: kE.T>kdiff
experimental rate constants and distances can be compared with those derived from theory
generally observed between singlet states
Describe the shirt range electron exchange mechanism of energy transfer
Energy transfer by an exchange of electrons between D* + A without photon emission at short distances < 2 nm
For efficient energy transfer
- the A/A* and D/D* energy gaps must match - energy released matches energy absorbed
- D* - A distance typically = 1 nm - similar to molecular size
Characteristics of shirt range electron exchange
rate cinstant typically approaches the diffusion controlled limit if the energy of A* lies below D*
kE.T = kdiff if E(A*) ≤ E(D*)
A wider range of possible transfers due to the detailed spin selection rules
What is photosensitisation?
Energy transfer to create T1 states that cannot be cerated readily
- cannot create directly by absorption (spin selection rule)
- quantum yield for intersystem crossing may be low
Ceate by triplet triplet energy transfer
D* (T1) + A (S0) —-> D(S0) + A* (T1)
Requires a donoro with high Φisc to create T1 and a T1 state energy that is higher than that of the acceptor T1
What is oxygen quenching?
Quenching by oxygen is quite common so air is often excluded from ophotochemical studies
