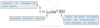Chapter 7 Waves Flashcards
Wave Motion
A wave is a means of transferring energy from one point to another in a fluid without the bulk movement itself through moving oscillations or vibrations (of particles themselves) which moves in the direction of you allowing you to hear
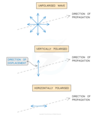
transverse and longitudinal waves
The oscillations/vibrations can be perpendicular or parallel to the direction of wave travel: When they are perpendicular, they are transverse waves When they are parallel, they are longitudinal waves
Ripple tanks
Waves can also be demonstrated by ripple tanks. These produce a combination of transverse and longitudinal waves -Ripple tanks may be used to demonstrate the wave properties of reflection, refraction and diffraction
General Wave Properties
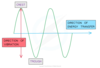
-Displacement (x) o -Amplitude (A) -Wavelength (λ) -These are all measured in metres (m) -Period (T) Measured in seconds (s) -Frequency (f) Measured in Hertz (Hz) or s-1 -Speed (v) Measured in metres per second (m s-1)
displacement
is the distance between points on successive oscillations of the wave that are in phase
amplitude
is the maximum displacement of a particle in the wave from its equilibrium position
wavelength
of a wave is the distance from its equilibrium position. It is a vector quantity; it can be positive or negative
period
or time period, is the time taken for one complete oscillation or cycle of the wave.
frequency
is the number of complete oscillations per unit time.
Speed (v)
is the distance travelled by the wave per unit time. Measured in metres per second (m s-1)
Phase
The phase difference tells us how much a point or a wave is in front or behind another
-This can be found from the relative positive of the crests or troughs of two different waves of the same frequency
–When the crests or troughs are aligned, the waves are in phase -When the crest of one wave aligns with the trough of another, they are in antiphase
Wave Energy
- Waves transfer energy between points, without transferring matter
- When a wave travels between two points, no matter actually travels with it:
–The points on the wave simply vibrate back and forth about fixed positions -Waves that transfer energy are known as progressive waves
-Waves that do not transfer energy are known as stationary waves
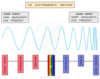
Cathode-Ray Oscilloscope
is a laboratory instrument used to display, measure and analyse waveforms of electrical circuits -The x-axis is the time and the y-axis is the voltage (or y-gain)
-An A.C. current on an oscilloscope
is represented as a transverse wave. Therefore you can determine its frequency and amplitude
Cathode-Ray Oscilloscope display showing wavelength and time-base setting. The period of the wave can be determined from the
time-base.
- This is how many seconds each division represents measured commonly in s div-1 or s cm-1
- Use as many wavelengths shown on the screen as possible to reduce uncertainties
- Dividing the total time by the number of wavelengths will give the time period T (Time taken for one complete oscillation)
- The frequency is then determined through 1/T
Using the definitions of speed, frequency and wavelength
v = f λ v= velocity (ms^-1) f=frequency(Hz or S^-1) λ=wavelength (m)
speed of a particle on a wave =
speed = distance/time
wave speed =
distance travelled by wave / time
The wave equation tells us that for a wave of constant speed
-As the wavelength increases, the frequency decreases -As the wavelength decreases, the frequency increases
Wave Intensity
-Progressive waves transfer energy -The amount of energy passing through a unit area per unit time is the intensity of the wave -Therefore, the intensity is defined as power per unit area
wave intensity =
power (w)/Area(m^2) The area the wave passes through is perpendicular to the direction of its velocity The intensity of a progressive wave is also proportional to its amplitude squared and frequency squared
Intensity is proportional
to the amplitude2 and frequency2 -This means that if the frequency or the amplitude is doubled, the intensity increases by a factor of 4 (22)
Spherical waves
- A spherical wave is a wave from a point source which spreads out equally in all directions
- The area the wave passes through is the surface area of a sphere: 4πr2
- As the wave travels further from the source, the energy it carries passes through increasingly
intensity at surface of sphere=
I=P/4pie(r)^2 the energy twice as far from the source us spread over four times the area, hence one-fourth the intensity
- Assuming there’s no absorption of the wave energy, the intensity I decreases with increasing distance from the source
- Note the intensity is proportional to 1/r2
- This means when the source is twice as far away, the intensity is 4 times less
- The 1/r2 relationship is known in physics as the inverse square law
Transverse waves
-A transverse wave is one where the particles oscillate perpendicular to the direction of the wave travel (and energy transfer) -Transverse waves show areas of crests (peaks) and troughs
Examples of transverse waves are:
-Electromagnetic waves e.g. radio, visible light, UV -Vibrations on a guitar string —-These can be shown on a rope —-Transverse waves can be polarised
Longitudinal waves
-A longitudinal wave is one where the particles oscillate parallel to the direction of the wave travel (and energy transfer) -Longitudinal waves show areas of compressions and rarefactions
Examples of longitudinal waves are
—-Sound waves —-Ultrasound waves -These can be shown on a slinky spring -Longitudinal waves cannot be polarised
Doppler Shift of Sound
This frequency change due to the relative motion between a source of sound or light and an observer is known as the doppler effect (or doppler shift) -When the observer (e.g. yourself) and the source of sound (e.g. ambulance siren) are both stationary, the waves are at the same frequency for both the observer and the source
When the source starts to move towards the observer,
the wavelength of the waves is shortened. The sound therefore appears at a higher frequency to the observer
Moving source and stationary observer
The frequency is increased when the source is moving towards the observer The frequency is decreased when the source is moving away from the observer
Calculating Doppler Shift
Properties of Electromagnetic Waves
-Visible light is just one part of a much bigger spectrum: The Electromagnetic Spectrum -All electromagnetic waves have the following properties in common: —They are all transverse waves —They can all travel in a vacuum —They all travel at the same speed in a vacuum (free space) — the speed of light 3 × 108 ms-1 —The speed of light in air is approximately the same
electromagnetic waves are transverse and consist of
-electric and magnetic fields oscillating at right angles to each other and to the direction in which the wave is travelling (in 3D space) -Since they are transverse, all waves in this spectrum can be reflected, refracted, diffracted, polarised and produce interference patterned
Uses of electromagnetic waves: radio (plus wavelength and frequency)
communication (radio and tv) -Wavelength: >0.1 -Frequency: <3 x 10^9
Uses of electromagnetic waves: microwave (plus wavelength and frequency)
-heating foods -communication( wifi,satellites) -Wavelength: 0.1 - 1 x 10^-3 -Frequency: 3 x 10^9 - 3 x 10^11
Uses of electromagnetic waves: infrared (plus wavelength and frequency)
-remote controls -fibre optics communication -thermal imaging (medicine and industry) -night vision -Heating or cooking things -Motion sensors -Wavelength: 1 x 10^-3 - 7 x 10^-7 -Frequency: 3 x 10^11 - 4.3 x 10^14
Uses of electromagnetic waves: visible light (plus wavelength and frequency)
seeing and taking pics or videos -Wavelength: 4 x 10^-7 - 7 x 10^-7 -Frequency: 7.5 x 10^14 - 4.3 x 10^14
Uses of electromagnetic waves: ultraviolet (plus wavelength and frequency)
-security marking -fluorescent bulbs -getting a suntan -Wavelength: 4 x 10^-7 - 1 x 10^-8 -Frequency: 7.5 x 10^14 -3 x 10^16
Uses of electromagnetic waves: x-rays (plus wavelength and frequency)
-x-ray images( medicine, airport security and industry) -Wavelength: 1 x 10^-8 - 4 x 10^-13 -Frequency: 3 x 10^16 - 7.5 x 10^20
Uses of electromagnetic waves: gamma rays (plus wavelength and frequency)
sterilizing medical instruments and treating cancer -Wavelength: 1 x 10^-10 - 1 x 10^-16 -Frequency: 3 x 10^18 - 3 x 10^24
Visible Light
-Visible light is defined as the range of wavelengths (400 – 700 nm) which are visible to humans -Visible light is the only part of the spectrum detectable by the human eye —However, this is only 0.0035% of the whole electromagnetic spectrum -In the natural world, many animals, such as birds, bees and certain fish, are able to perceive beyond visible light and can see infra-red and UV wavelengths of light
the electromagnetic spectrum (different waves and order)
1.radiowaves 2.microwaves 3.infrared 4.visible light(ROY G BIV) 5.ultraviolet 6.x-rays 7.gamma rays 1. lower energy, long wavelength, low frequency 7. higher energy, short wavelength, high frequency
To alternatively find the range of frequencies
convert the wavelengths using the wave equation: c = fλ where c is the speed of light: 3.0 × 108 m s-1
Polarisation of transverse waves
-Vibrations are restricted to one direction -These vibrations are still perpendicular to the direction of propagation/energy transfer
longitudinal waves cannot be polarised
since they oscillate parallel to the direction of travel
Waves can be polarised through a
polariser or polarising filter. This only allows oscillations in a certain plane to be transmitted
Malus’s Law
Malus’s law is used to find the intensity of light after passing through a number of polarising filters
Malus’s Law equation
The change in intensity against the angle of transmission axis
The half rule
When unpolarised light passes through the first polariser, half the intensity of the wave is always lost (Io/2)
Table of transmission depending on polariser orientation
Brewster’s angle
Brewster’s angle is an angle of incidence at which light with a particular polarisation is perfectly transmitted through a surface tan(angleb)=n2/n1 n1 is the refractive index of the initial material (in this case, air) n2 is the refractive index of the material scattering the light angleb=angle of incidence
Node
Is a point along a standing wave where it has minimum amplitude Displacement = 0
Anti nodes
Is the maximum displacement amplitude on a single wave
Measuring wavelength
Wavelength distance is the distance between two anti nodes with 2 nodes between them
