Chapter 24 - Magnetic fields

Question 1

What is a magnetic field?

Answer 1

Force field surrounding a magnet or current-carrying wire which acts on any other magnet or current-carrying wire placed in a field

Question 2

Where is a magnetic field of a bar magnet strongest?

Answer 2

At north-seeking or south-seeking poles

Question 3

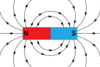
What is a line of force of a magnetic field?

Answer 3

Line along which a north pole would move in the field

Question 4

When will a wire in a magnetic field experience a force?

Answer 4

When it carries current

Question 5

What is the motor effect?

Answer 5

When a current carrying wire placed at non zero angle to the lines of force of an external magnetic field experiences a force due to the field.

Force is perpendicular to the wire and to the lines of force

Question 6

How can the direction of the force on a current carrying wire be worked out?

Answer 6

Fleming’s left hand rule

Question 7

What is the force on the current-carrying wire when the wire is parallel to the magnetic field

Answer 7

zero

Question 8

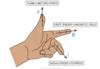
What does each finger represent in fleming’s left hand rule?

Answer 8

First finger = Field

seCond finger = Current

Thumb = Force

Question 9

What is magnetic flux density, B, of the magnetic field?

Answer 9

Force per unit length per unit current on a current-carrying conductor at right angles to the magnetic field lines

unit: Nm^-1A^-1 or Tesla (T)

Question 10

What is the equation for the force on a wire carrying a current in a uniform magnetic field, at 90º to the field lines?

Answer 10

F = BIL

where B = magnetic flux density

I = current

L = length of wire

Question 11

For a straight wire at angle theta to the magnetic field lines, how do you calculate the force on the wire?

Answer 11

Due to component of the magnetic field perpendicular to the wire, Bsin(angle)

therefore F = BILsin(angle)

Question 12

How can the magnnitude of a force on a current-carrying wire in a magnetic field be investigated?

Answer 12

Stiff wire frame is connected in series with a switch, an ammeter, a variable resistor, and a battery. When the switch is closed, the magnet exerts a force on the wire which can be measured from the change of the top pan balance reading.

Question 13

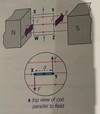
How is a couple created on a rectangular coil that has n turns in a magnetic field?

Answer 13

Long sides of the coil are vertical and each wire down each long side experiences a force BIL where L = length of each long side, therefore each wire experiences F = (BIL)n in opposite directions at right angles to the field lines.

The pair of forces acting on the long sides form a couple as the forces are not directed along the same line.

Question 14

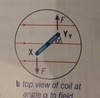
What is the equation for torque of the couple on a coil ?

Answer 14

Torque = Fd, where d = perpendicular distance between the line of action of the forces on each side. If the plane of the coil is at angle alpha to the field lines, then d = wcos(alpha) where w = width of coil

torque = Fwcos(alpha) = BLnwcos(alpha) = BIAncos(alpha) where A = lw

Question 15

What is the torque when the coil is parallel to the field?

Answer 15

alpha = 0 so the torque = BIAn as cos(0) = 1

Question 16

What happens to charged particles in a magnetic field?

Answer 16

experiences a force due to the magnetic field. Causes a beam of charged particles to be deflected

Question 17

Why does a current-carrying wire in a magnetic field experience a force?

Answer 17

Electrons moving along the wire are pushed to one side by the force of the field

Question 18

What is the difference between the direction of force on moving positive and negative charged particles when in a magnetic field?

Answer 18

Forces in opposite directions

Use fleming’s left hand rule for positive particles and right hand rule for negative particles

Question 19

What is the equation for force on a particle of charge moving in a magnetic field, perpendicular to the field lines?

Answer 19

F=BQv

where v = speed

as: I = Q/t and L = vt, so F=B(Q/t)vt

Question 20

What does it mean for a charged particle in a magnetic field, that the constant force is perpendicular to the velocity and field?

Answer 20

The particle travels in a circular path

Question 21

How does the constant force being perpendicular to the velocity mena it follows a circular path?

Answer 21

Force changes velocity (direction) but stays perpendicular to the velocity, meaning the path is a complete circle with the force always acting towards the centre of the circle. Force causes a centripetal acceleration

Question 22

What is the equation for the radius of a charged particle in circular motion acted on by a magnetic force?

Answer 22

BQv = mv²/r

r = mv/BQ

Question 23

when does the radius of a charged object in circular motion decrease?

Answer 23

• If B is increased or if v is increased
• If particles with a larger specific charge Q/m are used

Question 24

What is a cyclotron used for?

Answer 24

• Used in hospitals to produce high energy beams for radiation therapy
• Producing radioactive tracers

Question 25

Describe the structure of a cyclotron.

Answer 25

• Two hollow semicircular electrodes with alternating p.d.
• Slight gap between them
• Uniform magnetic field applied perpendicular to the plane of the electrodes

Question 26

Describe how a cyclotron works.

Answer 26

• Particle is fired into one of the electrodes
• The magnetic field makes it flow a semicircular path and return to the gap between electrodes
• The potential difference between them creates an electric field that accelerates the particle
• The velocity in now higher, so the particle takes a path with a larger radius before leaving the other electrode
• As it exits, the potential difference is reversed so that the electric field is reversed and therefore the particle can accelerate across the gap
• This repeats as the particle spirals outwards, increasing in speed, before exiting the cyclotron