applications of forces

Question 1

Answer 1

the box can move in any two directions , down or up, i figured out T when it goes up, but since the question asks for a “range of values”, you have to include the instance in which it goes down too. T minimum happens when the box moves down, T max ( The one i successfully worked out) occurs when the box goes up

Question 2

Answer 2

REMEMBER: THE COEFFICIENT OF FRICTION IS THE SAME AT ALL TIMES, HENCE WE CAN GET IT FROM BEFORE ANY FORCES ARE APPLIED !

Question 3

general steps to solve static rigid bodies (ie ladder questions )

Answer 3

1. resolve vertical and horizontal components
2. resolve moments

Question 4

how to draw ladders etc in static rigid bodies ?

Answer 4

R coming off teh bottom, N as contact force on the top

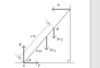
and friction going one way to the right !

Question 5

note

Answer 6

1. work out horizontal and vertical components

in this case, F=N

2. resolve and work out moments!

(mistake made here: Forgot about anticlockwise moment of “N * 8sin(theta) “ where 8 sin(theta) is the vertical component of the WHOLE ladder

Question 7

note

Answer 7

if unknown distance is mentioned… then assume that distance and call it “x”

Question 8

(HARD QUESTION ) MOST COMMON MISTAKE HERE !

Answer 8

not getting teh OVERALL HEIGHT OF THE LADDER so i can multiply the force thawt the wall exerts on the ladder by the perpendicular distance (overall HEIGHT OF THE LADDER ) to get the overall moment

in this case it was (2a)(cos30) for the perpendicular distance from P

Or (preffered method )

you could have done “nsin60 *2a, nsin60 being the HEIGHT

Question 9

continuing on from card 8 …

Answer 9

assign new forces… and solve

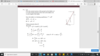
note, moments are around B , so take F into consideration ( multiply by perpendicular distance of Ico60)

Question 10

Answer 10

easy question: find moments about P, then individually find moments about Q

to find R1 AND R2

SILLY MISTAKE: i too kteh antivlockwise moment as just “S” , when i had to do “S *40 (PERPENDICULAR DISTANCE )

Question 11

what do you do if yo uwant to find the coeffcient of friction of something on an inclined plane

Answer 11

find the total horizontal force(which includes coefficient of friction ) then. equate tto f =ma (F being the total horizontal force)

t

Question 14

note: “acceleration” stays positive her !)

Question 15

Question 16

How to go about solving oulley problems

Answer 16

solve for each component ( vertical part) and horizontal part (being pulled by the pulley)

eg

note: vertical part is just equating forces oing upwards and downwards

Question 17

how to get coefficient of friction ? (in pulleys?