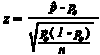1 Proportion Z Test Flashcards
(4 cards)
When do you use a 1 Proportion Z Test?
When one tries to test a given proportion against sample data. EXAMPLE: The CEO of a large electric utility claims that 80 percent of his 1,000,000 customers are very satisfied with the service they receive. To test this claim, the local newspaper surveyed 100 customers, using simple random sampling. Among the sampled customers, 73 percent say they are very satisified. Based on these findings, can we reject the CEO’s hypothesis that 80% of the customers are very satisfied? Use a 0.05 level of significance.
What is the general hypothesis for a 1 Proportion Z Test?
State the null hypothesis, and an alternative hypothesis. If one of the hypotheses are true, the other must be false. For example– Null hypothesis (Ho): P=0.8 Alternative hypothesis (Ha): P≠0.8 Note that these hypotheses constitute a two-tailed test. The null hypothesis will be rejected if the sample proportion is too big or if it is too small.
What are the conditions and assumptions for the 1 Proportion Z Test?
CONDITIONS: ◾The sampling method is simple random sampling. ◾Each sample point can result in just two possible outcomes. We call one of these outcomes a success and the other, a failure. ◾The sample includes at least 10 successes and 10 failures. (Some texts say that 5 successes and 5 failures are enough.) ◾The population size is at least 10 times as big as the sample size. ASSUMPTIONS: 1) SRS 2) Normality (Show that np>10 and n(1- p)>10
What is the formula for the test statistic for the 1 Proportion Z Test?