Thermodynamics Flashcards
From the definition of the various state functions to the properties of complex thermodynamic systems and processes, use these cards to master the topic of thermodynamics as tested in most introductory undergrad chemistry courses and on the AP Chemistry exam.
Define and give an example of:
a thermodynamic system
A thermodynamic system is a macroscopic body which is engaged in mass and/or energy exchange with its surroundings.
Ex: The classic example of a thermodynamic system is a piston filled with gas.
Define and give an example of:
an open thermodynamic system
An open thermodynamic system can exchange both mass and energy with the environment.
Ex: A bottle of gas with no lid is an open thermodynamic system.
Define and give an example of:
a closed thermodynamic system
A closed thermodynamic system cannot exchange mass with the environment, but can exchange energy.
Ex: A bottle of gas with the lid securely on is a closed thermodynamic system.
Define and give an example of:
an isolated thermodynamic system
An isolated thermodynamic system can not exchange either energy or mass with the environment.
Ex: A closed, double-walled (insulated) container which is temperature-independent of its environment is an isolated system.
Define “the surroundings” in a thermodynamic problem
The surroundings (also known as the environment) are everything capable of exchanging mass and/or energy with the system.
Define:
a state function
A state function is any property of a thermodynamic system that depends only on comparing the characteristics of the system at that moment compared to a prior moment.
Since state functions are calculated based on current vs past properties only, their values do not depend on the path by which the current state was achieved; they are path-independent.
If X is a state function, what is the change in X between a system’s values X1 and X2?
ΔX = X2 - X1
Since X is a state function, the path by which it gets from state 1 to 2 is irrelevant, the change in X depends only on the starting and finishing states.
Define:
enthalpy, H
Enthalpy is a measure of the heat contained in a system.
Enthalpy’s absolute value cannot be directly measured, so the change in enthalpy’s value, ΔH, is measured instead.
What are the properties of an exothermic reaction?
An exothermic reaction is any reaction whose products have a lower enthalpy than the reactants. Therefore, ΔH < 0, and heat is lost from the system to the environment.
(Exo = exit)
What are the properties of an endothermic reaction?
An endothermic reaction is any reaction whose products have a higher enthalpy than the reactants. Therefore, ΔH > 0, and heat is absorbed by the system from the environment.
(Endo = into)
Define:
a material’s standard enthalpy of formation, ΔHof
The standard enthalpy of formation (ΔHof) is the enthalpy change of the formation reaction for the material from its fundamental elements under standard conditions.

Ex: The enthalpy of formation for NaCl is -411.12 kJ mol−1 and follows from the general equation:
What is the standard enthalpy of formation, ΔHof, of oxygen gas, O2?
Zero.
By definition, the enthalpy of formation for any material in its standard state is zero.
What are standard conditions?
Standard conditions for a reaction require that:
- Pressure = 1 atm
- Temperature = 25º C = 298 K
- Concentration = 1 M for all products and reactants
If the chemical reaction
(1) 2A ⇒ C ΔH1
can be broken down into the sub-reactions
(2) 2A ⇒ B ΔH2
(3) B ⇒ C ΔH3
what does Hess’s Law tell you about the overall enthalpy change ΔH1?
ΔH1 = ΔH2 + ΔH3
Hess’s Law simply states that the enthalpy of a reaction can be calculated by adding together the enthalpies of a chain of sub-reactions which add up to the overall reaction.
Although most commonly applied to enthalpy, Hess’s Law applies to all state functions.
What is the enthalpy change when 2 moles of CH4 are formed, according to the following reactions?
rxn1: 2H2(g)⇒4H(g)
ΔH1= -870 kJ/mol
rxn2: C(s) + 4H(g)⇒CH4(g)
ΔH2= +794 kJ/mol
-152 kJ
Adding the reactions together yields the formation reaction of CH4:
C(s) + 4H(g) + 2H2(g) ⇒
CH4(g) + 4H(g)
Canceling common terms leaves:
C(s) + 2H2(g) ⇒CH4(g)
To complete the calculation, combine the reactions’ enthalpies in the same way the reactions were combined.
ΔHrxn = ΔH1 + ΔH2
-870 + 794 = -76kJ/mol
Finally, multiply by the number of moles (2) to get the final answer.
How can the enthalpy change of a reaction be calculated from the enthalpies of formation of the reactants and products?
∆Hºrxn= Σ∆Hºf(products)-Σ∆Hºf(reactants)
Sum the enthalpies of formation of the products, and subtract the sum of the enthalpies of formation of the reactants.
Is this reaction endothermic or exothermic?
CH4+ 2O2 ⇒ CO2+ 2H20
- ΔHof (CH4) = -75 kJ/mol
- ΔHof (CO2) = -394 kJ/mol
- ΔHof (H2O) = -286 kJ/mol
The reaction is exothermic.
∆H°rxn= Σ∆Hof(products)-Σ∆Hof(reactants)
= [CO2 + 2*H2O] - [CH4 + O2]
= [-394 kJ/mol + 2(-286 kJ/mol)]
- [-75 kJ/mol + 2(0 kJ)]
= -891 kJ/mol
Define:
bond enthalpy
Bond enthalpy is the energy absorbed or released when a particular chemical bond is broken.
Most chemical bonds are stabilizing, so most bond-breaking reactions are endothermic, and most bond enthalpies are positive.
How can the enthalpy of a reaction be calculated from the bond enthalpies of the reactants and products?
∆Hrxn = Σ∆H(bonds broken) - Σ∆H(bonds formed)
The reaction’s enthalpy change is identical to the energy needed to break all the bonds in the reactants, minus the energy released when the bonds in the products form.
What is the overall enthalpy change of this reaction?
CH4+ 2O2 ⇒ CO2+ 2H20
- ΔH (C-H) = 411 kJ/mol
- ΔH (O=O) = 494 kJ/mol
- ΔH (C=O) = 799 kJ/mol
- ΔH (O-H) = 463 kJ/mol
-818 kJ/mol
∆Hrxn = Σ∆H(bonds broken) - Σ∆H(bonds formed)
= [4*(C-H) + 2*(O=O)] - [2*(C=O) + 2*2*(H-O)]
= [(4 * 411) + (2 * 494)]
- [(2 * 799) + (4 * 463)] kJ/mol
= -818 kJ/mol
Define:
specific heat, c
Specific heat is a characteristic property of a material, and is the amount of heat which must be added to raise 1 g of the substance by 1ºC.
The higher the value of c, the more heat it takes to raise the substance’s temperature.
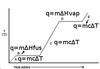
What is the formula for necessary quantity of heat in a specific heat problem?
q = mcΔT
Where q is the necessary heat, m is the mass of substance present, c is the substance’s specific heat, and ΔT is the desired temperature change.
What is the specific heat of water?
4.184 J/g*K
This is a value that you should have memorized. It means that it takes 4.184 J to raise 1 g of water by 1ºK.
This value is equal to 1 cal/g*K.
8 J of heat is applied to 1 g of both iron and water at 25ºC. Which changes temperature more?
The specific heat of water is 4.184 J/g-K.
The specific heat of iron is 0.46 J/g-K.
The iron changes its temperature more.
Applying the equation q = mcΔT to both cases and solving for ΔT reveals that the iron will change temperature by about 20 degrees (final T = 45ºC), while the water will only increase by 2 degrees (final T = 27ºC).
The higher a material’s specific heat, the less responsive its temperature is to heat flow.