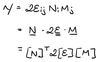Test 1 Material Flashcards
What is the Kronecker delta (definition)?
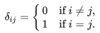
What is the permutation symbol (Levi-Civita) definition?
What is the transformation law for a tensor of rank n?
This description shows how a quantity (e.g., density, stress, velocity, etc) changes with time and is most convenient in solid mechanics.
Material or Lagrangian Description
(X , t)
This description shows how a quantity (e.g., density, stress, velocity, etc) changes in a particular (fixed) location in space and is most convenient in fluid mechanics.
Spatial or Eulerian Description
(x , t)
Give the equation/definition for the Deformation Tensor F
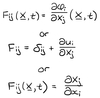
Write the equation for the stretch in terms of the right Cauchy Green deformation tensor and unit vectors
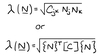
We use uppercase and lowercase letters to denote the reference and deformed configurations. Which one denotes which configuration?
Uppercase letters denote the reference configuration.
Lowercase letters denote the deformed configuration.
What is the equation for the cosine of the angle between two unit vectors in the reference configuration?
(Remember: this is the angle between the fibers in the deformed configuration that were in the direction of the unit vectors M and N in the reference configuration)
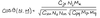
What is the stretch of the material when it’s aligned with the e1 direction of the reference configuration?
When it’s aligned with the e2 direction?
When it’s aligned with the e3 direction?
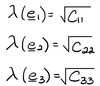
What is the change in angle (cos(theta)) between e1 and e2?
Between e2 and e3?
Between e1 and e3?
What is the equation for a differential oriented area with respect to the deformed configuration?
With respect to the reference configuration?
What is the equation for the Piola Transformation?
What is the equation for a change in volume?
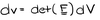
What is an equation for the right Cauchy Green tensor in terms of the deformation tensor?
In terms of the Lagrangian strain tensor?
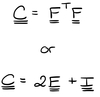
What is the equation for the Lagrangian strain tensor (also called the Green strain tensor) in terms of the right Cauchy Green tensor?
What is the equation for the Lagrangian strain tensor (also called the Green strain tensor) in terms of the deformation tensor?
(Remember: the diagonal of E describes the extensional strains in the direction of the basis vectors. The off-diagonal components of E describe the change in angles between the direction of the basis vectors)
What is the equation for the Lagrangian strain tensor (also called the Green strain tensor) in terms of extensional strains?
(Remember: the diagonal of E describes the extensional strains in the direction of the basis vectors. The off-diagonal components of E describe the change in angles between the direction of the basis vectors)
A deformation is said to be infinitesimal if…
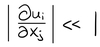
What is the equation for the infinitesimal strain tensor?
(these are the compatibility equations)
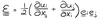
Deformation mapping is defined as…(equation)
Give the equation for stretch in terms of deformation for a finite stretch.
This is the exact stretch, not assuming infinitesimal strains.
Give the equation for stretch in terms of infinitesimal deformations or the infinitesimal strain tensor.
Give the equation for shear strain in terms of the infinitesimal strain tensor.
