Telescopes Flashcards
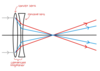
What is a Convex / Convering Lens
A lens that focuses incident light
Describe a real image being formed from a Convex Lens
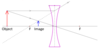
What is a Concave / Diverging Lens
A lens that spreads out incident light
Describe a diagram of light on a concave lens
What is the Principal Axis
The line passing through the centre of the lens at 90º to its surface
What is the Principal Focus
- In a converging lens - the point where incident beams passing parallel to the principal axis will converge
- In a diverging lens - the point from which the light rays appear to come from (This is the same distance either side of the lens)
What is the Focal Length
The distance between the centre of a lens and the principal focus
What is a Real Image and a Virtual Image
- Real image - formed when light rays cross after passing through the lens, can be formed on a screen
- Virtual image - formed on the same side of the lens. The light rays do not cross, so a virtual image cannot be formed on a screen
What is the Lens Formula
- 1 / u + 1 / v = 1 / f
- u is the distance of the object from the centre of the lens
- v is the distance of the image from the centre of the lens
- f is the focal length of the lens
What are the words used to describe an image that is formed
- Real or Virtual
- Magnified or Diminished
- Upright or Inverted
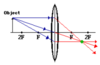
Describe the ray diagram for an object that is beyond 2F and describe the image’s apperance
Real, Inverted and Diminshed
Describe the ray diagram of an image that is very close to the lens and describe the image’s apperance
Virtual, Upright and Magnified
Describe the ray diagram of an image that is fairly close to the lens (between 2F and F) and describe the image’s apperence
Real, Inverted and Magnified
Describe the ray diagram of an image that is exactly 2F from the lens and describe the image’s apperence
Real, Inverted and same size
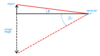
What is the Equation for Angular Magnification in Normal Adjustment
M = Angle subtended by the eye to the image/ Angle subtended by the eye to the object
What is the Equation for Angular Magnification in terms of the focal length
M = f0 / fe
Only used if both angles in other equation are below 10º
Describe the ray diagram for a refracting telescope in normal adjustment
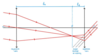
How does a Cassegrain telescope work
- There is a concave primary mirror and a small convex secondary mirror in the centre
- The light is collected by the primary mirror and focused onto the secondary mirror
- This is then reflected onto an eyepiece lens
Describe the Cassegrain Reflecting Telescope Ray Diagram
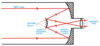
How do you minimise distortions in an image in the Cassegrain Telescope
- The mirrors in a reflecting telescope are a very thin coating of atoms
- This allows the mirrors to be as smooth as possible and minimises distortions in the image
What are the two types of aberration that can happen in a telescope
- Chromatic Aberration
- Spherical Aberration
What is Chromatic Aberration
- When a lens refracts different colours of light by different amounts as they have different wavelengths
- This causes the image for each colour to form in a slightly different position, causing coloured fringes around the image
- Since chromatic aberration is caused by refraction, it has very little effect on reflecting telescopes as it only occurs in the eyepiece lens.
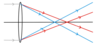
What is Spherical Aberration
- The curvature of a lens or mirror can cause rays of light at the edge to be focused in a different position to those near the centre, leading to image blurring and distortion
- This effect is most pronounced in lenses with a large diameter, and can be avoided completely by using parabolic objective mirrors in reflecting telescopes
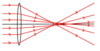
What is an Achromatic doublet
- An achromatic doublet consists of a convex lens and a concave lens cemented together in order to bring all rays of light into focus in the same position
- It minimises spherical and chromatic aberration
What are the advantages of using a Reflecting telescope
- Mirrors are mostly unaffected by chromatic aberration
- Spherical aberration can be solved by using parabolic mirrors
- Collects more light so better resolution
- Compact and portable
What are the disadvantages of using a Refracting Telescope
- Large magnifications require very large diameter objective lenses with very long focal lengths
- Heavy and bulky therefore can be difficult to manoeuvre
- Can be affected by chromatic aberration
What is a Radio Telescope
Radio telescopes use radio waves to create images of astronomical objects
Describe the similarities and differences of radio telescopes compared to optical telescopes
Similarities
- Both types of telescopes function in the same way: they intercept and focus incoming radiation to detect its intensity
- Both can be moved to focus on different sources of radiation or track a source
- Both have a parabolic dish curved dish (to collect radiation)
- Both collect electromagnetic radiation
- Both optical and radio can be built on the ground since both radio waves and optical light can pass easily through the atmosphere
Differences -
- Since radio wavelengths are much larger than visible wavelengths, radio telescopes have to be much larger in diameter than optical telescopes in order to achieve the same quality image/have the same resolving power
- Construction of radio telescopes is cheaper and simpler because a wire mesh is used instead of a mirror
- A radio telescope must move across an area to build up an image, unlike optical telescopes
- Unlike optical telescopes, Radio telescopes experience a large amount of man-made interference from radio transmissions, phones, microwave ovens. Optical telescopes experience interference from the weather conditions, light pollution, stray radiation
Explain the structure, positioning and uses of an infrared telescope
- Structure - large concave mirror focusing light onto a detector. Must be cooled with cryogenic fluids to absolute zero to avoid interference
- Positioning - must be in space as infrared light is blocked by the atmosphere
- Uses - observing cooler regions in space (from a few tens to 100K)
Explain the structure, positioning and uses of an ultraviolet telescope
- Structure - cassegrain configuration that focuses radiation onto solid state devices
- Positioning - must be in space as ultraviolet light is blocked by the ozone layer
- Uses - observing the interstellar medium and star formation regions
Explain the stucuture, positioning and uses of an x-ray telescope
Structure - combination of hyperbolic and parabolic mirrors to focus radiation onto a CCD
Positioning - must be in space as x-rays are blocked by the atmosphere
Uses - observing high-energy events and areas such as active galaxies, black holes and neutron stars
Explain the structure, positioning and uses of a gamma telescope
Structure - no mirrors, radiation passes through a detector made of layers of pixels
Positioning - must be in space as gamma rays are blocked by the atmosphere
Uses - observing gamma ray bursts, quasars, black holes and solar flares
Compare the resolving and collecting powers of infrared and ultraviolet telescopes
- The collecting power of infrared and ultraviolet telescopes is similar to that of an optical telescope, because their diameters are similar
- However, the resolving power of an ultraviolet telescope is better than an optical telescope of the same diameter - this is because ultraviolet light has a shorter wavelength than visible light whereas the wavelength of infrared radiation is longer than that visible light
What is Collecting Power
A measure of the ability of a lens or mirror to collect incident EM radiation
What is Collecting Power directly proportional to
- Directly proportional to the area of the objective lens
- Directly proportional to the sqaure of the diameter
What is Resolving Power
The smallest angle of speration at which two points can be distinguished
What is the Rayleigh Criterion
The Rayleigh Criterion states that two objects can just be resolved if any part of the central maximum of either of the images falls within the first minimum diffraction ring of the other
What is the Rayleigh Criterion Equation
θ = λ / D
Minimum angular resolution = Wavelength of radiation / diameter of the objective lens
Smaller angle means higher resolution
What are Charged Couple devices
An array of light-sensitive pixels, which become charged when they are exposed to light by the photoelectric effect
What is Quantum Efficiency
The percentage of incident photons which cause an electron to be released
What is Spectral Range, Pixel Resolution and Spatial Resolution
- Spectral range - the detectable range of wavelengths of light
- Pixel resolution - the total number of pixels used to form the image on a screen
- Spatial resolution - the minimum distance two objects must be apart in order to be distinguishable
Compare the human eye and CCD as detectors in terms of quantum efficiency, resolution, and convenience of use
