Number Properties Flashcards
Is 0 odd or even?
Is 0 positive or negative?
0 is even.
0 is neither positive or negative. Problems often use the phrase “positive integers”– read carefully and realize they are talking about 1, 2, 3…. etc
What is 5 - (9 - x) ?
-4 + x
Be sure to distribute the - sign!
The double negative becomes a positive
5 - 9 + x
If xy < 0 , what can we conclude about x and y?
x and y have opposite signs:
one is positive, one is negative
If xyz > 0 , what can we conclude about x, y and z?
All 3 can be positive, OR there are 2 negatives and 1 positive
If y is an integer, what determines whether (-2)y is positive or negative?
If y is even, (-2)y is positive
If y is odd, (-2)y is negative
e.g. (-2)4 = -2 * -2 * -2 * -2 = 16
(each pair of negative signs cancels each other out)
(-2)5 = (-2)4 * -2 = 16 * -2 = -32
(there is 1 negative sign left without a pair)
What is (-2)3 * 1/2 * -3 * -1/2
-6
First, determine whether it’s positive or negative
There are an ODD # of negative signs, so it will be negative.
-8 * 1/2 * -3 * -1/2 = -6
If xy < 0, what can we conclude about x and y?
x must be negative
y can be positive or negative but can’t be an even integer
(-2)3 = -8
(-2)-3 = -1/8
(-2)4 = 16
If x is an integer, what can we conclude about 2x?
2x must be even
even * any integer = even
If b is odd, which must be even? E: 2b + 2
A) 2b - 3 –> 2b is even –> even - odd = odd
B) (b-1) / 2 –> even / 2 = even?
If b is a multiple of 4, yes. If not, no. (4/2 = 2 , 6/2 = 3)
C) b/2 must be a fraction, since odd means NOT a multiple of 2 (3/2 = 1.5)
D) odd*odd + even = odd + even = odd
E) even + even = even
What are the first 10 prime numbers?
2, 3, 5, 7, 11, 13, 17, 19, 23, 29
If x is divisible by y, how can we write that?
x / y = Integer
x = y * Integer
We can also say that y is a Factor of x,
and x is a Multiple of y
How do you tell if an integer is divisible by 2?
by 3?
by 4?
by 5?
by 6?
by 8?
by 10?
2: It’s even
3: The sum of the digits is a multiple of 3
4: Divisible by 2 twice, or the last 2 digits are divisible by 4
5: ends in 0 or 5
6: Divisible by both 2 and 3
8: Divisible by two 3 times, or the last 3 digits are divisible by 8
10: ends in 0
If x/2 is even, what can we conclude about x?
X must be a multiple of 4
“Even” means a multiple of 2
x/2 = even
x = 2 * even
x = multiple of 4
1) If the units digit of N is 0, what do we know about the factors of N?
1) The factors include 2 and 5, because N must be a multiple of 10.
(For any number we want to factor that ends in one or more 0’s, it is easiest to factor the 10’s out first)
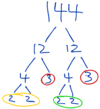
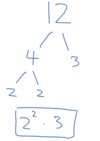
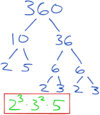
What is the prime factorization of 360?
(Draw a factor tree and then write the exponents)
Example:
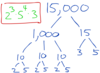
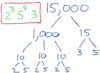
If the integer N ends with “5000”, what do we know about the factors of N?
From the 3 zeros at the end, the factors include 3 pairs of 2’s and 5’s, because N is a multiple of 1000.
Once we factor out 1000, we know that there is another 5 as a factor, since N/1000 would end with a 5.
Factors include 23 * 54
The image below shows an example of 15,000:
What is the remainder for 75/8?
What is 75/8 as a mixed number?
Remainder is 3. 9 3 /8
The remainder is the number left over after it is divided evenly.
If there were 75 cookies divided evenly among 8 people, each person would get 9, with 3 left over.
The 3 left over is 3/8 for each person.
If x is the product of the integers from 1 to 10, inclusive, what is the greatest integer n for which 2n is a factor of x?
8
We can represent x as 10! (10 Factorial)
x = 10! = 10*9*8*7*6*5*4*3*2
The question boils down to “How many 2’s are in the prime factorization of x?”
Only the even numbers have 2’s as factors:
2 has 1
4 has 2 (22)
6 has 1 (2*3)
8 has 3 2’s (23)
10 has 1 (2*5)
Add up all the 2’s: 1+2+1+3+1 = 8
If x is the square of an integer, what must be true about its factors?
The prime factors must have Even Powers
(the factors must match up in pairs)
example: 144 = 12*12 = 24 * 32
