Lecture 2

Question 1

• Interaction of the electron spin with the nuclear ‘… …’/’spins’ of atoms gives rise to … … providing the nuclear spin of the atom is non-…
• This arises when an external magnetic moment, B is present that … electron and nuclear magnetic dipoles giving an … hyperfine

Answer 1

• Interaction of the electron spin with the nuclear ‘magnetic moments’/’spins’ of atoms gives rise to hyperfine structure providing the nuclear spin of the atom is non-zero
• This arises when an external magnetic moment, B is present that aligns electron and nuclear magnetic dipoles giving an isotropic hyperfine

Question 2

• Describe the isotropic contribution of the hyperfine to the hyperfine energy

Answer 2

• Isotropic Hamiltonian relates directly to strength/energy of hyperfine interaction –> Fermi contact interaction
• This includes the wavefuntion, ψ(0), which is the unpaired electron wavefunction at the nucleus

Question 3

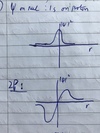
• Using a sketch to aid your answer, describe the wavefunction on a nucleus and the probability of finding an electron in the 1s and 2p orbital. How does this relate to the Fermi contact?

Answer 3

• 1s: Finite probability of finding electron at r = 0, would expect hyperfine to be non-zero
• 2p: Probability of finding electron at r = 0 is 0

–> only get fermi contact interaction if unpaired electron is in an s type orbital

Question 4

• In a ^•CH₃ radical, unpaired electron not in an s orbital still produces a hyperfine interaction, why is this?

Answer 4

• Electron magnetic field interacts through space with hydrogens producing a dipole-dipole interaction –> Anisotropic interaction; splitting of which depends on orientation of dipoles
• Hyperconjugation also spreads density across molecule ( see McConnell equation – lec 4)

Question 5

• When must the anisotropic term be accounted for in the hyperfine energy?

Answer 5

• In high viscosity liquids/ solids where rapid tumbling of radicals is not present, meaning anisotropic hyperfine interaction is non-zero and should be not be ignored

Question 6

• State the Zeeman resonance equation and use it to describe the origin of states in an unpaired electron in a magnetic field

Answer 6

• ΔE = hv = gµ_BB
• An electron has spin = ½ aligned parallel (m_s = + 1/2) or anti parallel (m_s = - 1/2)
• Interactions of magnetic moment with magnetic field (gµ_BB*m_s) cause energy levels to split as field, B increased
• Radiation absorption matching energy separation causes a transition between the 2 states

Question 7

• Describe two methods of analysing the environment of an unpaired electron via the Zeeman splitting constant

Answer 7

• g_e = 2.0023, exact value for free electron in vacuum, value differing from this implies electron is interacting with others/in an electric field

Question 8

• Electrons have spin/magnet moment, nuclei surrounding it with their own … …(e.g proton - m_I = … ). This contribution is seen by electron as well as the … magnetic field and this contribution scales with …. The electron magnetic moment >> nuclear magnetic moment

Answer 8

• Electrons have spin/magnet moment, nuclei surrounding it with their own magnetic field (e.g proton - m_I = ± ½). This contribution is seen by electron as well as the applied magnetic field and this contribution scales with distance. The electron magnetic moment >> nuclear magnetic moment

Question 9

• Sketch and label the energy level splitting diagram for a S = ½, I = ½ system, give the overall energy of the interaction as well as the transitions possible between levels

Answer 9

Question 10

• How does the value of hyperfine splitting relate to the transitions in a S = ½, I = ½ system? specifically the resulting wavepatterns of these transisitons

Answer 10

• 1 derivative due to field modulation of peaks to increases sensitivity
• Peaks due to interaction of nucleus and electron give hyperfine splitting, a

Question 11

• What is the electron Zeeman interaction?

Answer 11

• When in a magnetic field, B and unpaired electron/radical (S = ½) will split into 2 states
• A low energy state, which is aligned with B: m_s = - ½
• A high energy state which is aligned against B: m_s = + ½

Question 12

• What is the electron-nuclear hyperfine interaction?

Answer 12

• Nuclei of atoms with S ≥ ½ possess a magnetic moment, which produces a local magnetic field at the electron resulting in hyperfine splitting with Zeeman splitting
• E = M_saM_I
• From this, info on distribution of the unpaired electron probability density can be attained.

Question 13

• What are the EPR selection rules for transitions between energy levels

Answer 13

• Δm_s = ± 1
• Δm_I = 0

Question 14

• How is the energy change associated with hyperfine transitions in an S = ½ nuclei and I = ½ system related to the magnetic field?

Answer 14

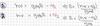
Question 15

• Sketch the energy level splitting for a S = ½ and I = ½ system where the nuclear Zeeman splitting is greater than the hyperfine. Include transitions and the hyperfine splitting profile associated with this system

Answer 15

• a written before as larger than Zeeman, so interacts first
• N.B. Energy level diagram may be given one way round in terms of splitting then change part way through the question

Question 16

• Sketch the energy level splitting for a S = ½, I = ½, I = ½ system where the hyperfine is greater than the nuclear Zeeman splitting. Include transitions and the hyperfine splitting profile associated with this system

Answer 16

Note difference in splitting profile

2 nuclei therfore 2 hyperfine splittings followed by 2 nuclear zeeman splittings

Question 17

• Give the Binomial distribution for n equivalent I = ½ nuclei and I = 1 nuclei

Answer 17

• I= ½ (protons), 1 (electron coupled to N)

Question 18

• Sketch the splitting of a ^•CHCH₂ radical

Answer 18

• 1 proton environment splits in to 2 with radical electron
• 2 equivalent proton splits cause 1 2 1 splitting when interacting with radical electron
• Get a doublet of triplets
• Larger splitting for environment closer to radical (stronger coupling)

Question 19

• Sketch the splitting of the naphthalene anion radical

Answer 19

Question 20

• What is the expected hyperfine splitting from the following molecule?

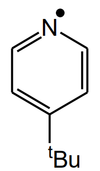
Answer 20

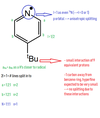
Question 21

• Tips for hyperfine splitting pattern analysis
  
  • Start at edge as … splitting shifts something out to edge
  • Use a … to measure distances when reading across spectrum

Answer 21

• Tips for hyperfine splitting pattern analysis
  
  • Start at edge as biggest splitting shifts something out to edge
  • Use a ruler to measure distances when reading across spectrum