Brainscape's Knowledge GenomeTM


Top Flashcards (Certified)
All Flashcards

CSET Math II > Geometry > Flashcards

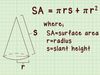
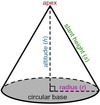
Geometry Flashcards

1
Q

Collinear

A

Three or more points on same line

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
2
Q

Line

A

Defined by two distinct points and contains an infinite number of points

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
3
Q

Segment

A

A line bounded at both ends

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
4
Q

Segment Bisector

A

A line that goes through the midpoint of a segment, dividing the segment into two equal parts

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
5
Q

Perpendicular AKA

A

AKA Orthogonal

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
6
Q

Perpendicular lines

A

Meet at 90* right angles

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
7
Q

Perpendicular line slopes

A

Negative reciprocals

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
8
Q

Parallel line slopes

A

Identical slopes

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
9
Q

Distance Formula

A

√ (x2-x1) ^2+ (y2-y1)^2

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
10
Q

Slope

A

(x2-x1) / (y2-y1)

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
11
Q

Midpoint (between two points)

A

[(x1+x2)/2 , (y1+y2)/2]

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
12
Q

Angle

A

The intersection of two non-identical lines

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
13
Q

Angle Bisector

A

A line through the vertex that divides the angle into two equal angles (point is equidistant from sides).

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
14
Q

Vertex

A

The point of intersection

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
15
Q

Coplanar Angles

A

Lie in the same plane but are not necessarily adjacent

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
16
Q

Adjacent Angles

A

Share a common side

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
17
Q

Vertical Angles are…

A

Congruent (Angles opposite each other where two lines cross)

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
18
Q

Acute angle

A

Less than 90*

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
19
Q

Right angle

A

Equals 90*

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
20
Q

Obtuse angle

A

Greater than 90*

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
21
Q

Linear pair

A

Two angles that form a straight angle

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
22
Q

Congruent figures

A

Same shape and same size

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
23
Q

Congruent Triangles proofs

A

Side-Angle-Side, Side-Side-Side, Angle-Side-Angle, or Angle-Angle-Side

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
24
Q

Similar figures

A

Congruent angles and proportional sides

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
25
Q

Relation

A

Mapping of input values to output values, or x to y

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
26
Q

Reflexive relation

A

Can map x to itself (ie: x=x)

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
27
Q

Symmetric relation

A

If a=b, then b=a

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
28
Q

Transitive relation

A

a=b, b=c, therefore a=c

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
29
Q

Equivalence Relation

A

Is reflexive, symmetric and transitive

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
30
Q

Irregular/Scalene Triangle

A

Has NO sides that are the same length and NO angles that are the same measure

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
31
Q

Scalene Triangle AKA

A

AKA Irregular Triangle

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
32
Q

Isosceles Triangle

A

2 sides that are the same length and 2 angles that are the same measure

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
33
Q

Equilateral Triangle

A

All 3 sides of equal length and all 3 angles of equal measure

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
34
Q

If a² + b² = c²

A

Triangle is right

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
35
Q

If c² (longest side) is more than a² + b²

A

Triangle is obtuse

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
36
Q

If c² (longest side) is less than a² + b²

A

Triangle is acute

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
37
Q

Area (of a triangle)

A

(1/2) base x height

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
38
Q

Perimeter (of a triangle)

A

Sum of Sides

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
39
Q

Longest side (of a triangle) is opposite which angle?

A

Largest Angle

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
40
Q

Shortest side (of a triangle) is opposite which angle?

A

Smallest Angle

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
41
Q

Median of a triangle

A

Bisects a side and passes through opposite vertex

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
42
Q

Perpendicular Bisector of a triangle

A

Splits side, right angle to side

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
43
Q

The Exterior Angle of a triangle equals…

A

The sum of the two remote interior angles

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
44
Q

Side of a triangle must be less than…

A

The sum of the other two sides

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
45
Q

Side of a triangle must be more than…

A

The difference of the other two sides

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
46
Q

For 45-45-90 triangles, the hypotenuse is…

A
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
47
Q

For 30-60-90 triangles, the length of the longer leg is ___ times the length of the shorter leg

A

√3

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
48
Q

For 30-60-90 triangles, the hypotenuse is ___ the length of the shorter leg

A

2x

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
49
Q

Sine = (Ratio only true for right angles)

A

opposite/hypotenuse

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
50
Q

Cosine = (Ratio only true for right angles)

A

adjacent/hypotenuse

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
51
Q

Tangent = (Ratio only true for right angles)

A

opposite/adjacent

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
52
Q

Cosecant = (Ratio only true for right angles)

A

1/sine

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
53
Q

Secant= (Ratio only true for right angles)

A

1/cosine

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
54
Q

Cotangent = (Ratio only true for right angles)

A

1/tangent

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
55
Q

Law of Cosines

A

a² = b² + c² - 2bc cosA

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
56
Q

Ray

A

A line that begins at an initial point and extends infinitely in one direction

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
57
Q

Altitude

A

A segment perpendicular to a side that goes through the opposite vertex (all meet at a point which might be outside the triangle)

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
58
Q

Triangle midline

A
  • A line segment from midpoint of one side to midpoint of another
  • Length equals half the third side
  • Midline parallel to third side
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
59
Q

Geometric mean between a and b

A

x^2 = ab (ie: geometric mean between 4 and 9 = 6).

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
60
Q

Radius (of a circle)

A

Distance from center to the circle

61
Q

Diameter (of a circle)

A

2r distance between opposite points on the circle

62
Q

Area (of a circle)

63
Q

Circumference (of a circle)

A

2πr or πd

64
Q

Arc (of a circle)

A

a portion of the circumference

65
Q

Minor Arc (of a circle)

A

less than 180*

66
Q

Major Arc (of a circle)

A

greater than 180*

67
Q

Chord (of a circle)

A

a segment with both ends on the circle

68
Q

Secant (of a circle)

A

containss a chord and extends beyond the circle

69
Q

Tangent (of a circle)

A

touches a circle at single point

70
Q

Central Angle (of a circle)

A

An angle formed by two radii with the vertex at the center of the circle. This measure equals the arc it defines.

71
Q

Measure of an Inscribed Angle (of a circle) is equal to…

A

1/2 the measure of the intercepted arc

72
Q

Circumscribed figures (of a circle) are located on the…

73
Q

Inscribed figures (of a circle) are located on the…

74
Q

Area of a Sector is proportional to the…

A

central angle

75
Q

A (sector)

A

pi r^2 (theta/360)

76
Q

Quadrilateral

A

4 sided, sum of interior angles = 360

77
Q

Parallelogram

A

A quadrilateral with 2 sets of parallel sides

78
Q

Rectangle

A

A quadrilateral [and special type of parallelogram] with 4 right angles

79
Q

Square

A

a rectangle with four equal sides

80
Q

Rhombus

A

A quadrilateral with all sides of equal length

A parallelogram with four equal sides but not necessarily with 4 right angles. The diagonals are perpendicular

81
Q

Trapezoid

A

has two parallel bases

82
Q

Regular polygon

A

has equal sides and equal angles

83
Q

Sum of interior angles of an n-gon =

84
Q

Apothem

A

The perpendicular bisector of a side that extends to the center of the polygon

85
Q

Area of a regular polygon =

A

1/2 (Apothem x Perimeter)

86
Q

Exterior Angle of a Polygon is created by….

A

extending one of the sides

87
Q

The sum of the exterior angle and the adjacent angle of a polygon =

A

180, a linear pair

88
Q

The sum of all exterior angles (of a polygon) in the same direction =

89
Q

Midpoint between two points (a,b) and (c,d)

A

( [a+c]/2, [b+d]/2) think of the avg of the x coords, and avg of y coords

90
Q

Distance between two points (a,b) and (c,d)

A

sq rt [(a-c)^2 + (b-d)^2]

91
Q

Area of a trapezoid

A

1/2 (b1+b2) (h)

92
Q

Complementary Angles

A

Two angles that add up to 90*

93
Q

Supplementary

A

Two angles that ad up to 180

94
Q

If A=C in equation: Ax^2+Bx+Cy^2+Dy+E

95
Q

If A≠C, but same sign in equation: Ax^2+Bx+Cy^2+Dy+E

96
Q

If A=0 or C=0 in the equation: Ax^2+Bx+Cy^2+Dy+E

97
Q

If A and C are opposite sign in the equation: Ax^2+Bx+Cy^2+Dy+E

98
Q

directrix

A

a line in the plane

99
Q

eccentricity

A

a measure of the shape. the closer to zero, the closer the shape is to a circle.

100
Q

two parallel lines, two intersecting lines, or three points define a….

101
Q

two lines that are not parallel and do not meet are…

102
Q

A line that is perpendicular to a plane is perpendicular to all lines in the plane that passes through the point of…

A

intersection

103
Q

A line that intersects a plane must be perpendicular to at least ___ line in the plane

104
Q

Prism

A

has two congruent, parallel bases (a triangular prism has two parallel triangle bases, a rectangular prism has 2 rectangular bases, etc)

105
Q

Volume of a Prism

A

BH (base area * height)

106
Q

Surface Area of a Prism

A

2xBase Area + Lateral Area

107
Q

Volume of a Cylinder

108
Q

Surface Area of a Cylinder

A

2 π r h + 2 π r^2

109
Q

Volume of a Cone

110
Q

Surface Area of a Cone

111
Q

Slant Height of a Cone

A

apply pythagorean theorem

square root of altitude^2 + radius^2

112
Q

Volume of a Pyramid

A

1/3 x Base area x Height

113
Q

Surface Area of a Pyramid

A

B + (Perimeter x slant) / (2)

114
Q

Volume of a Sphere

115
Q

Surface Area of a Sphere

116
Q

Cross Section

A

Made by slicing through a 3D object to produce a 2D polygon

117
Q

The cross section of a sphere is a…

118
Q

The cross section of a triangular prism is a…

A

triangle or rectangle

119
Q

The cross section of a pyramid with a square base is a…

A

square or triangle

120
Q

Transformation

A

changes the position of a shape on a coordinate plane — shape is moving from one place to another

121
Q

Flip/Reflection

A

A shape is transposed across a line and faces the opposite direction

122
Q

Slide/Translation

A

An entire shape moves in a straight path. All points on the shape move an equal distance in the exact same direction

123
Q

Turn/Rotation

A

A shape moves in a circular path around an axis point

124
Q

Isometry

A

Transformation in two or three dimensions that preserves distance, shape and size

125
Q

Dilation

A

Transformation that retains shape but changes size. Resulting image is in proportion (or scale) to original

126
Q

Similar shape

A

congruent angles and proportional sides

127
Q

If the side of a square is doubled, the area increases by a factor of…

128
Q

If the side of a cube is doubled, the volume increases by a factor of…

129
Q

If the radius of a circle is doubled, the circumference is doubled, and the area increases by a factor of…

130
Q

pentagon

131
Q

hexagon

132
Q

octagon

133
Q

heptagon

134
Q

nonagon

135
Q

decagon

136
Q

trapezoid

A

a convex quadrilateral with at least one pair of parallel sides

137
Q

Centroid (of a circle) how to find…

A

The point of concurrency for the medians of a triangle (bisecting each line segment). Centroid is the point of balance for any triangle.

138
Q

Incenter (of a circle) how to find…

A

The point of concurrency of the angle bisectors

139
Q

Circumcenter (of a circle) how to find…

A

The point of concurrency of the perpendicular bisectors

140
Q

Conversion formulas to convert from polar coordinates to rectangular coordinates.

A

x = (r)cosθ and y = (r)sinθ

141
Q

Outside Angles Theorem (tangents or secants of circles)

A

1/2 (major arc - minor arc) = angle of the outside angle

142
Q

Distance from a point to a line formula (h, k) and Ax + By + C = 0

A

|Ah + Bk + C| over SqRt A^2 + B^2

143
Q

Interior angles of a regular polygon

144
Q

Exterior angles of a regular polygon

145
Q

Golden ratio

A

Approx 1.618 : 1

146
Q

Angle Bisector of a triangle

A
  • Splits an angle and extends to a side
  • Meeting point is equidistant from the sides
147
Q

Altitude of a Triangle

A
  • Segment perpendicular to a side that goes through opposite vertex
  • All point meet, which might be outside the triangle

CSET Math II (3 decks)

Brainscape helps you reach your goals faster, through stronger study habits.
© 2025 Bold Learning Solutions. Terms and Conditions