Fourier Analysis

Question 1

Give the three goniometric relationships of scalar products of trig functions

Answer 1

Question 2

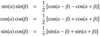
Give the integrals of scalar products of trig functions from -pi to pi

Answer 2

note: δ = 1 if m = n and 0 if not

Question 3

Give the general form of a continuous f(x) over a period 2pi and its coefficients

Answer 3

Question 4

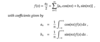
Give the expansion of a continuous function f(x) over a period of 2L and its coefficients

Answer 4

Question 5

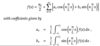
Give the integrals of scalar products of trig functions from -L to L

Answer 5

Question 6

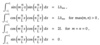
Give Parseval’s identity for a continuous function f(x) over period 2L

Answer 6

Question 7

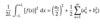
Define an even function f(x)

Answer 7

f(x) is an even function if f(x) = f(-x)

eg cos(x)

Question 8

Define an odd function f(x)

Answer 8

A function is odd if f(x) = -f(-x)

Question 9

Give the equations for a Fourier transform

Answer 9

Question 10

Give Parseval’s identity for Fourier transforms

Answer 10