Derivatives Flashcards
1
Q
Explain the derivative formula. Draw it out.
A

2
Q

A

3
Q
What are the notations for a derivative of a function and a derivative of a point?
A

4
Q
When is a function diferentiable?
A

5
Q
Use basic Derivative rules

A

6
Q
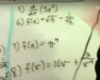
A

7
Q

A
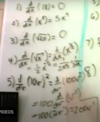
8
Q
Proof of the sum/difference rule of derivatives?
A

9
Q
The power rule?
A

10
Q
Constant Multiple Derivative Rule
A

11
Q
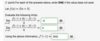
A

12
Q
A

13
Q

A

14
Q

A

15
Q

A
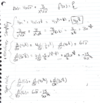
16
Q
What is the derivative of sin(x)
A

17
Q
What is the product rule?
A

18
Q

A

19
Q
What is the quotent rule?
A
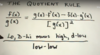
20
Q
What is the derivative of tan(x)
A

21
Q
What are the trig function derivatives?
A

22
Q
Saying for rembering product of a derivative?
A
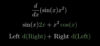
23
Q

A

24
Q

A

25
What is the chain rule?

26


27

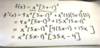
28
Find the derivative of Y with respect to X


29
Find the derivative and explain it using lebienze notation


30


31
Explain this functions using the chain rule and liezben notation


32
33

Second Derivative with implicited diferentiaition

34


35


36
37


38

39
What is the derivatives of the natural log and logbase a?

40

Use logarithimic differentiation. Notice there is a variable in as an exponent and base!

41


42
What are the derivaives rules for exponents and logs and ln

43


44
What are critcal points?

X=0 is a critical point becuase the tangent line goes to 0. at points -3 and 3 they are undefined in the actual function so they cannot be critical points

45
watch out for this
when taking the log of something, make sure you ultiply that entire exponent back onto the log

46
Find the critical point?


47
What is the mean value theorum? how does it look on a graph. What is the formula?


48
49
Describe the extreame value therom

50
What are the absolue max mins and local max mins?


51
Explain concavity using graphs of the first and second derivitives.
the first deriviates shows over the purple bit that the slope is decreasing. the first derivites shows this. Ones the slope of the graph stops decreasing the first derviive become zero because no change is happining and it starts increasing. This is why setting the second deriviative to zero gives us inflections point. the slope of the first dervite become zero.

52
What is the inflection point?
Point on in the function where the slope changes from either increasing or decreasing
53
how can you prove if a function is deacreasing or decreasing?

54
When sketching a function what is everything you should find?

55
What is the first derivative test? And the the increasing/ decreasing test?



