Brainscape's Knowledge GenomeTM

Browse over 1 million classes created by top students, professors, publishers, and experts.


See full index

Statistics > Chapter 9 > Flashcards

Chapter 9 Flashcards

1
Q

What is Hypothesis Testing

A

Hypothesis testing is a methodology to test a theory, claim, or an assertion about a particular parameter of a population.

  • You reject H0 when the sample evidence suggests that it is far more likely that H1 is true.
  • Failure to reject H0 is not proof that it is true. You can never prove that is H0 correct b/c the decision is based on sample information, not the entire population
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
2
Q

Null Hypothesis

A

The null hypothesis (H0)

  • typically represents the status quo
  • stated in terms of the population parameter (e.g. μ, not x̄)
  • uses a sample statistic to make inferences about the population parameter
  • if you reject H0, you have proof that H1 is true
  • if you do not reject H0, it does not mean you’ve proved it.
  • Always contains an equal sign

Proper phrasing of accepting H0: “there is insufficient evidence to reject H0”

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
3
Q

Alternative Hypothesis

A

H1

The alternative hypothesis

  • is the opposite of H0
  • represents the conclusion reached by rejecting H0
  • represents a research claim or specific inference you would like to prove
  • H1 never has an equal sign
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
4
Q

What is Hypothesis Testing

A
  1. Uses a test statistic based on a given sample result
  2. provides clear definitions for evaluating differences
  3. enables you to quantify the decision-making process by computing the probability of getting a certain sample result if the H0 is true
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
5
Q

What is the test statistic

A

Identified by STAT

A statistic computed from a given sample and tested against the sampling distribution for the sample statistic of interest.

If it falls within the Region of Nonrejection, then H0 cannot by rejecte

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
6
Q

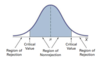
What are the Regions of Rejection and Nonrejection

A

The region of rejection (also “critical region”) consists of values of the test statistic (e.g. ZSTAT) that are unlikely to occur if H0 is true, and thus indicate you should reject H0

The region of nonrejection consists of values of the test statistic that indicate H1 is true and you cannot reject H0.

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
7
Q

What is Type I Error

A

A Type 1 error (TIe) - if you reject H0 when it is true

  • The probability of a TIe occurring is α
  • α is the level of significance of committing a TIe
  • The confidence coefficient, (1 - α), is the probability you will not reject H0 whien it should not be rejected
  • Decreasing α decreases the chance of a TIe, but increases the chance of a TIIe. Use this when you want to be very confident of not making a TIe.
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
8
Q

What is a Type II Error

A

A Type II error (TIIe) - if you don’t reject H0 when it is false

  • The probability of a TIIe occurring is β
  • β is the risk of committing a TIIe
  • The ower of a statistical test, (1 - β), is the probability that you will reject H0 when it should be rejected
  • Decreasing β decreases the chance of a TIIe, but increases the chance of a TIe. Use this when you want to be very confident of not making a TIIe.
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
9
Q

Hypothesis Testing and Decision Making (Review Table)

A
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
10
Q

Describe the Z Test for the Mean (σ Known)

A

The Z Test defines the ZSTAT for determing the difference between the x̄ and the μ

  • Note: it is rare that the σ is known
  • You must assume standard distribution

Z Test Equation:

How well did you know this?
1
Not at all
2
3
4
5
Perfectly
11
Q

Define the Critical Value Approach to Hypothesis Testing

A

Compares the value of the computed ZSTAT test statistic to critical values that divide the normal distribution into regions of rejection and nonrejection

Critical Values are expressed as standardized Z values determined by the level of significance (α)

  • .1: ± 1.645
  • .05: ± 1.96
  • .01: ± 2.575
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
12
Q

Define a two-tail test

A

When H0 contains an ‘=’ sign, the region of rejection is divided into 2 parts.

  • α is ÷’d by 2
  • a rejection region is located below the critical value and above the critical value
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
13
Q

Define the 6 Steps of the Critical Value Approach to Hypothesis Testing

A
  1. State the null hypothesis, H0, and the alternative hypothesis, H1.
  2. Choose the level of significance, α, and the sample size, n. (This is based on the relative importance of the risk of committing TIe and TIIe in the problem)
  3. Determine the appropriate test statistic and sampling distribution
  4. Determine the critical values that divide the rejection and nonrejection regions
  5. :
    • Collect the sample data,
    • organize the results, and
    • compute the value of the test statistic
  6. :
    • Make the statistical decision,
    • determine whether the assumptions are valid, and
    • state the managerial conclusion in the context of the theory, claim, or assertion being tested
      • If the test statistic falls into the nonrejection region, you do not reject H0
      • If the test statistic falls into the rejection region, you reject H0
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
14
Q

p-Value Approach to Hypothesis Testing

A
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
15
Q

What are the decision rules for rejecting H0 in the p-value approach

A
How well did you know this?
1
Not at all
2
3
4
5
Perfectly
16
Q

How do you use the p-value approach for a two-tail test

A

You find the probability that the test statistic ZSTAT is equal to or more extreme than the [something] standard error units from the center of a standardized normal distribution.

i.e.

  • ZSTAT is greater than the critical value of the upper tail
  • ZSTAT is less than the critical value of the lower tail

this means you’re finding hte area under the curve for the two tails, adding them together, and then comparing that number agains α.

17
Q

Define the p-value approach to Hypothesis Testing (5 steps)

A
  1. State H0 and the alternative H1
  2. Choose the level of significance, α, and the sample size, n
  3. Determine the appropriate test statistic and the sampling distribution
  4. Collect the sample data, compute the value of the test statistic, adn compute the p-value
  5. Make the statistical decision and tate the managerial conclusion in the context of the theory, claim, or assertion being tested.
    • If the
18
Q

Define the connection b/t Confidence INterval Estimation and Hypothesis Testing

A

In chapter 8, confidence intervals estimated parameters

In chapter 9, hypothesis testing makes decisions about specified values of population parameters

Equations:

ZSTAT =

19
Q

t Test for the Mean (σ Unknown)

A

Equation defines defines the test statistic for determining the difference between the sample mean,

20
Q

p-value approach (when using tSTAT)

A
21
Q

Ways to evaluate the Normality Assumption

A
  • Examine how closely the sample statistics match the normal distribution’s theoretical properties
  • Histogram
  • Stem and leaf
  • Boxplot
  • Normal probability plot

in order to visualize the distribution of the data

22
Q

The Critical Value Approach for One-Tail Tests

A
  1. Define H0 and H1 (note: H1 contains the statement for which you are tyring to find evidence)
    • H0 ≥ x
    • H1 < x
  2. Collect data and determine α and n
  3. Because σ is unknown, use the t distribution and the t**STAT test staticstic
  4. The rejection region is entirely contained in the lower tail of the sampling distribution of the mean, b/c you only want to reject H0 when the sample mean is significantly < x. This is called a one-tail test
  5. From the sample n you selected, find the tSTAT
  6. Evaluate and determine managerial action
23
Q

7 Key Points about H0 and H1 for One-Tail Tests

A
  1. H0 reporesnts the status quo or the current belief in a situation
  2. H1 is the opporite of H0 and represents a research claim or specific inference you would like to prove
  3. If you reject H0, you have proof that H1 is correct
  4. If you don’t reject H0, you have failed to prove H1. The failure does not mean you have proven H0
  5. H0 always refers to a specified value of the population parameter, not to a sample statistic. (e.g. μ, not x̄)
  6. The statement of H0 always contains an equal sign
  7. The statement of H1 never contains an equal sign
24
Q

Z Test for the Proportion

A

To test a hypotheses about the proportion of events of interest in the population, π, rather than test the population mean, μ.

Compute the sample proportion, p = X/n. Then compare this to the hypothesized value of the parameter π

ZSTAT = (p - π) / sqrt(π(1 - π))/n

Note: confirm that X and (n - X) are each at least 5. This confirms that the sampling distribution of a proportion approximately follows a normal distribution.

25
Q

The Critical Value Approach for Proportions

A
  1. Define H0 and H1
  2. Find the proportion of the sample
  3. Determine (choose) level of significance (α)
  4. Validate that X and n - X are > 5
  5. Find ZSTAT for the proportion
  6. Evaluate and make managerial deccision

Statistics (11 decks)

Brainscape helps you reach your goals faster, through stronger study habits.
© 2024 Bold Learning Solutions. Terms and Conditions