chapter 3- Practical forecasting techniques

Question 1

Thickness of a layer of the atmosphere is

Answer 1

a measure of how warm or cold the layer is; high values mean warm air, and low values mean cold air

Question 2

Thickness can be calculated from

Answer 2

the heights reported on a radiosonde ascent, or a thermodynamic diagram

Question 3

Thickness can be calculated by

Answer 3

subtracting the lower (height) value from the upper value, which always gives a positive number

Question 4

Suppose 500 hPa height is 5407 m and 1000 hPa height is 23 m, what is the thickness of the layer 500‐1000 hPa?

Answer 4

The 500‐1000 hPa layer thickness = 5407 – 23 = 5384 m

Question 5

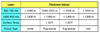
In practical meteorology, the most common layers wherein thickness values are analyzed and forecast are:

Answer 5

500‐1000 hPa;

850‐1000 hPa;

700‐1000 hPa;

700‐850hPa

and 500‐700 hPa

Question 6

The 500‐1000 hPa thickness also known as the

Answer 6

‘total’ thickness

Question 7

The 500‐1000 hPa thickness also known as the ‘total’ thickness, is used to

Answer 7

define the broad average temperature for the lower half of the troposphere.

Question 8

850‐1000 hPa:

Answer 8

This is useful for defining the temperature structure in the lowest 1500 m or so of the atmosphere, and can therefore be used in such things as rain/snow prediction, maximum temperature forecasting etc.

Question 9

700‐1000 hPa:

Answer 9

Similar to 500‐1000 hPa but focused more on the lowest 3 km of the atmosphere, the layers nearer the earth’s surface.

Question 10

500‐700 hPa/700‐850 hPa:

Answer 10

Used in studies of differential thermal advection, particularly when considering possible convection, degrees of instability etc

Question 11

The use of 850‐1000 hPa thickness to forecast the

Answer 11

potential daytime maximum temperature has long been recognized

Question 12

The use of 850‐1000 hPa thickness to forecast the potential daytime maximum temperature has long been recognized. For example

Answer 12

the relationship between 850‐1000 hPa thickness (h*) and the unadjusted maximum temperature (Tu), obtained for regions south of England, is:
Tu =‐192.65 + 0.156h*

Question 13

For example, the relationship between 850‐1000 hPa thickness (h*) and the unadjusted maximum temperature (Tu), obtained for regions south of England, is:
Tu =‐192.65 + 0.156h*
An adjustment is then

Answer 13

added to this figure (Tu), depending upon forecast ‘cloud class’ and the time of the year.

Question 14

The four cloud classes are:

Answer 14

• Class 0: Low and medium cloud generally less than half cover. High cloud not overcast. Fog only around dawn, if at all.
• Class 1: Roughly 50% cloudiness. If fog occurs, it clears slowly during the morning.
• Class 2: Mainly cloudy. If fog occurs, clears by midday, but slowly.
• Class 3: Mainly cloudy. If fog occurs, clears by midday, but slowly.

Question 15

The empirical equation for forecasting maximum temperature (TMax) from 850‐1000 hPa thickness is of the form:

Answer 15

y=a+bx

where

y- Predictand, the variable whose value is to be predicted (e.g., TMax) and

x- Predictor, the variable whose value is used for prediction (e.g., 850‐1000 hPa thickness)

a- intercept

b- slope

Question 16

The slope and intercept are estimated from

Answer 16

the historical data

Question 17

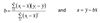
The hypsometric equation tells us that

Answer 17

the thickness of a pressure layer is proportional to the mean temperature of that layer.

Question 18

The hypsometric equation tells us that the thickness of a pressure layer is proportional to the mean temperature of that layer.
Thus,

Answer 18

an empirical relationship can be derived between the thickness of pressure layers and the occurrence of rain, snow, and freezing precipitation

Question 19

Hank’s Rain‐Snow Relationship

Answer 19

It is an empirical rain‐snow relationship based on the 1000‐700 mb thickness

Question 20

Hank’s Rain‐Snow Relationship

It is an empirical rain‐snow relationship based on the 1000‐700 mb thickness.
In this relationship,

Answer 20

a critical thickness value of 2480 m (for 1000‐700 mb layer) has been used to separate rain from snow effectively.

Question 21

That is, if the thickness of 1000‐700 mb layer is:

Answer 21

• Less than 2480 m –mostly snow can be expected
• Greater than 2480 m –mostly rain can be expected
• Equal to 2480 m – 50% snow and 50% rain can be expected

Question 22

That is, if the thickness of 1000‐700 mb layer is:
• Less than 2480 m –mostly snow can be expected
• Greater than 2480 m –mostly rain can be expected
• Equal to 2480 m – 50% snow and 50% rain can be expected
It is to be noted that

Answer 22

we need moisture and lift to produce precipitation. Thus, thickness values alone just provide a clue to the precipitation type, not the occurrence of the precipitation.

Question 23

Rain‐snow Relationship

Answer 23

Question 24

It is to be noted that

Answer 24

there will be some variation of these values with elevation. Essentially, as the elevation increase, the critical thickness value for rain versus snow also increases.

Question 25

Freezing Rain ‐Snow Relationships
Freezing rain and sleet (ice pellets) require

Answer 25

the presence of a warmer than freezing layer above the colder than freezing layer near the earth’s surface.

Question 26

Freezing rain and sleet (ice pellets) require the presence of a warmer than
freezing layer above the colder than freezing layer near the earth’s surface.
Thickness can be used to

Answer 26

approximate this warm‐over‐cold requirement. Specifically, the warm layer can be represented with the 850‐700 mb thickness and the cold layer with the 1000‐850 mb thickness.

Question 27

The table shows the relative values to forecast freezing precipitation.

Answer 27

Thus, the type of precipitation can be determined from the thicknesses of the 850‐700 mb and 1000‐850 mb layers, and the surface temperature.