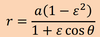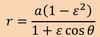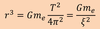chapter 2: Orbits and Navigation Flashcards
The gravitational and astronomical laws were originally formulated to explain
the motion of planets in the solar system and their orbits around the sun.
…………………………were originally formulated to explain
the motion of planets in the solar system and their orbits around the sun.
The gravitational and astronomical laws
These laws (Newtonian and Keplerian) are equally applicable to
the orbits of artificial satellites placed around the earth.
…………………….the orbits of artificial satellites placed around the earth.
These laws (Newtonian and Keplerian) are equally applicable to
Newton’s Law of Universal Gravitation
The force of attraction between two point masses m1 and m2 separated by a distance r is
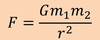
where G is
is theUniversal gravitation constant(6.67259x10‐11 N m2 kg‐2).
the following is
Newton’s Law of Universal Gravitation
The force of attraction between two point masses m1 and m2 separated by a distanceris:
Consider the simple circular orbit shown in Figure. Assuming that the Earth is a ……………………, we can treat it as ………………….
sphere, we can treat it as a point mass
The centripetal force is required to
keep the satellite in a circular orbit is rnv^2/r, here v is the orbital velocity of the satellite.
………………………………….. that balances this centripital force is ……………………..
The force of gravity (F)
Gmem/r2
Theforce of gravity (F) that balances thiscentripetal forceis Gmem/r2
,where me is
and m is
the mass of the Earth (5.97370x1024kg)
the mass of the satellite
Theforce of gravity (F) that balances thiscentripetal forceis Gmem/r2
,whereme is the mass of the Earth (5.97370x1024kg) and mis the mass of the
satellite. Equating the two forces gives:
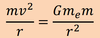
Division bym eliminates the mass of the satellite from the equation, which
means that
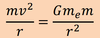
the orbit of a satellite is independent of its mass
Orbital Period (T):
The time taken by a satellite to travel around its orbit once is known as the
period.
The period of an orbit simply depends on
its altitude
The period of the satellite is
the orbit circumference divided by the velocity:
T=2πr/v
orbital period =
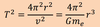
For any given height above the Earth’s surface, a satellite will
take a fixed time
to complete an orbit, regardless of the mass of the satellite.
for …………………………………………… a satellite will take a fixed time to complete an orbit, regardless of the mass of the satellite.
For any given height above the Earth’s surface
Kepler’s laws of motion:
- law of orbits
- law of areas
- law of periods
Kepler’s laws of motion state that:
1.
Law of orbits: All planets travel in elliptical paths with the sun at one focus.
Kepler’s laws of motion state that:
2.
Law of areas: The radius vector from the sun to a planet sweeps out equal areas in equal times
- This empirical law discovered by Kepler arises from conservation of angular momentum.
- When the planet is closer to the sun,it moves faster, sweeping through a longer path in a given time.
Kepler’s laws of motion state that:
3.
Law of Periods: The ratio of the square of the period of revolution of a planet to the cube of its semimajor axis (radius of orbit) is the same for all planets revolving around the sun.
Kepler’s laws of motion
These laws are also applicable to
the artificial satellites placed in elliptical orbits (also called Keplerian orbits) around the earth at one focus.
define perigee
The point where the satellite most closely approaches the Earth
perigee more generally the
perifocus
apogee is also known as
apofocus
apogee or apofocus:
The point where the satellite is furthest from the Earth
semimajor axis is denoted by the symbol
a
semimajor axis:
The distance from the center of the ellipse to the perigee (or apogee)
the eccentricity (E):
The distance (aE) from the center of the ellipse to one focus (to the center of the Earth) divided by the semimajor axis (a)
For an ellipse, the eccentricity is a number between
0 and 1 (0 < E < 1)
A circle is an ellipse with
zero eccentricity
The equation for the elliptical path that the satellite follows, in polar coordinates with the Earth as origin is given as:
the angle O- is
the true anomaly and is always measured counter clockwise (the
direction of satellite motion) from the perigee.
The two basic types of meteorological satellite orbits are:
- Geostationary orbit – high altitude orbit over the equator
- Polar orbit – low altitude orbit from pole to pole
Geostationary orbit:
- A satellite in this orbit will remain stationary relative to the earth’s surface.
- For any orbit to be geostationary, it must be geosynchronous and circular with zero inclination over the equator.
A geosynchronous orbit is
any orbit with an orbital period equal to the earth’s rotational period.
A geosynchronous satellite completes one orbit around the earth in the same
time
that the earth takes to make one rotation
A geosynchronous satellite completes one orbit around the earth in the same time that the earth takes to make one rotation
This time period is known as …………………………. and is equivalent to ………………………….
onesidereal day and is equivalent to 23 h 56 m 04 s
This time period is known as onesidereal dayand is equivalent to 23 h 56 m 04 s .It represents the
time taken by the earth to rotate on its axis relative to the stars, and is almost four minutes shorter than the solar day.
To achieve this earth’sorbital period,the satellite should have
the same angular velocity as the earth.
To achieve this earth’sorbital period,the satellite should have the sameangular
velocityas the earth. Based on the Kepler’s laws, to achieve this, the satellite
must be placed at a height of
35,786 km above the earth
To achieve this earth’sorbital period,the satellite should have the sameangular
velocityas the earth. Based on the Kepler’s laws, to achieve this, the satellite
must be placed at a height of 35,786 km above the earth.
That is, to achieve the earth’s angular velocity of ……………………………………. the satellite altitude can be calculated from
ς = 7.292 x 10-5 s-1
To ensure that a satellite remains over a particular point on the earth’s surface, the orbit must also be
circular and havezero inclination over the equator.
However, a geosynchronous orbit has an inclination of
20 degrees
However, a geosynchronous orbit has an inclination of 20 degrees, because of
which a geosynchronous satellite will move
north and south of the equator during its orbit while the geostationary satellite will not.
Therefore, geostationary satellites are those which orbit with a period
equal to the earth’s rotational period and with zero eccentricity and zero inclination
The theoretical coverage area of a geostationary satellite extends to about
40% of the Earth’s surface.
………………………………..can provide full coverage of the Earth, except for ………………………….
A constellation of three equally spaced geostationary satellites
the polar regions
As satellites in geostationary orbit continuously cover a large portion of the
Earth, this makes it an ideal orbit for
telecommunications or for monitoring continent‐wide weather patterns and environmental conditions
As satellites in geostationary orbit continuously cover a large portion of the
Earth, this makes it an ideal orbit for telecommunications or for monitoring
continent‐wide weather patterns and environmental conditions.
It also ………….. cost as ……………………………………………………………
decreases
as ground stations do not need to track the satellite.
Major disadvantage of this high altitude satellite is, of course,
a low resolution in the images (the typical resolution can be 1 to 4 km).
Some of the operational geostationary satellites are:
GOES (US), METEOSAT (Europe) and INSAT (India)
Polar orbit
The main objective of the satellites rotating in these low‐altitude, high inclination polar‐orbits is ‘global coverage’ with high spatial resolution.
The polar orbiting satellites fly in ………….orbits
much lower orbits typically at around 850 km
The polar orbiting satellites fly in much lower orbits typically at around 850 km, with the orbital plane at
an angle of about 80o to the equator.
the period of these satellites is given by:
Thus, these satellites circle the earth from
pole to pole once in less than 2 hours (T = 104 min) i.e., they circle the earth 14 times each day.
As the earth rotates to the …………….. beneath the satellite, each pass ………………………………………to the ………… of the previous pass
east
monitors an area to the west of the previous pass.
Each polar‐orbiting satellite can typically observe
the entire planet twice in 24‐ hours, once during daylight and once at night
Each polar‐orbiting satellite can typically observe the entire planet twice in 24‐
hours, once during daylight and once at night, with …………………. resolution than the ………………………………….
better resolution than the geostationary satellites.
The orbital plane of these satellites is…………………………, which means ……………………………………….. . hence, this orbit is also known as
fixed relative to the Sun
that the plane of the orbit keeps a constant angle with the sun throughout the year.
sun‐synchronousorbit
The sun‐synchronous orbit ensures that the satellite
passes over a given location on the earth at the same local time each day.
Some of the operational polar orbit satellites are:
TIROS‐N / NOAA‐A (US), METEOR‐2 (Russia), SPOT (France), ERS‐1 (Europe)
Comparison of the characteristics of Geostationary and Polar‐orbiting satellites
To define the position of a point on the celestial sphere (……………………..) we use the …………………………….. known as
an imaginary sphere of infinite radius surrounding the earth
celestial equatorial coordinate system
known as the Right Ascension‐Declination Coordinate System.
Like terrestrial coordinates (i.e., latitude and longitude), the two coordinates to define a point on the celestial sphere are:
the declination and the right ascension.
declination
The coordinate indicating where an object is between the celestial poles
Declinationis measured
from the celestial equator
……………………is measured from the celestial equator
declination
Declinationis measured from the celestial equator
it extends from
0° at the celestial equator to +90° at the north celestial pole and from 0° at celestial equator to‐90° at the south celestial pole.
right ascension
the second coordinate in the celestial equatorial system
- It is analogous to longitude of the terrestrial coordinates.
- Like Greenwich which is the arbitrary zero point for longitude, right ascension also has a zero reference point atVernal Equinox.
- Right ascension, consequently, is measured in (sidereal) hours, 0h to 24h east from the Vernal Equinox Point. That is, east is the direction of increasing right ascension.
equinoxes
The intersections of theecliptic(apparent path of the satellite) with the celestial equator
Consequently, the vernal equinox point:
the autumnal equinox point:
Consequently, the vernal equinox point (RA = 00h 00m 00s) and the autumnal equinox point (RA = 12h 00m 00s).
The position of the satellite at any given time can be correctly identified, if
a set of parameters (orbital elements) fully describe a satellite orbit, are known.
The sixorbital elements, which completely determine the motion of a satellite are:
The semimajor axis and eccentricity define the
size and shape of the ellipse
right ascension of ascending node, inclination angle andargument of perigee combine to
position the ellipse relative to the Earth.
These five parameters define the
shape and location of the orbital path
mean anomaly:
“how far round” the ellipse the satellite lies at any given time
Thus the first two parameters give the
dimensions of the ellipse
the final three define the
position of the orbit relative to the Earth
the mean anomaly indicates
the location of the satellite along the orbital path.
Keplerian orbit
For a satellite in a truly elliptical orbit
For a satellite in a truly elliptical orbit (also known as aKeplerian orbit)allthe
elements
remain constant, with the exception of the mean anomaly which changes with the motion of the satellite, and so increases with time.
Satellite Tracking
Knowing the position of the satellite in orbit
navigation
- In addition to knowing where a satellite is in its orbit, it is necessary to know the Earth coordinates (latitude, longitude) of the particular scene it is viewing and calculating the Earth coordinates is known as thenavigation.
- Navigation– Calculating the location (latitude and longitude) of the spot being sensed by the satellite.
