CH. 9. Concepts and Generic Knowledge

Question 1

Understanding Concepts

Answer 1

UNDERSTANDING CONCEPTS – Ordinary concepts (Ex: concept of what a “restaurant” is or what “money” is) are the building blocks out of which all knowledge is created. You depend on your knowledge in all aspects of day-to-day functioning.

• You need concepts in order to have knowledge, and you need knowledge in order to function.
  
  • In this way, your understanding of ideas like “spoon” and “shoe” might seem commonplace, but it is an ingredient without which cognition cannot proceed.
• EX: Thus, you know what to pay attention to in a restaurant because you understand the basic concept of “restaurant.”
• EX: You’re able to understand a simple story about a child checking her piggy bank because you understand the concepts of “money,” “shopping,” and so on.

LUDWIG WITTGENSTEIN – Argued that the simple terms we all use every day actually don’t have definitions.

• For each clause of the definition, we can find an exception.
  
  • An activity that we call a “game” may NOT share all the relevant characteristics that we associate with “games” and yet, it is a “game” nonetheless, despite not fitting the exact definition.
    
    • The same is true for almost any concept. When we think of “people” or “work” or “floors”, we have a general idea of what that means, but all “people” will not exactly fit any definition of people. Nor will all “work” necessarily fit a definition of “work”. But they do all have things in common with that definition.
• FAMILY RESEMBLANCE – Members of a category have a family resemblance to one another.
  
  • The common features in the family depend on what “subgroup” you’re considering — hair color shared for these family members; eye shape shared by those family members; and so on.
    
    • EX: “Dogs usually are creatures that have fur, four legs, and bark, and a creature without these features is unlikely to be a dog.” This probabilistic phrasing preserves what’s good about definitions — the fact that they do name relevant features, shared by most members of the category.
      
      • But this phrasing also allows a degree of uncertainty.
  • One way to think about this pattern is by imagining the “IDEAL” for each family — someone who has all of the family’s features.
    
    • Each member of the family shares at least some features with this ideal — and therefore has some features in common with other family members.
  • FEATURE OVERLAP – is why the family members resemble one another, and it’s how we manage to recognize these individuals as all belonging to the same family.
• Wittgenstein proposed that ordinary categories like “dog” or “game” or “furniture” work in the same way. There may be no features that are shared by all dogs or all games. Even so, we can identify CHARACTERISTIC FEATURES for each category — features that many (perhaps most) category members have.

Question 2

Prototype Theory

Answer 2

PROTOTYPE THEORY – The best way to identify a category is to specify the “center” (or prototype) of the category, rather than the boundaries.

• EX: Just as we spoke earlier about the “ideal” family member, perhaps the concept of “dog” is represented in the mind by some depiction of the “ideal” dog, and all judgments about dogs are made with reference to this ideal.

IDEAL— The prototype — will be an average of the various category members you’ve encountered.

• Different people, each with their own experiences, will have slightly different prototypes.
• You’ll use this “ideal” as the benchmark for your conceptual knowledge.
• Your reasoning is done with reference to the prototype.

Question 3

Prototypes and Graded Membership

Answer 3

PROTOTYPES AND GRADED MEMBERSHIP – Membership in a category depends on resemblance to the prototype, and resemblance is a matter of degree. (After all, some dogs are likely to resemble the prototype closely, while others will have less in common with this ideal.) As a result, membership in the category isn’t a simple “yes or no” decision.

• GRADED MEMBERSHIP – Objects closer to the prototype are “better” members of the category than objects farther from the prototype.
• SENTENCE VERIFICATION TASK – Participants were presented with a series of sentences, and their job was to indicate (by pressing the appropriate button) whether each sentence was true or false.
  
  • In this procedure, participants’ responses were slower for sentences like “A penguin is a bird” than for sentences like “A robin is a bird”; slower for “An Afghan hound is a dog” than for “A German shepherd is a dog”
    
    • When there was a close similarity between the test case and the prototype, participants could make their decisions quickly – a Robin is closer to an “ideal” bird than a Penguin is, so the association between a robin and “bird” comes faster than an association between a penguin and “bird”.
      
      • In contrast, judgments about items distant from the prototype (penguin) took more time.
      • Penguins and Afghans are more distant from their respective prototypes than are robins and German shepherds.
• PRODUCTION TASK – Simply ask people to name as many birds or dogs as they can.
  
  • According to a prototype view, they’ll do this task by first locating their bird or dog prototype in memory and then asking themselves what resembles this prototype.
    
    • So birds close to the prototype should be mentioned first; birds farther from the prototype, later on.
      
      • The first birds mentioned in the production task should be the birds that yielded fast response times in the verification task; that’s because what matters in both tasks is proximity to the prototype. Likewise, the birds mentioned later in production should have yielded slower response times in verification. This is exactly what happened.
      • This pattern is evidence for prototype theory – Members of a category that are “privileged” on one task (e.g., they yield the fastest response times) turn out also to be privileged on other tasks.
• RATING TASKS – Participants are given instructions like these: “We all know that some birds are ‘birdier’ than others, some dogs are ‘doggier’ than others, and so on. I’m going to present you with a list of birds or of dogs, and I want you to rate each one on the basis of how ‘birdy’ or ‘doggy’ it is”.
  
  • Respondents know exactly what this means and they rate the Robin as “Birdier” (closer to their ideal “bird prototype”) and the penguin as less “birdy” (further from their “bird prototype”).

Question 4

Basic-Level Categories

Answer 4

BASIC-LEVEL CATEGORIES – There is a “natural” level of categorization, neither too specific nor too general, that people tend to use in their conversations and their reasoning.

• EX: Imagine that we show you a picture (of an elaborately upholstered armchair”) and ask, “What is this?” You’re likely to say “a chair” and unlikely to offer a more specific response (“upholstered armchair”) or a more general one (“an item of furniture”).
  
  • EX: Likewise, we might ask, “How do people get to work?” In responding, you’re unlikely to say, “Some people drive Fords; some drive Toyotas.” Instead, your answer is likely to use more general terms, such as “cars,” “trains,” and “buses.”
• If you’re asked to describe an object, you’re likely to use the basic-level term.
  
  • Children learning to talk often acquire basic-level terms earlier than either the more specific subcategories or the more general, more encompassing categories. In these (and other) ways, basic-level categories do seem to reflect a natural way to categorize the objects in our world.

Question 5

Exemplars

Answer 5

EXEMPLARS: – are the “Most Typical” or “Ideal” Examples we have stored in our brain that represent various categories. These exemplars are the prototype.

• All of this fits well with the idea that conceptual knowledge is represented via a prototype and that we categorize by making comparisons to that prototype. It turns out, though, that your knowledge about “birds” and “fruits” and “shoes” and so on also includes another element.
• TYPICALITY – How typical an example is – influences many judgments about category members, including ATTRACTIVENESS. Which of these pictures shows the most attractive-looking fish? Which one shows the least attractive-looking? In several studies, participants’ ratings of attractiveness have been closely related to (other participants’) ratings of typicality — so that people seem to find more-typical category members to be more attractive.
• DIFFERENCE BETWEEN EXEMPLAR and PROTOTYPE –
  
  • PROTOTYPE is an abstract average of the members of a category.
  • EXEMPLAR is an actual member of a category, pulled from memory.
    
    • Prototypes are economical—meaning they are more conducive to quick judgments than exemplars.
• In some cases categorization relies on knowledge about specific category members (i.e. an EXEMPLAR – e.g., “Jerry’s chair”) rather than the PROTOTYPE (i.e. the average of all chairs – e.g., the ideal chair).
• EXEMPLAR-BASED REASONING os reasoning that uses a specific remembered instance (i.e. an EXEMPLAR) – in essence, an example in memory – to compare to in order to come to a conclusion.
  
  • You categorize objects by comparing them to a mentally represented “standard.”
    
    • The difference between the views lies in what that standard is.
    • PROTOTYPE THEORY** – The standard is the prototype — an **average representing the entire category.
    • EXEMPLAR THEORY – The standard is provided by whatever example of the category comes to mind (and different examples may come to mind on different occasions).
      
      • In either case, the process is then the same. You assess the similarity between a candidate object and the standard.
        
        • If the resemblance is great, you judge the candidate as being within the relevant category.
        • If the resemblance is minimal, you seek some alternative categorization.

Question 6

Exemplars and Prototypes in Combination

Answer 6

COMBINATION OF EXEMPLARS AND PROTOTYPES:

• PROTOTYPE** – an **average representing the entire category.
  
  • ADVANTAGE: Prototypes provide an economical representation of what’s typical for a category, and there are many circumstances in which this quick summary is useful.
• EXEMPLAR** – a specific **example of the category that comes to mind (and different examples may come to mind on different occasions).
  
  • ADVANTAGE: But exemplars, for their part, provide information that’s lost from the prototype — including information about the variability within the category.
  • People can adjust their categories in fairly precise ways to match the circumstance.
    
    • EX: not just “gift,” but “gift for a 4-year-old” or “gift for a 4-year-old who recently broke her wrist” or “gift for a 4-year-old who likes sports but recently broke her wrist.”
      
      • This pliability in concepts is easy to understand if people are relying on EXEMPLARS; after all, different settings, or different perspectives, would trigger different memories and so bring different exemplars to mind.
  • ADVANTAGE: knowledge may vary from person to person and from concept to concept. One person might have extensive knowledge about horses, so she has many exemplars in memory; the same person might have only general information (a prototype, perhaps) about snowmobiles.
• Both Exemplars and Prototypes are used in essentially the same way. In either case, an object before your eyes triggers some representation in memory.
• EXEMPLARS are particularly useful if you have:
  
  • Situational Event
  • Vast Knowledge about the type of object (EX: you know a lot about horses)
  • Very Little Knowledge – You formed no prototype but you do have that one example to refer to.

Question 7

Typicality vs. Categorization: DEEP Features

Answer 7

DIFFERENCES BETWEEN TYPICALITY AND CATEGORIZATION – There’s some basis for judging Category Membership that’s separate from the Assessment of Typicality.

• Something can be ATYPICAL and yet still clearly be within a particular category.
  
  • EX: Robins strike us as being closer to (more typical) the typical bird than penguins are; even so, most of us are certain that both robins and penguins are (in the category of) birds.
  • EX: Likewise, Moby Dick was definitely not a typical whale, but he certainly was a whale.
  • EX: Abraham Lincoln wasn’t a typical American, but he was an American.
• How are category judgments made when they don’t rely on typicality?
  
  • EX: Consider a lemon. Paint the lemon with red and white stripes. Is it still a lemon? Most people say that it is. Now, inject the lemon with sugar water, so it has a sweet taste. Then, run over the lemon with a truck, so that it’s flat as a pancake. What have we got at this point?
    
    • Most people still accept this abused fruit as a lemon.
    • We’ve taken steps to make this object more and more distant from the prototype and also very different from any specific lemon you’ve ever encountered – (And therefore very different from any remembered exemplars)
  • But this seems not to shake your faith that the object remains a lemon. Apparently, something can be a lemon with virtually no resemblance to other lemons.

NATURAL vs. MANUFACTURED – People reason differently about naturally occurring items like raccoons and manufactured items like coffee pots.

• Manufactured Items were categorized based on FEATURES, allowing a toaster to be turned into a coffee pot.
• Naturally occurring items were categorized based on DEEP FEATURES – like PARENTAGE.
  
  • EX: Children were asked whether it would be possible to turn a toaster into a coffee pot. They realized there would have to be mechanical changes, but they saw no obstacles to these manipulations and were quite certain that with these adjustments in place, we would have created a bona fide coffeepot.
    
    • Things were different, though, when the children were asked a parallel question — whether one could, with suitable adjustments, turn a skunk into a raccoon. The children understood that we could dye the skunk’s fur, teach it to climb trees, and, in general, teach it to behave in a raccoon-like fashion. Even with these adjustments, the children steadfastly denied that we would have created a raccoon. A skunk that looks, sounds, and acts just like a raccoon might be a very peculiar skunk, but it would be a skunk nonetheless.
      
      • What lies behind all these judgments? If people are asked why the abused lemon still counts as a lemon, they’re likely to mention that it grew on a lemon tree, is genetically a lemon, and is still made up of (mostly) the “right stuff.” It’s these DEEP FEATURES that matter, not the lemon’s current properties. And so, too, for raccoons: In the children’s view, being a raccoon isn’t merely a function of having the relevant features; instead, according to the children, the key to being a raccoon involves (among other things) having a raccoon mommy and a raccoon daddy. In this way, a raccoon, just like a lemon, is defined in ways that refer to deep properties and not to mere appearances.
      • An object’s deep properties depend on a web of other beliefs “tuned” to the category being considered.
        
        • Thus, you’re more likely to think that a creature is a raccoon if you’re told that it has raccoons as parents, but this is true only because you have some ideas about how a creature comes to be a raccoon.
  • It’s this understanding that tells you that PARENTAGE is relevant here.
    
    • EX: Consider as a contrasting case the steps you’d go through in deciding whether Judy really is a doctor. In this case, you’re unlikely to worry about whether Judy has a doctor mommy and a doctor daddy, because your beliefs tell you, of course, that for this category parentage doesn’t matter.
      
      • EX: A counterfeit bill, if skillfully produced, will have a nearly perfect resemblance to the prototype for legitimate money. Despite this resemblance, you understand that a counterfeit bill isn’t in the category of legitimate money, so here, too your categorization doesn’t depend on typicality.
        
        • Instead, your categorization depends on a web of other beliefs, including beliefs about the circumstances of printing a real $20 bill – that it’s legitimate only if it was printed with the approval of, and under the supervision of, the relevant government.
    • You consider circumstances of printing only because your understanding tells you that the circumstances are relevant here, and you won’t consider circumstances of printing in a wide range of other cases.
      
      • EX: If asked, for example, whether a copy of the Lord’s Prayer is “counterfeit,” your beliefs tell you that the Lord’s Prayer is the Lord’s Prayer no matter where (or by whom) it was printed. Instead, what’s crucial for the prayer’s “authenticity” is simply whether the words are the correct words.

Question 8

Resemblance and Similarity

Answer 8

THE COMPLEXITY OF SIMILARITY:

• Judgments about categories are often influenced by typicality.
• Sometimes, though, category judgments are independent of typicality: You judge some candidates to be category members even though they don’t resemble the prototype (think about Moby Dick or the abused lemon).
• You judge some candidates not to be in the category even though they do resemble the prototype (think about counterfeit money or the disguised skunk).
• So how do we think about categories when we’re NOT guided by typicality?
  
  • Answer: We focus on attributes that you believe are essential for each category. Your judgments about what’s essential, however, depend, on your beliefs about that category.
    
    • Therefore, you consider PARENTAGE when thinking about a category (like skunk or raccoon) for which you believe biological inheritance is important. You consider circumstances of printing when you’re concerned with a category (like counterfeit money) that’s shaped by your beliefs about economic systems. And so on.
• RESEMBLANCE – resemblance either to a prototype or to some remembered instance.
  
  • PROTOTYPE and EXEMPLAR views both depend on judgments of RESEMBLANCE.
  • Objects resemble each other if they share properties.
    
    • The more properties shared, the greater the resemblance.
  • HOWEVER, simply sharing various properties won’t work. For RESEMBLANCE to work, the shared properties MUST be IMPORTANT, ESSENTIAL properties.
    
    • On this basis, you regard plums and lawnmowers as different from each other because the features they share are trivial or inconsequential.
    • Your decisions about which features are important depend on your BELIEFS ABOUT THE CONCEPT IN QUESTION.
    • Thus, in judging the resemblance between plums and lawnmowers, you were unimpressed that they share the feature “cost less than a thousand dollars.” That’s because you believe cost is irrelevant for these categories.
• PROTOTYPE use depends on judgments of RESEMBLANCE.
  
  • Judgments of resemblance, in turn, depend on your being able to focus on the features that are essential so that you’re not misled by trivial features.
    
    • Decisions about what’s essential (cost or weight or whatever) vary from category to category and vary in particular according to your beliefs about that category.
• You’re influenced by your background beliefs when considering oddball cases like the mutilated lemon. But you’re also influenced by your beliefs in ordinary cases, including any case in which you’re relying on a judgment of resemblance.
• Whenever you use a prototype or exemplar, you’re relying on a judgment of resemblance.
  
  • ​Resemblance, we’ve argued, depends on other Knowledge (and beliefs).

Question 9

Explanatory Theories

Answer 9

INFERENCES BASED THEORIES – Using our existing knowledge and beliefs, we make inferences that allow us to apply our knowledge to new scenarios and draw broad conclusions from our experience.

• Categorization enables you to apply your general knowledge (e.g., knowledge about dogs) to new cases you encounter (e.g., Milo).
• Categorization enables you to draw broad conclusions from your experience (so that things you learn about Milo can be applied to other dogs you meet).
  
  • All this is possible only because you realize that Milo is a dog; without this simple realization, you wouldn’t be able to use your knowledge in this way.
    
    • People will make inferences from a typical case to the whole category, but not from an atypical case to the category.
• Your inferences are also guided by your broader set of beliefs.
  
  • EX: If told that grass contains a certain chemical, people are willing to believe that cows have the same chemical inside them. This makes perfect sense if people are thinking of the inference in terms of cause and effect, relying on their beliefs about how these concepts are related to each other. (Cows eat grass)

Question 10

Different Concepts Require Different Approaches

Answer 10

DIFFERENT PROFILES FOR DIFFERENT CONCEPTS – People may think about different concepts in different ways.

• EX: Naturally occurring items are seen as stable and consistent across years (plants, animals, mountains) whereas manufactured items (ARTIFACTS – objects made by human beings) can be made or manipulated as we see fit. We can even change manufactured items into other things (a toaster into a coffee pot or a table into a chair), but we cannot change naturally occurring items. a stone is still a stone and a skunk is still a skunk no matter what we do to it.
  
  • People will reason differently about natural kinds and artifacts because they have different beliefs about why categories of either sort are as they are.
  • People tend to assume more stability and more homogeneity when reasoning about natural kinds than when reasoning about artifacts.
  • Many concepts can be characterized in terms of their features (e.g., the features that most dogs have, the features that chairs usually have, and so on; after Markman & Rein, 2013).
  • Other concepts, though, involve Goal-Derived Categories.
• GOAL-DERIVED CATEGORIES – Your understanding of some concepts depend on your understanding of a GOAL.
  
  • EX: Your understanding of concepts like “diet foods” or “exercise equipment” depends on your understanding of the goal (e.g., “losing weight”) and some cause-and-effect beliefs about how a particular food might help you achieve that goal.
• RELATIONAL CATEGORIES (“rivalry,” “hunting”) and EVENT CATEGORIES (“visits,” “dates,” “shopping trips”)
  
  • Here, you’re influenced by a web of beliefs about how various elements (the predator and the prey; the shopper and the store) are related to each other.
• ANOMIA – When people lose the ability to name certain objects or to answer simple questions about these objects.
  
  • Often, the problem is specific to certain categories, such that some patients lose the ability to name living things but not nonliving things.
  • Different brain sites are activated when people are thinking about living things than when thinking about nonliving things.
  • Different sites are activated when people are thinking about manufactured objects such as tools rather than natural objects such as rocks.
  • This is because different types of information are essential for different concepts.
    
    • The recognition of nonliving things may depend on their functional properties.
    • The recognition of living things may depend on perceptual properties (especially visual properties) that allow us to identify horses or trees or other animate objects.
• EMBODIED or GROUNDED COGNITION – The body’s sensory and action systems play an essential role in all our cognitive processes; it’s inevitable, then, that our concepts will include representations of perceptual properties and motor sequences associated with each concept.
• EX: when someone is thinking about the concept “kick,” we can observe activation in brain areas that (in other circumstances) control the movement of the legs; when someone is thinking about rainbows, we can detect activation in brain areas ordinarily involved in color vision.
  
  • Conceptual knowledge is intertwined with knowledge about what particular objects look like (or sound like or feel like) and also with knowledge about how one might interact with the object.
• Conceptual knowledge has many elements. These include:
  
  • PROTOTYPES
  • EXEMPLARS
  • THEORIES
  • and now, REPRESENTATIONS of perceptual properties and actions associated with the concept
    
    • EX: Brain activation in areas that normally control leg movement is a REPRESENTATION of the PERCEPTUAL PROPERTY of “Thinking of Kicking”.
• Your needs at the moment will determine which of these elements you’ll focus on in each circumstance.

Question 11

Knowledge Network

Answer 11

KNOWLEDGE NETWORK:

• LONG-TERM MEMORY is represented by means of a network, with ASSOCIATIVE LINKS connecting NODES to one another.
  
  • Associative Links don’t just tie together the various bits of knowledge; they also help represent the knowledge.
    
    • EX: you know that George Washington was an American president. This simple idea can be represented as an associative link between a node representing Washington and a node representing the president.
      
      • In other words, the link itself is a constituent of the knowledge.
• RETRIEVAL from the KNOWLEDGE NETWORK – Retrieval relies on activation spreading from one node to the next.
  
  • Closely related ideas will require less time to retrieve knowledge due to high priming.
  • “Robin” >> “Bird” should be quick retrieval due to their strong association – as their nodes are likely directly linked.
    
    • But other details are not kept in memory through direct connections – like the fact that robins have hearts and the fact that dogs have hearts and the fact that squirrels have hearts.
    • Instead, it would be more efficient just to store the fact that these various creatures are animals, and then the separate fact that animals have hearts.
      
      • As a result, the property “has a heart” would be associated with the “animals” node rather than the nodes for each individual animal.
      • According to this logic, we should expect relatively slow responses to sentences like “Cats have hearts,” since, to choose a response, a participant must first locate the link from “cat” to “animals” and then a second link from “animals to have hearts”.
        
        • We would expect a quicker response to “Cats have claws,” because here there would be a direct connection between cat and the node representing this property.
      • Even with complications, we can often predict the speed of knowledge access by counting the number of nodes participants must traverse in answering a question.
      • This observation powerfully confirms the claim that associative links play a pivotal role in knowledge representation.

Question 12

Propositional Networks

Answer 12

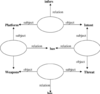
PROPOSITIONAL NETWORKS – uses propositions to determine HOW concepts are related (See image below) – a diagram in which the terms of a proposition and the relations between them are represented as nodes linked to form a network.

• Relationships between objects are represented by symbols and not by mental images of the scene.
• PROPOSITIONS – the smallest units of knowledge that can be either true or false.
  
  • EX: “Children love candy” is a proposition (It is true or false), but “Children” is not.
  • EX: “Susan likes blue cars” is a proposition (It is true or false), but “blue cars” is not.
• To represent your knowledge, we need more than simple associations so that we can determine truth and how concepts are related.
  
  • EX: We need some way to represent the contrast between “Sam has a dog” and “Sam is a dog.”
    
    • If all we had is an association between sam and dog, we wouldn’t be able to tell these two ideas apart – we would not know the truth.
• This model shares many claims with network theories:
  
  • Nodes are connected by associative links.
  • Some links are stronger than others.
  • Strength of a link depends on how frequently and recently it has been used.
    
    • Once a node is activated, the process of spreading activation causes nearby nodes to become activated as well.

Question 13

Distributed Processing

Answer 13

DISTRIBUTING PROCESS: – each idea is represented, not by a certain set of nodes, but instead by a pattern of activation across the network.

• In the model just described (, individual ideas are represented with LOCAL REPRESENTATION. – each node represents one idea so that when that node is activated, you’re thinking about that idea, and when you’re thinking about that idea, that node is activated.
• CONNECTION NETWORKS, in contrast, take a different approach. They rely on DISTRIBUTED REPRESENTATION, in which each idea is represented, not by a certain set of nodes, but instead by a pattern of activation across the network.
  
  • We can only learn what’s being represented by looking at many nodes simultaneously to find out what pattern of activation exists across the entire network.
  • In addition, the steps bringing this about must all occur simultaneously — in parallel — with each other, so that one entire representation can smoothly trigger the next.
• This is why connectionist models are said to involve PARALLEL DISTRIBUTED PROCESSING (PDP) where:
  
  • The brain relies on parallel processing.
  • The brain uses a “divide and conquer” strategy, with complex tasks being broken down into small components, and with separate brain areas working on each component.
  • PDP models have an excellent capacity for detecting patterns
  • As a result, these models are impressively able to generalize what they have “learned” to new, never-seen-before variations on the pattern.
• How PDP models detect patterns and “learn”? :
  
  • ​Recall that in any ASSOCIATIVE NETWORK, knowledge is represented by the associations themselves.
  • EX: What it means to know this fact about Washington as President is to have a pattern of connections among the many nodes that together represent “Washington” and “President”.
• Knowledge refers to a POTENTIAL rather than to a STATE.
  
  • According to this view, “LEARNING” involves adjustments of the connections among nodes, so that after learning, activation will flow in a way that can represent the newly gained knowledge.
    
    • Technically, we would say that learning involves the adjustment of CONNECTION WEIGHTS — the strength of the individual connections among nodes.
      
      • ​Learning requires the adjustment of many connection weights.
      • The connections between nodes have weights with the most heavily weighted being the strongest and fastest connections.
        
        • So, going from ROBIN ⇒ BIRD ⇒ FEATHER will be a faster retrieval process than PENGUIN ⇒ BIRD ⇒ FEATHER.
          
          • Because the connection between ROBIN ⇒ BIRD is heavier than PENGUIN ⇒ BIRD.
  • In this way, LEARNING, just like everything else in the connectionist scheme, is a DISTRIBUTED PROCESS involving thousands of changes across the network.

Question 14

Summary So Far

Answer 14

SUMMARY SO FAR:

• People have a prototype as well as a set of remembered exemplars, and use them for a range of judgments about the relevant category. People also seem to have a set of beliefs about each concept they hold, and these beliefs reflect the person’s understanding of cause-and-effect relationships —
  
  • for example, why drunks act as they do, or how enzymes found in gazelles might be transmitted to lions. These beliefs are woven into the broader network that manages to store all the information in your memory, and that network influences how you categorize items and also how you reason about the objects in your world.
• Apparently, then, even our simplest concepts require a multifaceted representation in our minds, and at least part of this representation (the “theory”) seems reasonably sophisticated. It is all this richness, presumably, that makes human conceptual knowledge extremely powerful and flexible — and so easy to use in a remarkable range of circumstances.
• You don’t have (or need) a definition for most of the concepts in your repertoire; in fact, for many concepts, a definition may not even exist.
• And even when you do know a definition, your use of the concept often relies on other information including a prototype for that term as well as a set of exemplars.
  
  • In addition, your use of conceptual information depends on a broader fabric of knowledge, linking each concept to other things you know.
  • This broader knowledge encompasses what we’ve called your “theory” about that concept — a theory that (among other things) explains why the concept’s attributes are as they are.
  • Whenever you rely on a prototype, you’re drawing conclusions based on the resemblance between the prototype and the new case you’re thinking about, and that resemblance depends on your theory.
    
    • Specifically, it’s your theory that tells you which attributes to pay attention to in judging the resemblance, and which ones to ignore. (So if you’re thinking about computers, for example, your “theory” about computers tells you that the color of the machine’s case is irrelevant. In contrast, if you’re identifying types of birds, your knowledge tells you that color is an important attribute.)
• What does all of this imply for the learning of new concepts?
  
  • Definitions tell you what’s generally true of a concept, but rarely name attributes that are always in place.
  • It’s also important not to be fooled into thinking that knowing a definition is the same as understanding the concept.
  • In addition to the definition you should seek out examples of the new concept.
  • Also want to think about what these examples have in common; that will help you develop a prototype for the category.
• if you’re trying to learn about related concepts or categories?
  
  • ​In these settings, it’s best to hop back and forth with the examples — so that you examine a couple of instances of this concept, then a couple of instances of that one, then back to the first, and so on.
  • This interweaving may slow down learning initially, but it will help you in the long run (leaving you with a sharper and longer-lasting understanding) because you’ll learn both the attributes that are shared within a category and also the attributes that distinguish one category from another.
• In viewing the examples, though, you also want to think about what makes them count as examples — what is it about them that puts them into the category? How are the examples different, and why are they all in the same category despite these differences? Why are other candidates, apparently similar to these examples, not in the category?