Ch 4. Evaluating the Proportional Hazards Assumption

Question 1

General approaches for assessing the PH assumption (3)

Answer 1

• graphical
• goodness-of-fit test
• time-dependent variables

Question 2

Types of graphical techniques to assess PH assumption

Answer 2

To compare:

1. –ln(–ln) Survivor curves are parallel
2. observed vs predicted survivor curse are close

Question 3

Goodness-of-fit (GOF) tests for assessing the PH assumption

Answer 3

• Provides large sample Z or chi-square statistics (which can be computed for each variable in the model, adjusted for the other variables in the model).
• A p-value derived from a standard normal statistic is also given for each variable.

This p-value is used for evaluating the PH assumption for that variable

• p-value large(non-significant) => PH satisfied
• p-value small (e.g. P<0.05) => PH not satisfied

Question 4

Time-dependent variables for assessing the PH assumption for a time-independent variable

Answer 4

Extended Cox model:

Add product term involving some function of time.

Question 5

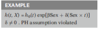
Pros of the 3 approaches for assessing PH assumption

Answer 5

• GOF provides a single test statistic for each variable but it might be too ‘global’ and it may not detect departures from PH (and other 2 can)
• Graphical: subjective
• Time-dependent: computationally cumbersome

Question 6

Graphical Approach to assess PH assumptions 1: Log–Log Plots

Answer 6

A log–log survival curve is simply a transformation of an estimated survival curve that results from taking the natural log of an estimated survival probability twice.:

-ln(-ln ^S)

• ln ^S is negative => -(ln ^S) is positive.
• can’t take log of ln ^S, but can take log of (-ln ^S).
• -ln(-ln ^S) may be positive or negative.