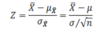Biostatistics (II) Normal & Sampling Distributions Flashcards
(16 cards)
What is the most important distribution in all of statistics?
The normal distribution
What is the normal (Gaussian) distribution equation?
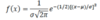
What are the characteristics of a normal distribution?
It is symmetrical around the mean (mu);
mean = median = mode
What is the empirical rule of normal distributions?
(i.e. how much of the data falls between 1, 2, and 3 SD?)
1 standard deviation - 68%
2 standard deviations - 95%
3 standard deviations - 99.7%
What is the following equation used to calculate?
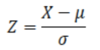
Z = the number of standard deviations above or below the mean that the value (X) lies.
(A Z-transformation of the data)
If the mean (mu) is plugged in for the X value in the following equation, what will be the result, and what does it indicate?
(Z-transformation)
The Z value will be 0;
this means that the selected value is exactly in the middle of the normal distribution, no standard deviations higher or lower
What equation can be used in a normal distribution to test how different (in standard deviations) a particular value is away from the mean?
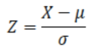
What does a Z value of -1 (using the below equation) indicate about a particular chosen data point?
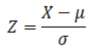
The point (X) is one standard deviation below the mean
To what will the average of a group of sample means in a normal distribution be equal?
The population mean (mu)
(i.e. the average of sample means is equal to the population mean)
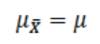
How can the variance of a group of sample means be calculated?
The population variance divided by the square root of the sample size

After using the Z score calculated in the equation below to normalize the data, how can we figure out what percentage of the data falls below a particular value (say, a value found at 2 SD above the mean?)?
(e.g. how much of the data falls below the point 2 standard deviations above the mean in a normal distribution?)
Find
(Hint: no extra calculation needed)
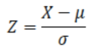
Find the specific Z value on a Z score table.
(in this example, find +2 on the Z score table. The value is 0.9772.
97.72% of the data falls below two standard deviations.)

How can we find the probability that a value picked at random from a normal distribution will be between -2.55 and +2.55 standard deviations?
Normalize the data using the Z score;
find the Z scores on the Z score table
(note: the upper value - the lower value will give you the probability of a data point being between those two points)
(for this example, 0.9946 - 0.0054 = 0.9892 (98.9%))

How can we find the probability that a value picked at random from a normal distribution will be between greater than or equal to a Z of 2.71?
Find a Z Score of 2.71 on the Z Score table;
1 - table value = answer
(1 - 0.9966 = 0.0034 (0.34% of values above 2.71 SD))
What are some of the useful characteristics of creating a sampling distribution from a normally distributed population?
(i.e. taking a sample n of certain characteristics (X-bar, variance, etc.))
The sampling distribution of X-bar is normal.
Mux-bar = Mu
σ2 = σ / SQRT(n)

What are some of the useful characteristics of creating a sampling distribution from a nonnormally distributed population?
(i.e. taking a sample n of certain characteristics (X-bar, variance, etc.))
The sampling distribution is approximately normal
Mux-bar = Mu
σ2 = σ / SQRT(n)
What is the Z-transformation equation for a sampling distribution?
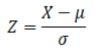
(Hint: the equivalent equation for the Z-transformation equation for normal distributions that is listed below)