AH 2.2.1 Capacitors Flashcards
In the circuit below the capacitor is initially uncharged.
The switch is moved to position 1.
a) what happens to the capacitor?
b) sketch the current v time graph for this situation.
c) sketch the voltage v time graph for this situation
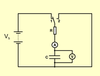
a) The capacitor charges
b) & c) See the graphs below.

In the circuit below the capacitor is initially charged.
The switch is moved to position 2.
a) what happens to the capacitor?
b) sketch the current v time graph for this situation.
c) sketch the voltage v time graph for this situation.
d) Explain the sign of the current in the current discharge graph.
a) The capacitor discharges.
b) & c) See the graphs below which show both the charging and DISCHARGING of the capacitor.

a) Define the time constant τ in an RC circuit (a circuit containing a resistor R in series with a capacitror C).
b) State the equation for the time constant in an RC circuit and sate the unit of τ.
a)
τ = the time for the charge (or voltage) to increase to 63% of its initial value.
= the time for the charge (or voltage) to decay to 37% of its initial value.
b) τ = RC
τ has the unit of seconds
Insert the missing words below.
In an RC circuit, an uncharged capacitor can be considered to be fully charged after a time approximately equal to ………..
In an RC circuit, a fully charged capacitor can be considered to be fully discharged after a time approximately equal to ………….
In an RC circuit, an uncharged capacitor can be considered to be fully charged after a time approximately equal to 5τ.
In an RC circuit, a fully charged capacitor can be considered to be fully discharged after a time approximately equal to 5τ
Where τ = the time constant for the RC circuit
The graphs below show the graphs of current in an RC circuit.
a) In the left-hand graph is the capacitor charging or discharging?
b) In the right -hand graph is the capacitor charging or discharging?
The graphs show the effect of changing the values of resistance R or capacitance C.
c) Copy the graphs and replace the letters D, E, F & G with the either ‘resistor’ or ‘capacitor’

a) Charging
b) Charging
c) See the graphs below.

The circuit below may be used het investigate the way current behaves in a A.C. capacitive circuit.
a) AC current I is directly proportional to three different variables
What are they?
b) State the equation which relates I to these variables

a) I is directly prportional to
- the supply voltage Vs
- the AC supply frequency f
- the capacitance itself C
b) I = 2πfVC
Look at the circuit below which shows a capacitor with an AC supply.
Assuming the capacitance C is contant and the supply Voltage V is constant, sketch a graph to show the variation of current I with frequency f for a capacitive circuit.


a) State the equation for capacitive reactance in terms of voltage and current in an AC circuit.
b) Develop the equation to include frequency
a) Xc = V/I
b) Xc = V/I
= V / 2πfCV
Xc = 1 / 2πfC V cancels
State as many uses of capacitors as you can.
- Timing circuits (e.g. in a pelican crossing)
- High-pass electronic filter (e.g. cross-over network in loudspeaker)
- Flashing neon light circuit.
- Smoothing circuits (smoothing rectified AC)
- Radio receiver circuit
- Camera flash units
- Capitive touch screens
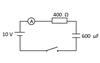
Look at the circuit below. The switch is now closed.
a) Sketch the charging graph of current v time
b) Calculate the current at switch-on and indicate this on your graph
c) Sketch the graph of voltage v time for the capacitor
d) Indicate the maximum voltage across the capacitor on your V-t graph
e) Add to your voltage graph a line indicating the voltage across the resistor
f) Calculate the charge stored on the capacitor.
g) If it takes 0.6 s for the capacitor to fully charge, find the average charging current