(53) Portfolio Risk and Return: Part II Flashcards
LOS 43. a: Describe the implications of combining a risk-free asset with a portfolio of risky assets.
The availability of a risk-free asset allows investors to build portfolios with superior risk-return properties. By combining a risk-free asset with a portfolio of risky assets, the overall risk and returns can be adjusted to appeal to investors with various degrees of risk aversion.

LOS 43. b: Explain the capital allocation line (CAL) and the capital market line (CML).
On a graph of return versus risk, the various combinations of a risky asset and the risk-free asset form the capital allocation line (CAL). In the specific case where the risky asset is the market portfolio, the combination of the risky asset and the risk-free asset form the capital market line (CML).
LOS 43. b: Explain the capital allocation line (CAL) and the capital market line (CML). Compare the CML and the SML.

LOS 43. c: Explain systemic and nonsystemic risk, including why an investor should not expect to receive additional return for bearing nonsystemic risk.
Systemic (market) risk is due to factors, such as GDP growth and interest rate changes, that affect the values of all risky securities. Systemic risk cannot be reduced by diversification. Unsystemic (firm-specific) risk can be reduced by portfolio diversification.
Because one of the assumptions underlying the CAPM is that portfolio diversification to eliminate unsystemic risk is costless, investors cannot increase expected equilibrium portfolio returns by taking on unsystemic risk.

LOS 43. d: Explain return generating models (including the market model) and their uses.
A return generating model is an equation that estimates the expected return of an investment, based on a security’s exposure to one or more macroeconomic, fundamental, or statistical factors.
The simplest return generating model is the market model, which assumes the return on an asset is related to the return on the market portfolio in the following manner:
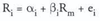
LOS 43. e: Calculate and interpret beta.
Beta can be calculated using the following equation: (See below)
Where [Cov(Ri,Rm)] and pi,m are the covariance and correlation between the asset and the market, and σI and σm are the standard deviations of asset returns and market returns.
The theoretical average beta of stocks in the market is 1. A beta of zero indicates that a security’s return is uncorrelated with the returns of the market.
How the formula is derived:

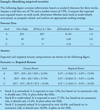
LOS 43. g: Calculate and interpret the expected return of an asset using the CAPM.
The CAPM relates expected return to the market factor (beta) using the following formula:
E(Ri) – Rf = βi[E(Rm) – Rf]

LOS 43. h: Describe and demonstrate applications of the CAPM and SML.
The CAPM and the SML indicate what a security’s equilibrium required rate of return should be based on the security’s exposure to market risk. An analyst can compare his expected rate of return on a security to the required rate of return indicated by the SML to determine whether the security is overvalued, undervalued, or properly valued.
LOS 43. h: Describe and demonstrate applications of the CAPM and SML. Describe the Sharpe ratio and the M-squared measure.
The Sharpe ratio measures excess return per unit of total risk and is useful for comparing portfolios on a risk-adjusted basis. The M-squared measure provides the same portfolio rankings as the Sharpe ratio but is stated in percentage terms:

LOS 43. h: Describe and demonstrate applications of the CAPM and SML. Describe the Treynor measure and the Jensen’s Alpha.
The Treynor measure measures a portfolio’s excess return per unit of systemic risk. Jensen’s alpha is the difference between a portfolio’s return and the return of a portfolio on the SML that has the same beta:



