Work, Energy & Momentum

Question 1

What is the work done by a force F which acts on an object as it moves through a distance d?

Answer 1

W = F*d cos(θ)

Where θ is the angle between the force and the direction of motion.

Since work is proportional to cos(θ), only the component of force parallel to the motion contributes to the work; any forces perpendicular to motion do no work since cos(90)=0.

Question 2

What are the units of work, as defined in physics?

Answer 2

Work has units of Joules (J).

Remember: work is defined as F * d cos(θ), which has units of N * m, or kg * m²/s², which is identical to the units of Joules.

Question 3

What is the sign of work done on an object if it begins at rest and an applied force accelerates in the same direction as the displacement of the object?

Answer 3

The work done on the object by the force is positive (+).

By definition, work done by a force that leads to a change in distance (hence an increase in speed) is positive work if it accelrates in the same direction as the displacement of the object.

If the force has a component in the direction opposite to the displacement, the force does negative work.

Question 4

What is the sign of work done on an object, if it begins at speed v and an applied force decelerates it to rest?

Answer 4

The work done on the object by the force is negative (-).

By definition, work done by a force (such as friction) that leads to a decrease in speed is negative work.

Question 5

What kind of work, positive or negative, can a person do by pushing on a box?

Answer 5

A person can do either positive or negative work by pushing on a box.

If the person pushes on the box in the same direction that it moves, then positive work is done. If the person pushes in the opposite direction of the moving box, then negative work is done.

Question 6

What kind of work (positive, negative, or both) can frictional forces do?

Answer 6

Frictional forces can only do negative work.

By definition, frictional forces are always in the opposite direction of an object’s motion. Hence, they can only slow the object down, and only do negative work.

Question 7

What is the work done by gravity as an object of mass m moves from the ground to a height h?

Answer 7

W = mgh

Remember, W = F * d cos(θ). In this case, as an object moves straight up, θ = 0º and cos(θ) = 1. So, W = F * d. The force of gravity is simply mg, and the distance is h, so the total work done is mgh.

Question 8

An object at rest is moved from the ground to a height h/2, then to rest at a height h. How much work does gravity do during this process?

Answer 8

W = -mgh

The work done by gravity while the object moves to h/2 is -mg(h/2). The work done while the object is moving from h/2 to h is also -mg(h/2). Work is negative in this case, because gravity works against the object being moved.

Notice that this is identical to the work done if the object is moved directly to h; the work done by gravity is path-independent.

Question 9

Define:

mechanical advantage

Answer 9

Mechanical advantage is the multiplication of a force using a mechanical device. A small force exerted over a large distance is transformed into a larger force over a smaller distance.

On the AP exam, mechanical advantage appears primarily in problems including levers.

Question 10

What is the relationship between force and distance in any system which includes mechanical advantage?

Answer 10

F₁d₁ = F₂d₂

In any system exhibiting mechanical advantage, the force exerted and the distance covered are inversely proportional,

Question 11

A force F₁ is exerted a distance d₁ from the fulcrum of a lever. What does the force F₂ at d₂ from the other end equal?

Answer 11

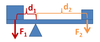
F₂ = F₁d₁/d₂

In any system exhibiting mechanical advantage, the force exerted and the distance are inversely proportional, F₁d₁ = F₂d₂. Rearranging yields the above relationship.

Question 12

A pulley system is set up that allows a weight m to be lifted using a force of only (1/3)mg. How far must the string on which the force is exerted be pulled in order to move the weight a distance d?

Answer 12

The string must be pulled a distance 3d.

In any system exhibiting mechanical advantage, the force exerted and the distance are inversely proportional, F₁d₁ = F₂d₂. So if one-third of the force is required, the distance must increase by the same proportion.

Question 13

Define:

power, as used in physics

Answer 13

Power is a measure of the rate of energy flow.

Power is defined as energy divided by time:

P = E/t

The units of power are Watts, where 1 W = 1 J/s.

Question 14

What is the power flowing through a wire, if 1,000 J of energy flow through in 0.1 s.

Answer 14

P = 10,000 W = 10 kW

Power is energy divided by time;

1000 J / 0.1 s = 10,000 W

Note that kW is a commonly-used unit on the AP exam.

Question 15

Define:

the kinetic energy of an object

Answer 15

An object’s kinetic energy is the energy resulting from its motion.

Kinetic energy is defined as:

KE = ½mv²

where m is the object’s mass and v is its speed. The units of kinetic energy are Joules, just like all other forms of energy.

Question 16

If objects 1 and 2 are moving at the same speed, but object 2 has twice the mass of object 1, how do their kinetic energies compare?

Answer 16

KE₂ = 2*KE₁

Kinetic energy is defined as ½mv², so it is directly proportional to the mass. If the objects’ speeds are the same, kinetic energy will increase proportionally with the mass.

Question 17

If objects 1 and 2 are the same mass, but object 2 is moving at twice the speed of object 1, how do their kinetic energies compare?

Answer 17

KE₂ = 4*KE₁

Kinetic energy is defined as ½mv², so it is directly proportional to the square of the velocity. If the objects’ masses are the same, kinetic energy will increase with the square of the velocity.

Question 18

Define:

the work-energy theorem

Answer 18

The work-energy theorem states that the net work done on an object by all the forces acting on it equals the change in the object’s kinetic energy.

W_net = ΔKE

Question 19

An object is at rest, and a person pushes on it, doing 50 J of work that convert to motion. What is the object’s final kinetic energy? (assume no dissipative forces)

Answer 19

The object’s final KE is 50 J.

According to the work-energy theorem, the net work done on the object results in a change in its kinetic energy. Since the person is exerting the only force on the object which causes motion, the work done equals the change in kinetic energy.

Question 20

An object is moving with 100 J of kinetic energy, and a frictional force acts on it, doing 50 J of work. What is the object’s final kinetic energy?

Answer 20

The object’s final KE is 50 J.

According to the work-energy theorem, the net work done on the object results in a change in its kinetic energy. Since the frictional force is the only force affecting motion, the work done equals the change in kinetic energy. Frictional forces can only do negative work, so the final kinetic energy is lowered.

Question 21

An object with mass m falls through a distance h. What is its final kinetic energy? (assume no dissipative forces)

Answer 21

The object’s final KE is mgh.

According to the work-energy theorem, the net work done on the object results in a change in its kinetic energy. Since gravity is the only force acting on the object, the change in kinetic energy simply equals the work done. Work = F * d, or, in this case, mg * h. You might also recognize this as the gravitational potential energy of the object before it fell.

Question 22

Define:

the gravitational potential energy of an object in a gravitational field

Answer 22

An object’s gravitational potential energy U_gr is defined as the work that was needed to raise it to its present height above an arbitrary reference point.

On the AP exam, the reference point will always be ground level, unless otherwise stated.

Question 23

What is its gravitational potential energy of an object of mass m that is a height h above the surface of the Earth?

Answer 23

U_gr = mgh

This is identical to the work needed to raise the object that height, and is also equal to the kinetic energy the object will gain if it is allowed to fall freely to the ground.

Question 24

How does the gravitational potential energy of a mass change, if its height above the surface of the Earth doubles?

Answer 24

The gravitational potential energy of the mass doubles.

Since gravitational potential energy of an object near Earth’s surface U_gr = mgh is proportional to height above the ground, changing the height changes the energy by the same amount.

Question 25

What is the potential energy of gravitational attraction between two objects of masses m₁ and m₂?

Answer 25

U_gr = -Gm₁m₂/r

G is the universal gravitational constant, and r is the distance between the centers of mass for the two objects.

This expression applies in all scenarios. Hence, is different from U_gr=mgh, which only approximates that near the Earth’s surface, where gravitational acceleration is roughly constant (equal to g, ~10 m/s²).

Question 26

How does the gravitational potential energy between two masses change, if the distance between the two masses doubles?

Answer 26

The gravitational potential energy between the masses increases.

Be careful of signs! The general expression for gravitational potential energy, U_gr = -Gm₁m₂/r, is always negative. Increasing r makes the fraction smaller; in this case, less negative, reflecting an increase in potential energy.

Question 27

What is the force on a mass m, attached to a stretched spring, at a distance x from equilibrium?

Answer 27

F = -kx

Where k is the spring constant (or force constant) of the spring (in N/m) and x is the distance from equilibrium. Notice that the negative sign means force will always act in the opposite direction of net displacement.

Question 28

If spring 1 has twice the value of k that spring 2 has, which spring will need to be moved the greater distance in order to create the same force?

Answer 28

Spring 2 (the lower k) will need to be displaced further.

Since F=-kx and spring 2 has 1/2 the k value of spring 1, spring 2 will need to move twice the distance in order to create the same value of F.

Question 29

Define:

the potential energy of a spring which has been stretched

Answer 29

A spring’s potential energy U_sp is defined as the work needed to stretch or compress the spring from its equilibrium length to the current length.

On the AP exam, the spring’s equilibrium length will be defined as x = 0.

Question 30

What is a spring’s potential energy, if a mass m is attached and the spring is stretched to a distance x from equilibrium?

Answer 30

U_sp = ½kx²

Where x is the distance from equilibrium and k is the spring constant. There is no difference between sign for compression and expansion, since x² will always be positive.

Question 31

How does the potential energy of a spring change, if its extension from equilibrium doubles?

Answer 31

The potential energy of the spring increases by a factor of 4.

Since the potential energy of the spring U_sp = ½kx² is proportional to the square of the displacement of the spring, stretching the spring increases the potential energy by the square of the proportional length change.

Question 32

Define:

the total mechanical energy of a physical system

Answer 32

The total mechanical energy of a physical system is the sum of the kinetic and potential energies of all the objects which make up the system.

E_total = KE_total + PE_total

On the AP exam, you will rarely have to track more than one kinetic and one potential energy in any system.

Question 33

What specific energies constitute the total mechanical energy of a cannonball in flight?

Answer 33

The kinetic energy from the cannonball’s velocity, and the gravitational potential energy due to its height above the ground.

Note: assume the canonball is not rotating, unless explicitly given. Rotational kinetic energy is rarely tested on the AP B exam (though it’s possible on the C exam).

Question 34

What specific energies constitute the total mechanical energy of a mass oscillating on a horizontal spring?

Answer 34

The kinetic energy from the mass’s velocity, and the potential energy due to the extension or compression of the spring from equilibrium.

Question 35

Define:

the principle of conservation of energy

Answer 35

The principle of conservation of energy states that, in the absence of dissipative forces, a system’s total mechanical energy is constant.

Dissipative forces such as friction or air resistance will decrease a system’s total mechanical energy.

Question 36

At the bottom of the initial hill, a roller coaster car is moving with speed v. Ignoring friction, what determines whether it can glide to the top of the next hill?

Answer 36

The car will reach the top of the next hill if its kinetic energy at the bottom K_i is greater than the gravitational potential energy will be at the top of the hill U_f.

Energy conservation states that total energy K+U_gr remains constant. At the bottom of the hill, potential energy is zero. If K_i > U_f, then the initial kinetic energy is sufficient to overcome the force of gravity and climb the hill. If K_i < U_f, the car would not make it to the top of the next hill.

Question 37

Define:

a conservative force in physics

Answer 37

A conservative force is one which does not dissipate mechanical energy.

When only conservative forces are acting on a system, the system’s total mechanical energy will stay constant.

Question 38

What are some common examples of conservative forces in physics?

Answer 38

The most common conservative forces on the AP exam are gravity, the electrostatic and magnetic forces, and the restorative force of a stretched spring.

Question 39

A spring with a mass on each end is floating in space, and one mass is pushed towards the other to compress the spring and then released. How does the total mechanical energy of the compressed spring compare to the oscillating spring?

Answer 39

The total mechanical energy will be the same.

The compressed spring has only potential energy of position, and the oscillating spring can only have as much kinetic energy at equilibrium as was already present. Since no dissipative forces exist, and springs are conservative, total mechanical energy will remain constant.

Question 40

Define:

a nonconservative force in physics

Answer 40

A nonconservative force is one which dissipates mechanical energy.

When nonconservative forces are acting on a system, the system’s total mechanical energy will decrease.

Question 41

What are some common examples of nonconservative forces in physics?

Answer 41

The most common nonconservative forces on the AP exam are friction, air resistance, and fluid viscosity.

Question 42

A box is pushed at some speed across the floor by a force and then released. How does the total mechanical energy of the box at the point of release compare to the box at a later time?

Answer 42

The total mechanical energy later will be less than when released.

The box only has kinetic energy due to velocity, but the dissipative force of friction acts between the box and the floor. Friction inhibits motion, thus kinetic energy, and total mechanical energy, will be less the longer friction acts.

Question 43

Define and give units for:

Force

Answer 43

The net force that an object is experiencing, is its current acceleration times its mass. Force can also be thought of as the change in momentum per unit time, or mass times the change in velocity per unit time.

The SI unit of force is the Newton (N),
1N = 1 kg*m/s²

Question 44

What is the force on an object if it has:

• m = 2kg, a = 10 m/s²?
• m = 4kg, ∆v = 20 m/s, ∆t = 1s?
• ∆p = 10kg*m/s, ∆t = 10 m/s?

Answer 44

• 20 N (F = ma = 2 (10) = 20)
• 80 N (m∆v/∆t = 4 (20/1) = 80)
• 1 N (∆p/∆t = 10 / 10 = 1)

Note: force can be positive or negative, depending on direction of velocity.

Question 45

Define and give units for:

momentum

Answer 45

The momentum, p, of an object is its mass times its current velocity. Momentum can also be thought of as the inertia of an object, or the mass times change in distance per unit time.

The SI unit of momentum is kg*m/s

Question 46

What is the momentum of an object if it has:

• m = 2kg, v = 15 m/s?
• m = 4kg, v = 20 m/s?
• m = 10kg, v = 0 m/s?

Answer 46

• 30 kg*m/s (mv = 2 (15) = 30)
• 80 kg*m/s (mv = 4 (20) = 80)
• 0 kg*m/s (mv = 10 (0) = 0)

Note: momentum can be positive or negative, depending on direction of velocity.

Question 47

Define and give the units for:

impulse

Answer 47

Impulse is the change in momentum of an object, due to a net force applied over some amount in time.

The SI unit of impulse is N*s, or kg*m/s.

Question 48

What is the impulse on an object if it has:

• F_net = 2 N, Δt = 2s?
• Δmv = 8 kg*m/s, F_net = 4 N?
• m = 10, a = 2 m/s², Δt = 2 s?

Answer 48

• 4 kg*m/s (F*Δt = 2 (2) = 4)
• 8 kg*m/s (Δmv = 8 … given)
• 40 kg*m/s (ma*Δt = 10 (2*2) = 40)

Question 49

What corresponding change in momentum will cause an object to suddenly experience half as much force on it?

Answer 49

Momentum per unit time must be halved.

Since force = ma = mΔv/t = Δp/t, momentum and force are directly proportional. Halving force requires momentum to be halved.

Question 50

What is the momentum change for an object which experiences a force that doubles its velocity?

Answer 50

Momentum will be doubled.

Since Δp = mΔv, a change in momentum is directly proportional to a change in velocity. Doubling one will double the other.

Question 51

Describe the difference in impulses if a mass experiences a force for a given time, vs half that force for twice the length of time.

Answer 51

The impulses are equal.

Original impulse = F₁*t₁

Since F₂ = F₁/2 and t₂ = 2t₁,
then: new impulse = F₂*t₂ =
= (F₁/2)*(2t₁) = F₁*t_{1 .}

Question 52

Describe why airbags reduce the force on passengers in a car accident.

Answer 52

Airbags lengthen the amount of time that a crash victim experiences the deceleration forces, meaning the forces are decreased.

From Impulse = F_net*Δt, force and time are inversely proportional. Therefore, if the time increases, the force is decreased.

Question 53

Describe Newton’s first law of motion with regards to force.

Answer 53

In order for an object to have its motion changed there must be an net force acting on it.

If all forces cancel to zero, the object will not experience any change in motion.

Question 54

Describe Newton’s first law of motion with regards to momentum.

Answer 54

An object with directional momentum will continue with that momentum unless acted on by a net force.

Similarly, an object with zero momentum will continue to remain at rest until acted on by a net force.

Question 55

Describe, in terms of force, what will cause an object at rest to gain some velocity?

Answer 55

The object must have experienced a net force.

Since all forces cancel to be zero at rest, there must have been a net force to change velocity.

Question 56

Describe, in terms of momentum, what will cause an object at rest to gain some velocity?

Answer 56

The object must have experienced an impulse (change in momentum) from a net force.

Since all forces cancel to be zero at rest, there must have been a net force to change velocity. If there is a change in velocity, that is directly proportional to a change in momentum.

Question 57

Define:

translation (as it applies to physics)

Answer 57

Translation is the net movement or change in location of an object. This may be thought of as a non-zero displacement in a direction.

Ex: a hockey puck may be said to have experienced translation when it began at one end of an ice rink and was later at the other end.

Question 58

Define:

translational equilibrium

Answer 58

Translational equilibrium occurs when the sum of all of the forces acting on an object cancel to zero. The object will not experience any force causing it to translate.

The trivial case for this is an object at rest, but a object moving with constant velocity is also at translational equilibrium.

Question 59

A skydiver is falling from a plane and has yet to reach terminal velocity. Why is the skydiver NOT in translational equilibrium?

Answer 59

The skydiver is still accelerating (changing velocity), which must be due to a non-zero net force. There is no translational equilibrium.

The downward force due to gravity is greater than the upward force due to air resistance, resulting in a net force on the skydiver.

Question 60

Define:

fulcrum and lever arm

Answer 60

The fulcrum is the point of an object that remains fixed during rotation.

The lever arm is the distance from the fulcrum where a force is applied, to create rotation around the fulcrum.

Question 61

Define and give units for:

torque

Answer 61

Torque is the ability of a force to create a change in the angular orientation of an object, by rotation about a fulcrum point. Torque can also be thought of as the component of work perpendicular to a lever arm.

The SI unit of torque is the N-m.

Question 62

Describe the relationship between the lever arm radius and force applied, which leads to torque.

Answer 62

τ = r*F sinθ

Where r is the distance between force applied and the fulcrum point, F is the magnitude of force applied, and θ is the angle between the lever arm and the force vector.

Only the component of force perpendicular to the lever arm will generate torque, since sin0 = sin180 = 0.

Question 63

How will the torque on a lever arm change, if the same force is applied half the original distance from the fulcrum?

Answer 63

Torque will be halved.

Since torque = r*F sinθ, torque is directly proportional to the distance along the lever arm. Halving the distance that the force is applied at will halve the torque as well.

Question 64

Describe the convention of rotation that is exibited from a positive torque.

Answer 64

Counter-clockwise rotation is positive torque.

The maximum positive (counter-clockwise) torque that a force can deliver is at 90 degrees to the lever arm.

Question 65

Describe the convention of rotation that is exibited from a negative torque.

Answer 65

Clockwise rotation is negative torque.

The maximum negative (clockwise) torque that a force can deliver is at 90 degrees to the lever arm.

Question 66

Define:

rotational equilibrium

Answer 66

Rotational equilibrium occurs when the net torque on an object is zero. If the sum of all torques acting on an object cancel to zero then the object will not undergo rotational acceleration.

The trivial case for this is an object at rest, but a object rotating with constant spin is also in rotational equilibrium.

Question 67

A child on a see-saw leans back, causing her to to lower and her partner to rise. Is the see-saw system in rotational equilibrium?

Answer 67

No, the see-saw is experiencing changing rotation, which must be due to a non-zero net torque, hence there is not rotational equilibrium.

The downward force due to one child creates a torque that is not balanced by the other child, resulting in a net acceleration of the see-saw.

Question 68

A child of mass 40kg sits 1.5m from the pivot of a see-saw. To balance exactly, where on the opposite end should her 30kg little sister sit? (assume the seesaw is massless)

Answer 68

She should sit 2m from the pivot point.

Since all forces due to gravity are perpendicular to the see-saw, torque from the 40kg child will be: (mg)r = 40(10)(1.5) = 600 Nm. To balance, the 30kg child needs to supply 600 Nm of torque, which requires a distance of 2m.

On the AP B exam, torque questions that require calculation always ask for the state of rotational equilibrium (on the AP C exam, further calculations are possible).

Question 69

What visual tool can be used to account for all forces acting on an object?

Answer 69

A free-body diagram.

Free-body diagrams represent force vectors with arrows pointing in the corresponding direction. Larger forces are represented by longer arrows.

Question 70

Describe/draw the free-body diagram for a box with mass m, accelerating towards the earth in free fall.

Answer 70

• A force due to gravity, pointing directly downwards with magnitude mg.
• A force due to air resistance, pointing directly upwards with magnitude kv² (the negative sign is only necessary for calculations).

Note: since the mass is accelerating downwards, the arrow for gravity is represented larger than for air resistance.

Question 71

Describe/draw the free body diagram for the stationary block of mass m on the slope below, providing specific values where possible.

Answer 71

• A force due to gravity pointing directly downwards, with magnitude mg.
• A normal force pointing perpendicular to the slope, with magnitude mg cos(θ).
• A static friction force pointing parallel to the slope, opposing gravity, with a magnitude of mg sin(θ).

Note: the length of the arrows should be proportional to the magnitude of each force.

Question 72

Define:

apparent weightlessness

Answer 72

Apparent weightlessness occurs when there is no force opposing gravity, and the object is in free-fall.

Ex: A passenger in an airplane which is in a free-fall dive, accelerating towards the earth at 9.8 m/s², will experience apparent weightlessness.

Question 73

Define:

real weightlessness

Answer 73

Real weightlessness occurs when the sum of all gravitational forces acting upon an object is zero.

This is an idealized concept that would require an object to be exactly at the center of mass for the entire universe. As soon as that object was in any other location, it could still have apparent weightlessness.

Question 74

A skydiver leaps from a plane, and assuming there is no air resistance, are they experiencing weightlessness?

Answer 74

Yes, apparent weightlessness.

There is no force opposing gravity, and the skydiver is not in contact with any objects that inhibit their inertial movement.

Question 75

Define:

the principle of conversation of linear momentum

Answer 75

The principle of conservation of linear momentum states that in an isolated system the initial momentum on any axis will be the same as the final momentum on that axis.

Note: this principle holds true no matter what type of interaction occurs in the system.

Question 76

Explain why in an isolated box of gas, individual molecules can gain or lose momentum, but the system of gas doesn’t change momentum.

Answer 76

Since this is an isolated system, there are no external forces that act on any molecules within it. With no way to add or remove momentum from the system, momentum must be conserved.

Note: this means that if one molecule gains momentum, another must lose exactly that much momentum.

Question 77

Explain with an example whether it is possible for objects in an isolated system to collide and stop.

Answer 77

It is possible.

Consider two cars of equal weight and equal-but-opposite velocity. Conceptually, this means that the system’s initial momentum is zero, since each car’s momentum is equal and opposite the other’s. If they collide and stick (totally inelastic collision), then the final object’s momentum must be zero - hence, both cars come to rest together.

Question 78

Define:

elastic collision

Answer 78

An elastic collision is one in which the mechanical (kinetic) energy and momentum of the system are both conserved.

Elastic collisions require objects to bounce off each other perfectly, in rigid fashion, without any deformation.

Question 79

Two ideal hockey pucks of equal mass, one traveling to the right and the other to the left, collide in space. Describe the motion of the pucks after collision, assuming they began with equal speed.

Answer 79

The pucks will bounce off of each other in a perfectly elastic collision.

The puck originally traveling left will now go back right, and the puck originally traveling right will now go back left. Their speeds and masses remain constant, but the direction (sign) of velocity and momentum will now be opposite.

Question 80

Define:

inelastic collision

Answer 80

An inelastic collision involves some mechanical (kinetic) energy of the system being converted to internal energy, as the objects collide and separate. Momentum will remain conserved.

An inelastic collision results from friction causing heat, or compression causing potential energy or work on an object. At least one object will be deformed, heated, broken, or otherwise changed.

Question 81

Two cars of equal mass, one traveling to the right and the other to the left, collide and deform in space. Describe the motion of the cars after collision, assuming they began with equal speed and are not stuck together afterwards.

Answer 81

The cars will bounce off of each other in an inelastic collision.

The cars will lose kinetic energy due to the collision. Their speeds will decrease and masses may change if one car loses mass to the other. The direction (sign) of velocity and momentum will now be opposite, but total momentum remains conserved.

Question 82

Define:

totally inelastic collision

Answer 82

A totally inelastic collision involves some mechanical (kinetic) energy of the system being converted to internal energy, as the objects stick and remain connected. Momentum will remain conserved.

An inelastic collision results in at least one object being deformed, heated, broken, or otherwise changed.

Question 83

Two soft clay balls of equal mass, one traveling to the right and the other to the left, collide and stick in space. Describe the motion of the balls after collision, assuming they began with equal speed.

Answer 83

The balls will stick together and stop, in a totally inelastic collision.

The balls will lose kinetic energy due to the collision. Their speeds will decrease to zero and masses combine together. The velocity will now be zero, but total momentum remains conserved (it began as zero).

Question 84

Two balls of equal mass collide in space. Knowing nothing else, what can be said about this system after collision?

What if the collision was elastic vs. totally inelastic?

Answer 84

Momentum will be conserved, no matter what collision type.

If the collision is elastic, kinetic energy will also be conserved.
If the collision is totally inelastic, the system will have one final object with double the mass.

Question 85

What is the equation relating momentum before and after a totally inelastic collision, for two masses traveling at some velocities?

Answer 85

m₁v₁ + m₂v₂ = (m₁+m₂)v_f

This is simply derived from the usual conservation of momentum, keeping in mind that after a totally inelastic collision the objects combine into a single mass.

Question 86

What is the equation relating kinetic energies before and after a totally inelastic collision, for two masses traveling at some velocities?

Answer 86

½m₁v₁² + ½m₂v₂² > ½(m₁+m₂)v_f²

This is simply derived from the usual decrease in kinetic energy in any inelastic collision, keeping in mind that after a totally inelastic collision the objects combine into a single mass.