Topic 6: Import Tariffs in HOS

Question 1

What extra assumptions are made to add imports to the HOS model?

Answer 1

1. Home hosuehodl does not reckognise any relationship between its consumption of imports and tariff revenu to the government.
2. The tarriff is p_X = p_X* + t, p_Y = p_Y*
3. Tarrif revenue is returned by the government to the collective hosuehold i a lump sum R.

Question 2

What is GDP at factor cost, GDP and GNP in the HOS model with a tarrif?

Answer 2

GDP at factor cost = M = wL+rK

GDP=GNP= M + R

Question 3

How is the consumer problem then solved in the HOS with import tarrif model?

Answer 3

From Max U = C_X^1/2C_Y^1/2

Subject to p_XC_X +p_YC_Y = M + R

Through the lagrange method, we will get

C_X = (M+ R)/2p_X, C_Y = (M+R)/2p_Y

But we don’t know R. But we can formulate it as

R = t(C_X - X). We then get.

R = t((M+R)/2p_X - X) = (M-2p_XX)t / (2p_X - t)

Question 4

How can the equlibrium in the HOS model with a tarrif be graphed?

Answer 4

The free trade equilibrium has production at A and consumption at B.

The tarrif raises p_X from p_X* to p_X*+t

Production is then determined at C. The line tangent is the GDP at factor costs, M.

Adding tarrif revenues yeilds consumer income, and so they can purchase on a budget constraint is shifted right from the GDP at factor costs line.

Question 5

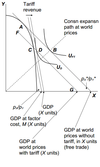
Give the equations for different measures of GDP in the HOS + tarrif model.

Answer 5

GDP_FC = M = wL + rK = P_XX+P_YY

GDP_Income = M + R = p_XX + p_YY + t(C_X - X)

GDP_Expenditure = C + p_XC_X + P_YC_Y

Question 6

Under what condition will the BOP be equal to zero in the HOS + tarrif model?

Answer 6

When the collective household satisfies its budget constraint. When the constraint is satisfied, there is no need to impose the BOP condition, it is implied.

Question 7

Prove that the BOP = 0 in the HOS + tarrif model.

Answer 7

GDP_Income = GDP_Expenditure

p_XC_X + p_YC_Y = p_XX + p_YY + t(C_X - X)

p_XC_X- p_XX + p_YC_Y - p_YY - t(C_X - X) = 0

p_X(C_X - X) + p_Y(C_Y-Y) - t(C_X - X) = 0

(p_X - t)(C_X - X) + p_Y(C_Y-Y) = 0

As p_X = p_X* + t, p_X* = p_X - t, and p_Y* = p_Y

Then p_X*(C_X-X) + p_Y*(C_Y-Y)=0 = BOP.