Topic 3.3: Dipolar relaxation

Question 1

• Use a diagram to describe the transitions between 2 spins coupled by dipolar coupling/ spin-lattice relaxation

Answer 1

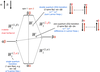
Question 2

• How would the relaxation transition be different if spins were only j coupled?

Answer 2

• Would be no transitions as j coupling does not depend on orientation

Question 3

• Describe the change of populations for all levels of 2 dipolar coupled spins

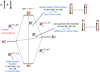
Answer 3

Question 4

• Change in populations must be given in terms of magnetisation, what are the mechanisms of dissipation of magnetisation during spin transitions?

Answer 4

• Auto/spin-lattice relaxation: dissipation of energy to surroundings; magnetisation goes away (unrecoverable)
• Cross relaxation: magnetisation disappears via switching from spin 1 to spin 2 as relaxation is about losing population/energy.
• Transfers polarisation magnetisation to another spin

Question 5

• How are transition probabilities related to spectral densities? How do spectral density profiles differ depending on transition type for 2 same nuclear spins

Answer 5

• Transition probabilities directly related to spectral densities sampled at the frequency of the transition

Question 6

• How does auto-relaxation and cross-relaxation relate to one another in a system of 2 same spins

Answer 6

• Both can contribute to magnetisation and both depend on correlation time

Question 7

• What must be considered first in a homonuclear dipolar relaxation experiment (auto)?

Answer 7

• Starting point/conditions of experiment
• R_auto represents R₁ for a proton in only specific case where proton is inverted with a constant saturation of the coupled spin i.e. inversion of both spins at same time
• Usually would do a non-selective inversion recovery where both coupled protons inverted (bottom)
• Function largest fq at 0, get larger rate, shorter T₁, faster recycling of experiment (top)

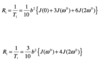
Question 8

• What must be done to observe a change of intensity for spin 1 due to Nuclear Overhauser effect, NOE (cross relaxation)?

Answer 8

• Perturb populations of spin 2 and observe how magnetisation/signal of spin 1 changes
• Transient NOE – invert spin 2 with a 180^o population inversion
• Steady state NOE – saturate spin 2 by equalizing populations via long weak selective irradiation of spin 2

Question 9

How is cross relaxation affected overall by its mechanism?

Answer 9

• When DQ = ZQ cant use NOE (medium molecules)

Question 10

Transient NOE

• If want to observe a change on spin 1 do cross relaxation for NOE. Must perturb populations on spin 2
• For transient NOE do by inverting populations of spin 2 (180 pulse)
• Populations = number of spins
• Single quantum Transitions are selectively irradiated (inverted) for spin 2 (1)
• Resulting spin 2’s inverted in single quantum transitions (2) (3)

Answer 10

• Left side shows result of above, where spin 1 still at eqbm, spin 2 inverted
• For spin 2 to go back to equilibrium must move more towards situation seen in spin 1 as represents unperturbed equilibrium populations
• Can be done via DQ (2 spins up then down) or ZQ (when 1 flips up the other flips down)
• DQ (W₂): many spin 1 in lower energy state (5) compared to higher energy state (1)
• Spin 2 currently has 3 in each
• DQ transition done (shaded spin 1 and spin 2 moved down)
• How is spin 1 affected by this overall perturbation of spin 2? Must look at transitions involving flipping of spin 1 (α↔β) i.e. single quantum (W₁) transition population differences
• Values annotated have increased population differences, giving larger signal for spin 1 due to NOE when DQ transitions involved. Positive NOE attained
• ZQ (W₀): at equilibrium equal populations for spin 1, unequal populations for spin 2
• Spin flipped, spin 1 and spin 2 added to other side to balance?
• Differences now smaller in SQ transitions
• NOE using ZQ transition decreases population for spin 1 due to inversion of spin 2, giving smaller signal and negative NOE

Question 11

• Steady-state NOE:
• To see changes to intensity on spin 1 must also perturb spin 2
• Instead of inverting, spin 2 in saturated
• Meaning populations equalised on spin 2 when transition irradiated
• Single quantum Transitions are selectively irradiated (saturated) for spin 2 (3)
• Resulting spin 2’s saturated in single quantum transitions (4) (5) balancing out spin 2’s across transitions

Answer 11

• Once again, for spin 2 to go back to equilibrium must move more towards situation seen in spin 1 as represents unperturbed equilibrium populations
• DQ: at eqbm there is much more spin in low energy α spin 1 state (5) than high energy β (1)
• For spin 2 to match this must take a spin 2 but also spin 1 (as DQ transition) from high to low energy
• Intensity of spin 1 due to this change in population can be seen by looking at SQ transitions (W₁)
• As before, overall difference is larger, leading to larger signal than equilibrium leading to positive NOE
• ZQ: spin 1 has equal populations at eqbm so to match with spin 2 using a zero-quantum transition, must transfer a spin 2 and a spin 1 (as flip flop transition) from αβ to βα
• As in transient, overall difference smaller, leading to smaller signal than equilibrium leading to negative NOE