The Horopter Flashcards
1
Q
Define Corresponding points
A
- Pair of points, one in each eye that, when stimulated simultaneously or rapidly in succession, are perceived to lie in identical directions
- To understand how we perceive visual space as a whole, we need to understand how groups of corresponding points are processed
2
Q
Define Horopter
A
- Horopter - Spatial map of corresponding points across the retina (that yield single vision)
- Horopter means “horizon of vision”
3
Q
Define Theoretical Horopter and what are its characteristics?
A
- Theoretical point horopter - locus of all points that are imaged on corresponding points of each eye when the eyes converge to aim at a particular fixation point
- An object located on the horopter has the same visual direction in each eye with its image falls on corresponding retinal points
- Corresponding retinal points have zero disparity (since they have same oculocentric visual direction)
- Horopter is a 3-D structure
- Theoretical horopter extends both horizontally and vertically from the fixation point
- However, the vertical axis of horopter is less understood
4
Q
What is a point horopter?
A
- 3-D horopter with zero disparity points
5
Q
What causes disparity?
A
- It caused by the horizontal displacement of the two eyes, the horopter is considered as an arc in horizontal plane (useful in explaining, fusion and stereopsis in binocular vision)
- The horopter falls on a circle that includes the fixation point & the nodal points of the 2 eyes
6
Q
When the eyes are fixating at a distant object, the circle (horopter) is ____
A
large
7
Q
When the eyes are fixating a nearer object, the circle (horopter) is _____
A
smaller
8
Q
The shape of the horopter was studied by who?
A
- Veith in 1818 and Muller in 1840
- The theoretical circle (horopter) with the geometry is known as Vieth-Muller horopter or Vieth-Muller circle
- Because it predicts the shape of the horopter solely by geometry or angular arrangment of corresponding points in each eye
9
Q
Theoretically, for symmetric fixation in the midline, the horopter exist only in the …?
A
- horizontal plane and in a vertical line that passes through the fixation point
- All other points will stimulate disparate retinal locations
- With asymmetric fixation, the horopter becomes twisted into a complex curve
10
Q
How do you measure the horopter?
A
- Our goal is to understand the basic principles of binocular fusion
- For this purpose, it is sufficient to limit the horizontal plane
- The horizontal horopter is usually measured by aligning vertical rods, such as those in the howard Dolman apparatus (real stereopsis assessment)
- Because it uses vertical rods to measure the horopter, the horizontal horopter is also called longitudinal horopter
- To determine if the horopter actually coincides with this theoretical vieth muller cicle, we must empirically measure the horopter on human subject
- If the measured horopter-lie on the VM cicle, then our corresponding points are precisely evenly spaced relative to the fovea
- If the measured horopter-falls off the VM cicle, then our corresponding points could not be arranged in such an orderly manner
11
Q
Define Empirical horopter & what are the different techniques to measure empircal horopter?
A
- An actual measured horopter is referred to as an empirical horopter
- Can be measured in a lab
- Techniques to measure the empirical horopter
- Apparent fronto-parallel plane (AFPP) technique
- Diplopia Threshold Technique
- Stereo acuity Horopter
- Nonius Horopter
12
Q
What is the Apparent Fronto-Parallel Plane (AFPP) Technique
A
- Hering used this simple technique to measure empirical horopters
- In this technique, the subject is aked to maintain steady fixation on a central fixation point
- Then aligns a number of stimuli on either side of the fixation point so that they are all in a plane parallel to the subject’s face plane
13
Q
What is diplopia threshold technique?
A
- Since the true horopter should be at the center of Panum’s space, another way to measure the empirical horopter is
- to find the limits of panum’s space, then compute its center
- In this, the subject fixates a center rod, and the peripheral rods are moved in or out until diplopia is observed
- This is repeated several times
- The near and far diplopia thresholds are plotted to delineate Panum’s space and the midpoint is plotted at the horopter
- The problem with this technique is that it is difficult for subjects to judge when they first see diplopia, especially in the periphery, where panum’s space becomes large
14
Q
What is Stereo Acuity Horopter?
A
- Another way to measure the horopter is to measure the proximal and distal limits of the zone of zero stereopsis
- A difficult visual task
15
Q
Nonius Horopter
A
- This technique is the most accurate method for measuring empirical horopter
- Named after Nunez, a portugese mathematician who did research using a vernier scale in the 1500s
- Tschermak, in 1900, was the first person to use this binocular vernier technique to measure the horopter
- The Nonius apparatus is similar to the Howard-Dolman device, except that the top half of the lines are seen by one eye, and the lower half are seen by the other eye
- While fixating the center rod, the subject must aling the top & bottom halves of each peripheral rod
- Vernier acuity is extremely precise, so this is an ideal way to measure the empirical horopter
- The upper & lower rods are all seen monocularly, not binocularly, so rods are never fused
- The rods will only appear to be aligned when they both have the same oculocentric visual direction, which is how the theoretical horopter is defined
- The nonius horopter is therefore considered the purest & most direct method for measuring the true horopter
- In all of these techniques, horopters are usually not measured peripherally behyond about 15 degrees of eccentricity
- Even at 12 degrees, the AFPP and diplopia techniques are very difficult to use, but the nonius alignment is still possible
16
Q
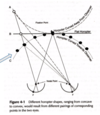
Do the Empirical and Theoretical Horopter Agree?
A
- Figure shows examples of an empirically measure horopter (AFPP method) compared to the Vieth-Muller circle for different fixation distances
-
In theory, the horopter should be a circle (Vieth -Muller Circle)
- Larger circle at distance
- At infinity = flat line
- The empirical horopter departs from the vieth-muller circle in several ways as show in figure
- Note that the rods (dots) are not located on the Vieth-Muller circle
- The departure from the Vieth-Muller circle is different from the different fixation distances
- The shape of empirical horopter changes for different fixation distance and is not always a circle
- Short fixation distances the arc is concave towards the observer
- At some distances, known as the abathic distance, the AFPP horopter becomes flat. The abathic distance maybe 1 to 6 meters, depending on the individual.
- Beyond the abathic distance the AFPP horopter becomes convex
-
Vertical horopter
- Should theoretically be parallel to the head
- However, when measured empirically, the vertical horopters tilts away from the observer
-
Horizontal Surfaces
- Appear to be tilted upward (for our perception) .
17
Q
Describe Hering-Hillebrand Deviation
A
- The difference between the measured horopter and the theoretical horopterfor that test distance is known as the Hering-Hillebrand deviation
- The nonius technique gives slightly different results from the AFPP method, but it still usually does not match the Vieth-Muller circle, though they are closer than with the AFPP or diplopia
- This may be due to irregularities in the distribution of visual directions in the two eyes, or to optical distortion in the retinal image.
- These were not taken into account in the Vieth-Muller circle.
18
Q
The Vieth-Muller circle assumes what?
A
-
The Vieth-Muller circle assumes that
- Both retinas are spherical (circular)
- Both retinas have symmetric distributions of local signs across nasal temporal retinas
- The distributions of local signs are also symmetric on nasal and temporal hemi-retinas in both
19
Q
What accounts for Hering-Hillebrand Deviation? (4 types)
A
Spherical Retinas
- Eyes are assumed to be roun d
- this is a close first-order approximation for most normal eyes, but it may not hold for everyone, especially for myopes
- Their eyes may be slightly elongated, and this could distort the horopter
- But the Hering-Hillebrand deviation is seen even with emmetropic eyes
Retinal Asymmetry
- One explanation for the Hering-Hillebrand deviation is the asymmetric distribution of oculocentric visual directions (local signs) in the nasal and temporal hemi-retinas
- Recall that in constructing the horopter, the visual direction associated with the nasal retina in one eye is matched to the visual direction of the temporal direction of the other eye
- Studies show that the photoreceptors are more densely packed in the nasla than temporal retina
- This nasal-temporal asymmetry could cause the horopter to depart from the Vieth-Muller circle
- This can also explain why the horopter’s form can change from concave to flat, then to convex with different viewing distances
Optical Distortion
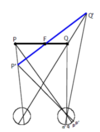
- Optical distortion may contribute to the Hering-Hillebrand deviation, especially if the optical magnification between the two eyes is different
- If the image to one eye is magnified, the AFPP horopter will tilt around the fixation point, as shown in figure
- The true endpoints of the fronto-parallel lane are indicated by points P and Q
- Assuming no image magnification, the retinal image of these points would be points P and Q on the 2 retinas
- A magnified right image is represented by points P’ and Q’
- Tracing these out of the eye and finding the intersection with the corresponding left eye visual lines, we can determine the perceived location of the fronto-parallel lane
- The plane appears to be farther away from the eye with the greater horizontal magnification
-
During Empirical Horopter Measurement
- To compensate a subject will move those rods closer, in an attempt to move them into the apparent frontoparallel plane
- So if the empirical horopter is tilted closer to one side, it indicates greater retinal magnification in the eye that is closer to the horopter
Fixation disparity
- A fixation disparity can also cause the empirical horopter to depart from the theoretical horopter
- In fixation disparity, the visual axes of the 2 eyes fail to perfectly converge on the fixation point, since they are still slightly under or over converged with respect to the fixation point they still have a residual disparity
20
Q
How would the horopter appear in intermittent exotropes?
A
- May exhibit horopter that is excessively curved
- When the pt is fusing, the horopter may actually lie within the Vieth Muller circle
- It may be possible that abnormal horopter may be the cause of their strabismus, rather than the strabismus resulting in horopter abnormality
21
Q
How would the horopter appear in constant strabismic subjects?
A
- In constant strabismic subjects, the horopter will be shifted toward the intersection of the two visual axes
-
Additional abnormalities in the horopters may be present in esotropic subejcts
- Horopters of such subjects do not follow the smooth curve of typical normal horopter
- There is a large “notch” in the horopter near the fixation point called the FLOM NOTCH
22
Q
Describe the flom notch
A
- Lies within the region between visual axes of the 2 eyes
- Suggestive of a regional spatial distortion under binocular conditions, may be a result of anomalous correspondence
- As the objective angle of strabismus is reduced to align the eyes, the horopter notch disappears
24
Q
Describe prisms
A
- Prisms can cause nonuniform magnification distortions of visual space with more magnification at the apex than at the base
- With base-in prisms the horopter bows out away from you (visual space curves towards the viewer)
- With base-out prisms the horopter curves towards you (visual space curves away from the viewer
25
Q
Describe Aniseikonia
A
- Aniseikonia is a difference in (magnification) perceived image size or shape between the two eyes
- Affects 2-3% of the population
26
Q
What are the different types of aniseikonia?
A
- Optical Aniseikonia: Caused by the difference in retinal image between the 2 eyes and may be caused by due to axial anisometropia (axial aniseikonia) or refractive anisometropia (refractive aniseikonia)
- Induced Aniseikonia: form of optical aniseikonia specifically caused by external factors such as an afocal magnifier-called size lens (thick lens)
- Neural or essential aniseikonia: small magnitude non optical aniseikonia that can occur even in emmetropes, in which two retinal images are equal in size yet still perceived to be different in size
33
Q
Describe the geometric effect
A
- The geometric effect occurs due to magnification of the retinal image in the horizontal plane only
- A true fronto-parallel plane appears tilted because the magnification causes horizontal disparities
- Figure is a rough illustration showing that horizontal magnification over the right eye causes an apparent rotation of a fronto-parallel plane away from the eye
- The effect is called “geometric” because the perceived orientation of the plane can be predicted from the geometry of the disparities caused by magnification on one side
34
Q
Describe the leaf room
A
- The tilt and distortion of the visual world induced by uniocular magnification is made more apparent in the leaf room
- leaf room is a cube with walls, floor and ceiling covered with elaves to help obscure monocular cues of depth
- The entire room looks tilted and distorted when a magnifier is palce before one eye (geometric effect)
35
Q
What is the Brecher Maddox Rod & Space Eikonometer used for?
A
- Magnification differences between the 2 eyes can also be detected with Brecher Maddox rod technique
- We can demonstrate tilted percepts secondary to magnification effects with a leaf room or space eikonometer
36
Q
What is the induced effect?
A
- The induced effect is caused by magnification in the vertical meridian only, and it causes an opposite rotation to that predicted for the geometric effect
- Vertical mag over the right eye makes a fronto-parallel plane appear rotated toward the right eye
- The exact reason for the induced effect is unknown
- Vertical mag causes a differential vertical disparity in the 2 eyes, but the vertical disparities do not contribute to a stereoscopic depth perception
- Somehow processing within the visual system causes the vertical disparities to modify actual retinal horizontal disparities
- One explanation is that the vertical mag of the retinal images stimulates compensatory mechanism in the visual system that, in effect, reduces the overall perceived size to match the vertical size in the other eye’s retina
- The compensation, however, shrinks both the vertical and horizontal dimensions
- The net result is that the horizontal dimension of the image becomes relatively smaller than the corresponding dimensions in the eye that had no magnifying lens. This summarized in figure
39
Q
An overall magnification (equal horizontal and vertical mag) before one eye will cause _______
A
- both geometric and induced effects
- Since these effects are opposite, they tend to cancel each other out, and the net result may be less perceived aniseikonia than would be expected from the compute magnification
- Overall power differences between the 2 eyes are often less problematic than differences in oblique magnification
41
Q
Describe uniform mag (less than 4%) and Mag greater than 5-7%
A
-
Uniform magnification (less than 4%): produces both geometric and induced effects
- which will cancel out resulting in little or no effect on the orientation in the frontoparallel plane
- Magnification greater than 5-7% breaks down the induced effect leaving an uncorrected geometric effect in high anisometropic pt with its accompanying rotation of visual space
