Test of hypothesis and confidence intervals Flashcards
(16 cards)
Size
Pr of type I error, rejecting a true model.
Power
Pr of rejecting a false model under the alternative hypothesis
Beta
Pr of type II error, accepting the null under the alternative
Preferable critical regions (simple hypothesis)
One critical region of size alpha is preferable to other region of size alpha, it if has a greater power under the alternative
MP test
A critical region of size alpha is most powerful if it is preferable to any other critical region of size alpha
Neyman pearson lemma

Uniformly most powerful test

Unbiased test
power within H0 is smaller that the power in H1
Consistent test
lim as n goes to infinite of the power function (under the alternative) is 1
Asymptotic distribution of likelihood ratio
-2ln(lambda) is chi square with r degrees of freedom
Monote likelihood ratio property

Theorem of monotone likelihood ratio property

Generalized LR test

two sided LR test for a normal pop with known variance

Two sided for the mean for normal pop with unknown variance
The transformation is decreasing in the LR then I reject iff statistic above critival value
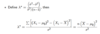
Bayesian posterior for normal pop and prior



