Test 2 Flashcards
(21 cards)
1
Q
Chain rule
A

2
Q
Derivative of Power functions:
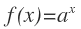
A
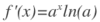
3
Q
Derivative of:

A

4
Q
Derivative of:

A
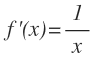
5
Q
Derivative of:
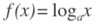
A
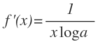
6
Q
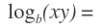
A

7
Q

A
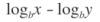
8
Q
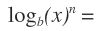
A
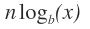
9
Q
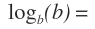
A

10
Q
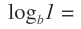
A

11
Q
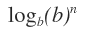
A

12
Q
Exponential Growth and Decay
A

13
Q
Compound Interest
A
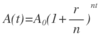
14
Q
Continously compounded interest
A

15
Q
Critical numbers
A
a number c in the domain of f, such that either f’(c)=0 or f’(c)= DNE
16
Q
Closed interval method (finding abs max/min)
A
- Find the values of f at the critical numbers of f in interval
- find the values of f at the endpoints of the interval
- largest value= abs max, lowest value= abs min
17
Q
Mean Value Theorem
A

18
Q
First Derivative Test (for finding local max/mins)
A
- if f’(c) goes from (+) to (-) at c = local max
- if f’(c) goes from (-) to (+) at c = local min
- if no change= no local min/max
19
Q
Concavity Test
A
- if f ¨(x)>0 in I, then = CU on I
- if f ¨(x)<0 in I, then = CD on I
20
Q
Inflection points
A
- if concavity changes at point P, then = POI
21
Q
Second Derivative Test
A
- if f’(x)=0, and f ¨(x)>0, then f has a local min at c
- if f’(x)=0, and f ¨(x)<0, then f has a local max at c


