Rate in chemical rxn

Question 1

Rate Law Summary

Answer 1

Rate law - rate (speed) of reaction (in moles / litre / second) can be expressed as function of concentration of REACTANTS (not products!).

Rate law equation in chemical reaction:

rate = k[A]^m[B]ⁿ

[] = concentration of corresponding reactant in m/L

k = rate constant

m = order of reaction with respect to A

n = order of reaction with respect to B

m + n = overall rate of reaction order

Reaction rate dependent ONLY on concentration of reactants not products!

• Note: Rate constant k is reaction specific, directly proportional to rate of reaction. It increases with increasing temperature since proportion of molecules with energies greater than activation energy E_a of reaction increases with higher temperatures.

Question 2

Rate Orders

Answer 2

• Zero rate order for reactant A (m = 0) - rate of reaction INDEPENDENT OF CONCENTRATION of reactant A (or B) = constant reaction rate. Rate equation expressed as rate constant k. Rate depends on temperature or other factors excluding concentration.

Rate = k

• First rate order for reactant A (m = 1) - rate of reaction DIRECTLY PROPORTIONAL TO CONCENTRATION of reactant A (or B), rate equation expressed as:

Rate = k[A]¹ OR

Rate = k[B]¹

• Second rate order for reactant A (m = 2) - rate PROPORTIONAL TO SQUARE OF REACTANT CONCENTRATION, rate equation expressed as:

Rate = k[A]² OR

Rate = k[B]²

Question 3

Rate Orders:

Concentration vs Time Diagram

Answer 3

Figure:

• For zero-order reactant - as concentration of reactant A decreases over time, slope of line is constant = rate is constant. Rate doesn’t change regardless of decrease in reactant A concentration over time = zero order rate order.
• For first order reactant - decrease in reactant A concentration affect rate of reaction in direct proportion = concentration decreases = rate decreases proportionally (slope↓)
• For second order reactant - rate of reaction decrease proportionally to square of reactant A concentration (slope↓)

NOTE! Curves for 1^st & 2^nd order reactions resemble EXPONENTIAL DECAY. 2^nd order reactions decay faster.

Question 4

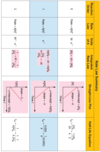
Rate Order: 0

Answer 4

Straight line Diagram

Reaction Order: 0

Rate Law: Rate = k[A]₀

Integrated Rate Law: [A]_t = -kt + [A]₀

Units of k: M⋅s^-1

Question 5

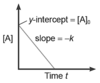
Rate Order: 1

Answer 5

Straight line Diagram

Reaction Order: 1

Rate Law: Rate = k[A]¹

Integrated Rate Law: In[A]_t = -kt + In[A]₀ or ln[A]_t/[A]₀ = -kt

Units of k: s^-1

Question 6

Rate Order: 2

Answer 6

Straight line Diagram

Reaction Order: 2

Rate Law: Rate = k[A]²

Integrated Rate Law: 1/[A]_t = kt + 1/[A]₀

Units of k: M^-1 ⋅ s^-1

Question 7

Rate Law Summary Diagrams

Answer 7

Question 8

Half-Life of Reaction

Answer 8

Half-life of reaction – time needed to decrease concentration of reactant to one-half of original starting concentration.

Each rate order has its own respective half-life.

Half life for first order is constant.

Half life for second order is increasing.

Question 9

Half-Life 1st Order

Answer 9

First order: length of half-life is constant

Half-Life Equation: t_1/2 = 0.693/k = 1/k(0.693)

Question 10

Half-Life 2nd Order

Answer 10

First order: length of half-life is increasing

Half-Life Equation: t_1/2 = 1/k[A]₀ = 1/k ·1/[A]₀

Question 11

Determining Rate Order From Experiment

Answer 11

First 3 experiments - [A] changes but [B] remains same, resultant changes in rate only depend on concentration of A, when [A] doubles (Exp. 1 & 2) reaction rate doubles, and when [A] triples (Exp. 1 & 3) reaction rate triples = directly proportional, exponent of [A] must be 1 & rate of reaction is first order with respect to A.

Final 3 experiments - [B] changes while [A] remains same, when [B] doubles (Exp. 3 & 4) rate increases by factor of 4 (= 24); when [B] triples (Exp. 3 & 5) rate increases by factor of 9 (= 54) = relation is exponential where exponent of [B] is 2 – rate of reaction is second order with respect to B.

• initial rate = k[A]¹ [B]²
• Overall rate of reaction (n+m = 1+2 =3) is 3^rd order.

Question 12

Rate-Determining Step

Answer 12

Rate of overall reaction is naturally limited by slowest step = rate-determining step in mechanism of reaction is slowest step - overall rate law of reaction = rate law of slowest step.

Faster processes have indirect influence on rate à regulate concentrations of reactants & products.

• Chemical equation of each elementary step reflects exact molecular process that transforms its reactants into its products - its rate law can be predicted from its chemical equation - in elementary process, orders with respect to reactants are equal to corresponding stoichiometric coefficients.

If non-elementary reaction (several steps) with slowest step – rate overall = rate of slowest step (if visible within written reaction)

If elementary reactions (single step) – rate = stoichiometry.

Reactants and products have opposite signs but same constant rate.

Question 13

Dependence of Reaction Rates upon Temperature

Answer 13

Many reactions slow down by ↓T & get faster by ↑T

From collision theory of chemical kinetics: rate constant of reaction: k = A·e^(-E_a/RT)

Rate constant Arrhenius equation describes relationship between rate constant (k) & temperature:

• A = Arrhenius constant (frequency factor), includes 2 components: orientation factor (p) & collision frequency (z). Collision frequency (z) – # of collisions that molecules acquire per unit time, orientation factor (p) – proper orientation reactant molecules require for product formation. Arrhenius constant related to both frequency of collisions (z) & proper orientation (p) of molecular collisions required for final product formation: A = pz
• e = base of natural logarithms
• E_a = activation energy – energy required to get reaction started

For reactants to transform into products, reactants must go through high energy state or “transition state” = minimum energy (activation energy) required for reactants to transform into products. If 2 molecules of reactants collide with proper orientation & sufficient energy or force in such way that molecules acquire total energy content surpassing activation energy, E_a, collisions result in complete chemical reaction & formation of products. Note! Only fraction of colliding reactant molecules will have sufficient kinetic energy to exceed activation energy barrier.

• R = ideal gas constant (1.99 cal mol^-1 K^-1)
• T = absolute temperature, NEVER NEGATIVE!

Shown in equation k = A·e^(-E_a/RT) that rate constant, k, contains T component as exponent - T affects reaction rate by affecting actual rate constant k.

• Note: Rate constant remains constant only when T remains constant.
• Either ↑T or ↓E_a will result in ↑constant k = ↑reaction rate. Because -E_a/RT is negative exponent, so biggest value when negative exponent is small, so ↑T or ↓E_a makes exponent smaller.

Question 14

Arrhenius Equation

Answer 14

Question 15

Exothermic Reaction

Answer 15

• Total energy of reactants (A+B) is higher than total energy of products (C+D) = exothermic reaction.
• ΔH = negative = exothermic

Question 16

Endothermic Reaction

Answer 16

• Total energy of reactants (A+B) is lower than total energy of products (C+D) = endothermic reaction.
• ΔH = positive = endothermic

Question 17

Difference between PE

& activated complex

Answer 17

• Difference in potential energy between reactant(s) & activated complex = activation energy of forward reaction.
• Difference between product(s) & activated complex = activation energy of reverse reaction.
• Note! The bigger the difference between total energy of reactants & activated complex = activation energy E_a the slower the reaction.

Question 18

Catalyst

Answer 18

Catalyst - increases rate of chemical reaction without being consumed by reaction.

Catalysts help ONLY by lowering E_a of reaction & help reaction to proceed = ↑Rate_initial.

Enzymes as typical biological catalysts – protein molecules with very large molar masses containing one or more active sites. Enzymes = very specialised catalysts – generally specific & operate only on certain biological reactants called SUBSTRATES.

Enzymes increase rate of reactions by large factors.

REMEMBER: ​Equilibrium K not affected by catalyst! Catalyst has no effect on K, ΔH, ΔE, ΔG or Q

Question 19

Reaction with or without catalyst

Answer 19

Potential energy diagram:

showing both exothermic without catalyst & exothermic with a catalyst - forward reaction being endothermic, thus reverse reaction is exothermic.

Question 20

Saturation Kinetics

Answer 20

Saturation kinetics diagram:

• When concentration of substrate large enough for substrate to occupy all available active sites on enzyme, any further increase would have no effect on rate of reaction = saturation kinetics

Question 21

Equilibrium constant K_eq

Answer 21

Equilibrium constant K_eq - relative concentrations of all components of forward & reverse reactions become constant at equilibrium = state of “dynamic equilibrium

aA + bB ⇌ cC + dD

K_eq = [C]^c[D]^d / [A]^a[B]^b

K value = indication of where equilibrium point of reaction actually lies, either far to right or far to left or somewhere in between.

1. If K_eq > 1 – forward reaction is favoured = reaction favours product formation. If K is very large, equilibrium mixture will then contain very little reactant compared to product.
2. If K_eq < 1 – reverse reaction is favoured = reaction doesn’t proceed very far towards product formation and thus very little product formed.
3. If K_eq = 1 – neither forward nor reverse directions are favoured.

• Note! Pure solids & pure liquids don’t appear in equilibrium constant - heterogeneous equilibria, since liquid & solid phases are not sensitive to pressure, their “concentrations” remain constant throughout reaction = their values denoted as 1.

Question 22

Law of Mass Action

Answer 22

LAW OF MASS ACTION:

Equilibrium constant K (or K_eq) has given value at given temperature:

If T changes value of K changes.

At given T, if we change concentration of A, B, C or D, system evolves in such way to re-establish value of K.

For chemical reaction mixture that is in equilibrium, ratio between concentration of reactants & products is constant.

With chemical equilibria there is constant interplay between molecules but, at given temperature, equilibrium constant Keq remains constant.

Question 23

Le Chatelier’s Principle

Answer 23

Le Chatelier’s principle -

whenever perturbation/stress applied to system at equilibrium, system evolves to compensate for applied perturbation = relieve the stress.

Question 24

Le Chatelier’s Principle Diagram

Answer 24