Quanta and Waves part I

Question 1

Under which condition will a wave undergo a phase change of λ/2 upon reflection?

Answer 1

The wave will be travelling from a medium with a lower refractve index and reflected by a medium with a higher refractive index.

Question 2

A stationary wave pattern is shown below.

What is the wavelength of the wave?

Answer 2

The wavelength is 1m.

(There are three half-wavelengths in 1.5m)

Question 3

Shown below is an example of a ‘black body radiation curve’ which shows the irradiance of all wavelengths of radiation emitted by an object.

What is the relationship between the ‘peak wavelength’ emitted by an object and its temperature in kelvin?

Answer 3

The shorter the ‘peak wavelength’ of the object, the higher the temperature of the object.

Quantatively, this relationship is known as ‘Wein’s law’ and is given by the relationship

T x λ_p = 2.9 x 10^-3

Question 4

There exists some well known experimental evidence for wave-particle duality.

Can you name some experimental evidence which shows the

1. Particle nature of waves
2. Wave nature of particles.

Answer 4

1. The photoelectric effect
2. Electron diffraction patterns

Question 5

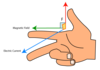
Describe the motion of a charged particle which enters a magnetic field:

1. Perpendicular to the field
2. Parallel to the field
3. At an angle

Answer 5

1. The particle will travel with circular motion only.
2. The particle will continue with linear motion parallel to the field only.
3. The particle will travel with helical motion (circular perpendicular to the field and linear parallel to the field).

Question 6

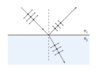
Shown below is an example of interference by division of amplitude- a lens is ‘bloomed’ with a non-reflective coating.

Derive the expression d = λ/4n where ‘d’ is the thickness of coating required to give destructive interference.

Answer 6

Optical PD = λ/2 for destructive interference

also from the diagram, Optical PD = 2nd

therefore

2nd = λ/2

d = λ/4n

Question 7

The relationship describing a travelling wave is shown below.

y = 4.0 sin2π(8t – 5x)

What is the relationship describing a reflected wave of the same type with half the amplitude, travelling in the opposite direction?

Answer 7

y = 2.0 sin2π(8t + 5x)

Question 8

What is the relationship between Brewster’s angle and the refractive index of a material?

Answer 8

n = tan i_p

Question 9

What is the condition for destructive interference in terms of optical path difference?

Answer 9

Constructive interference occurs when

Optical path difference = (m+1/2) λ

Question 10

Describe the effects of damping in simple harmonic motion. Consider:

1. Underdamping
2. Overdamping
3. Critical damping

Answer 10

1. The effects of underdamping are quite small and result in a slow reduction in amplitude.
2. The damping is so great that no complete oscillations are seen. (sometimes called heavy damping)
3. This means that the oscillator comes to rest in the minimum possible time

Question 11

Describe how aurorae are produced in the earth’s upper atmosphere

Answer 11

Cosmic rays enter the earth’s magnetic field and spiral with helical motion along the field lines until they enter the earth’s atmosphere at the north and south poles.

Collisions with atoms in the earth’s atmosphere produce energy in the form of light, known as aurora. (Borealis, Northern lights. Australis, Southern lights)

Question 12

Nils Bohr and Louis de Broglie suggested that electrons can be considered as ‘standing waves’ in orbit around the nucleus of an atom.

Using the circumference of an orbit (2πr) being equal to n de Broglie wavelengths (nλ), derive an expression for the quantised angular momentum of an electron.

Answer 12

2πr = nλ

2πr = nh/p (since λ = h/p)

2πr = nh/mv (since p = mv)

mvr = nh/2π

Question 13

What does it mean if a wave is said to be plane polarised?

Answer 13

The electric field vector (of the light) oscillates in a single plane.

(NOT ‘direction’)

Question 14

The phase difference (Φ) between two points on a travelling wave separated by a distance of half a wavelength (x = λ/2) is equal to how many radians?

Answer 14

Φ = x/λ x 2π

so if x = λ/2

Φ = π radians.

Question 15

Starting with the relationships

y = A sin ωt and v = Aω cos ωt

show that the velocity of a particle undergoing simple harmonic motion can be expressed as

Answer 15

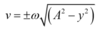
• y = A sin ωt and v = Aω cos ωt

rearranging gives

• sin ωt = y/A and cos ωt = v/Aω

since sin²ωt + cos²ωt = 1

• y²/A² + v²/A²ω² = 1

multiplying through by A²ω²

• y²ω² + v² = A²ω²

​now rearrange for v²

• v² = A²ω² - y²ω²

^​take ω² out as a common factor

• v^{2 =} ω²(A² - y²)

square root both sides

• v = ±ω√(A² - y²)

Question 16

What is the unit of phase angle, Φ?

Answer 16

Radians (rad).

Question 17

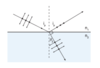
What effect would having a thinner ‘wedge’ (d) have on the spacing between bright fringes (∆x) in the following experiment?

Answer 17

A thinner wedge would make the spacing further apart.

Question 18

What is the condition for destructive interference in terms of phase?

Answer 18

Two waves meet completely out of phase by half a wavelength- crest meets trough.

The phase difference Φ = π radians.

Question 19

The relationship shown below can be used to determine the wavelength of a laser going through a double slit.

What effect would be seen on the interference pattern if a smaller wavelength of light was used?

Answer 19

The distance between adjacent bright fringes, Δx, would be smaller (the fringes would be closer together).

Question 20

The relationship shown below can be used to determine the wavelength of a laser going through a double slit.

Define each of the quantities in the relationship.

Answer 20

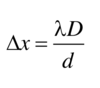
Δx = distance between adjacent bright fringes (m)

λ = Wavelength of the laser light (m)

D = distance from double slit to screen (m)

d = distance between slits (m)

Question 21

Considering the relationship shown for velocity in SHM, describe the conditions when the velocity is at a maximum and at a minimum.

Answer 21

Velocity is at a MAXIMUM when y = 0 (This simplifies the equation to v_max=ωA).

Velocity is at a MINIMUM (zero) when y = A or -A.

Question 22

Derive an expression for the kinetic energy of an object undergoing simple harmonic motion.

Answer 22

Question 23

What is the condition for constructive interference in terms of optical path difference?

Answer 23

Constructive interference occurs when

Optical path difference = mλ

Question 24

Explain the production of ‘cosmic air showers’ when cosmic rays enter the atmosphere.

Answer 24

The cosmic ray strikes a nucleus creating secondary particles (usually hadrons such as the pion) which in turn collide with other particles and so on creating a ‘shower’ of particles which are detectable a ground level (such as muons, positrons and neutrinos).

‘Cosmic air showers’ provided the very first evidence for sub nuclear particles and the quark model of matter.

Question 25

Energy can be measured in either joules or electron-volts.

How do you convert between Joules and electron-volts (eV)?

Answer 25

An electron volt is defined as the energy gained by an electron when accelerated through 1V of potential difference:

W = qV

= 1.6 x 10^-19 x 1

= 1.6 x 10^-19J

so 1 eV = 1.6 x 10^-19J

Question 26

Plane polarised light can be produced by partial reflection from a glass surface as shown below.

The reflected ray will be plane polarised if the angle of incidence is equal to Brewster’s angle (i_p).

What condition must be met to find Brewster’s angle, and produce plane polarised light?

Answer 26

The angle between the reflected and refracted rays must be 90 degrees as shown below. This will produce a reflected ray which is plane polarised.

Question 27

An experiment designed to show ‘thin wedge’ interference is set up as shown below:

What is the relationship used to describe thin wedge interference, and what does each quantity represent?

Answer 27

∆x = distance between bright fringes (m)

λ = wavelength of light (m)

l = length of ‘wedge’ (m)

d = height of ‘wedge’ at end (m)

Question 28

Stationary waves can only be formed by interference at very specific frequencies.

What name is given to these frequencies?

Answer 28

Resonant frequencies.

Question 29

Interference can be produced by two methods, division of wavefront and division of amplitude.

Give example(s) of an experiment demonstrating

1. Interference by division of wavefront
2. Interference by division of amplitude

Answer 29

1. Young’s double slit experiment, experiments using gratings.
2. ‘Bloomed’ lenses, thin film interference, ‘wedge’ fringes, newton’s rings.

Question 30

Simple harmonic motion can be defined mathematically in terms of force and acceleration.

What two relationships can be used to describe simple harmonic motion in the y direction?

Answer 30

F = - ky

and

a = -ω² y (where ω = 2πf)

Question 31

Assuming no energy losses, describe how the type of energy an object undergoing simple harmonic motion has changes over time.

Answer 31

The energy of the object is constantly being converted from kinetic energy to potential energy and back again.

Kinetic energy is at a maximum when velocity is at a maximum (at y=0).

Potential energy is at a maximum when velocity is zero (when y = A or -A)

At any point:

total energy = potential energy + kinetic energy.

Question 32

Assuming the relationship

y = A cos ωt

derive expressions for velocity and acceleration for an object undergoing simple harmonic motion.

Answer 32

y = A cos ωt

differentiating this gives

v = -Aω sin ωt

differentating this again gives

a = -Aω² cos ωt

= -ω²y

Question 33

State what is meant by ‘cosmic rays’.

Answer 33

Cosmic rays are high energy particles entering the atmosphere from space.

They consist mainly of protons, electrons and alpha partciles from the sun, known as the solar wind.

Question 34

Assuming the relationship

y = A sin ωt

derive expressions for velocity and acceleration for an object undergoing simple harmonic motion.

Answer 34

y = A sin ωt

differentiating this gives

v = Aω cos ωt

differentating this again gives

a = -Aω² sin ωt

= -ω²y

Question 35

How can the Bohr model of the atom account for line emission spectra?

Answer 35

Electrons orbit the nucleus of an atom in only certain allowed orbits.

Changes between orbits can only produce discrete, fixed quanta of energy, given by ΔE = hf.

This produces discrete frequencies (and wavelengths) of light.

Question 36

The displacement of an object undergoing simple harmonic motion can be described by the relationships

y = A sin ωt

and

y = A cos ωt

(depending on initial conditions.)

Sketch a displacement time graph for each of these situations.

Answer 36

Question 37

What is the unit of magnetic induction (magnetic field strength) (B)?

Answer 37

Tesla (T).

Question 38

Quantum Physics describes the behaviour of materials/atoms/molecules at very small (atomic) scales. What is meant by the word ‘quantised’?

Answer 38

Properties (such as energy) only exist in well defined discrete states, rather than in a continuous range.

Question 39

In simple harmonic motion, what is the relationship between angular frequency and the period of oscillation?

Answer 39

ω = 2πf and f = 1/T

so

ω=2π/T.

Question 40

The magnitude of the force on a charged particle entering a magnetic field can be determined using the relationship

F = qvB

where v is the particle’s velocity perpendicular to the magnetic field, B.

How should you deal with particles that do not enter fields perpendicularly?

Answer 40

You should calculate the component of the particle’s velocity perpendicular to the field.

In this example, v would become vsinθ

Question 41

What is the condition for constructive interference in terms of phase?

Answer 41

Two waves meet completely in phase- crest meets crest.

The phase difference Φ = 0 radians.

Question 42

What is the relationship between the optical path difference and the phase difference (Ф) of two coherent waves of wavelength λ?

Answer 42

phase difference Ф (in radians) = (2π/λ) x optical path difference

Question 43

Classical theory suggested that as an object becomes hotter, the irradiance of short wavelength radiation would increase dramatically. In fact, experiements show this irradiance is very small.

What is this effect known as, and why does it happen?.

Answer 43

• This is known as the ‘ultraviolet catastrophe’.
• The reason it happens is because individual photons have their energy quantised by the relationship E=hf.
• Due to energy conservation, there are far less high energy photons allowed than at other wavelengths.

Question 44

On a stationary wave, what is meant by the term node?

Answer 44

A point on the stationary wave pattern where there is no disturbance/zero displacement.

Question 45

Explain, in terms of superposition of waves, how a stationary wave is produced.

Answer 45

Stationary waves are formed by the interference of two waves, of the same frequency and amplitude, travelling in opposite directions.

Sometimes this is due to the original wave being reflected - the transmitted wave and the reflected wave undergo interference.

Question 46

By referring to the uncertainty principle relating energy and time, explain the concept of ‘quantum tunneling’.

Answer 46

A particle can exist in a position that, according to classical physics, it has insufficient energy to occupy

or

the lifetime of a particle may be sufficiently short for the uncertainty in its energy to give a realistic probability of the particle having sufficient energy to escape from the potential well (overcome an energy barrier)

(this links to the uncertainty principle: delta E x delta t ≥ h/4π )

Question 47

What is the relationship between the energy transferred by a wave and the amplitude of the wave?

Answer 47

The energy transferred by a wave is proportional to the amplitude squared of the wave.

E = kA²

or

E₁ / A₁² = E₂ / A₂²

Question 48

State what is meant by an object undergoing ‘simple harmonic motion’.

Answer 48

The restoring force (on the object) is directly proportional to, but opposite in direction to, the object’s displacement from its equilibrium position.

Question 49

How could you calculate the optical path difference if you know the optical path lengths of two coherent waves?

Answer 49

Optical path difference is the difference between the two optical path lengths.

Question 50

Any periodic (repeating) waveform can be created by adding a combination of sine and cosine waves together.

What is the name given to the process of adding waves together?

Answer 50

Superposition of waves

Question 51

What does the photoelectric effect tell us about the particulate nature of light?

Answer 51

• Photo-emission will not happen underneath a ‘threshold frequency’ of radiation (f₀) no matter the irradiance.
• If the light was, as thought classically, one continuous wave, then eventually the electrons would absorb enough energy to escape the material, even at smaller frequencies.
• Each individual photon must have enough energy to release an electron- the photon’s energy must be quantised.

Question 52

The de Broglie wavelength of a particle can be considered to be the same as the uncertainty in its position (Δx).

Considering this, explain what conditions are required to create diffraction patterns from beams of particles by a double slit.

Answer 52

The distance between the slits needs to be approximately equal to, or smaller than, the particle’s de Broglie wavelength, as there is an uncertainty to which slit the particle will travel through.

If the slits are a greater distance apart, the particles will only pass through one slit and no diffraction pattern will be observed.

Question 53

A polariser and analyser combination can be used to increase or decrease the transmission of a light wave.

What would be observed if a laser beam was shone through a polariser and then an analyser which were at 90 degrees to each other?

Answer 53

There would be no light transmission through the polariser and analyser combination.

Question 54

In the early 1900’s, experimental evidence suggested that certain phenomena could not be explained using classical physics theory.

State one experimental observation that cannot be explained by classical physics.

Answer 54

• Bohr model of the atom and energy levels for electrons.
• Emission and absorption spectra produced by elements in gaseous form.
• The photoelectric effect
• Black body radiation curves/ultraviolet catastrophe

Question 55

Considering the circular motion of a charged particle in a magnetic field, derive an expression for the radius of the particle’s circular motion.

Answer 55

The force exerted by the magnetic field is considered to be a centripetal force, and so:

qvB = mv²/r

rearranging this obtains

r = mv/qB

Question 56

State the Heisenberg uncertainty principle.

Answer 56

Heisenberg’s uncertainty principle states that the precise position of a quantum particle and it’s momentum cannot both be known at the same instant.

Question 57

On a stationary wave, what is meant by the term antinode?

Answer 57

A point on the stationary wave pattern where there is maximum disturbance/maximum displacement.

Question 58

What is the relationship between geometrical path length and optical path length?

Answer 58

Optical path length = n x geometrical path length.

Question 59

The direction of the force exerted on a negatively charged particle entering a magnetic field perpendicularly can be determined using the ‘right hand rule’.

Describe the use of the ‘right hand rule’ by determining which ‘finger’ represents which quantity.

Answer 59