Pythagoras' Theorem - Topic 1

Question 1

Find the hypotenuse

Answer 1

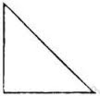
It’s opposite the right angle (It’s the longest side)

Question 2

Find the length of the hypotenuse with sides of 8cm and 6cm

Answer 2

a^2 = b^2 + c^2

a^2 = 8^2 + 6^2

a^2= 64 + 36

a^2 = 100

a = square root of 100

a = 10

Question 3

Find the shorter side of a triangle with the hypotenuse of 20cm and 1 side of 16cm.

Answer 3

a^2 = b^2 + c^2

20^2 = 16^2 + c^2

c^2 = 20^2 - 16^2

c^2 = 400 - 256

c^2 = 144

c = square root of 144

c = 12

Question 4

The slant height of a cone is 15cm and the base radius is 5cm. Find the height of the cone. (3sf)

Answer 4

a^2 = b^2 + c^2

15^2 = 5^2 + c^2

c^2 = 15^2 - 5^2

c^2 = 225 - 25

c^2 = 200

c = square root of 200

c = 14.1 cm

Question 5

Find if this triangle is right angled by using the converse of Pythagoras’ Theorem.

It has a hypotenuse of 6cm.

It’s sides are 3cm and 5cm.

Answer 5

a^2 = 6^2

a^2 = 36

b^2 + c^2 = 3^2 + 5^2

b^2 + c^2 = 9 + 25 = 34

By the converse of Pythagoras’ Theorem, this triangle is not right angled.

a^2 =/= b^2 + b^2

Question 6

O is the centre of the circle and AB is a chord of length 14.4cm. The radius of the circle is 9cm. Calculate the distance of the chord from the centre of the circle.

Answer 6

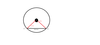
a^2 = b^2 + c^2

9^2 = 7.2^2 + c^2

c^2 = 9^2 - 7.2^

c^2 = 81 - 51.84

c^2 = 29.16

c = square root of 29.16

c = 5.4 cm