Mathematical relationships in anaesthesia and ICU

Question 1

What is a logarithm?

Answer 1

An inverse function, which is the inverse of the exponential function

Question 2

What is a linear function?

Answer 2

y = mx + c

m is the gradient of the line and c represents the y-axis intercept

Question 3

Give an example of a linear relationship.

Answer 3

• the polarographic Clark electrode produces an oxygen-dependent current and calibration is the process of relating this output of a transducer to what is to be measured
• the measured current (y) should vary linearly with blood O2 partial pressure (x)
• the best fit straight line can be determined from the calibration and gives values for m and c
• c is called the intercept and respresents the “zero-offset” of the electrode
• the sensitivity of the electrode to O2 determines the gradient of the calibration line given by m
  
  • increasing the value of m steepens the line

Question 4

What is the advantage and disadvantage of two point calibration?

Answer 4

It’s fast but may be error prone and full calibration usually uses more points

Question 5

What is a parameter?

Answer 5

A number which completely describes a relationship

Question 6

What is a hyperbolic function?

Answer 6

WHen two variables are oppositely related - as one increases the other decreases

y = 1 / x

This will make a rectangular hyperbola

Question 7

Give an example of a hyperbolic relationship

Answer 7

Boyle’s Law - the pressure (P) and volume (V) of a quantity of ideal gas varies reciprocally if the pressure is constant.

P = constant / V

The constant depends on the absolute temperature and number of gas particles.

The curves approach the axes but never cross them. These are called “asymptotes”

Question 8

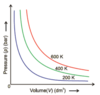
Name some important hyperbolic relationships

Answer 8

• Boyle’s Law - pressure and volume vary reciprocally
• serum creatinine and GFR
• alveolar CO2/end tidal CO2 and alveolar ventilation (for constant PaCO2 and CO)

Question 9

What is an exponential function?

Answer 9

Exponentials are functions where a number, b, (called the base) is raised to a power:

y = b^x

The curves pass through the y-axis but the x-axis is another example of an asymptote

Question 10

What is the number e?

Answer 10

2.7182…

It’s an important base because exponentials with base e are common in problems where the rate at which a quantity changes itself depending on that quantity

(eg radioactive decay, drug kinetics, oscillation, damping)

Question 11

What is a decaying exponential?

Answer 11

It’s when -x is substituted for x in the exponential relationship

y = b^-x

Eg wash out of drug from a single compartment

Question 12

What is the elimination rate constant?

Answer 12

It’s the rate of decay (k)

Question 13

What is the time constant?

Answer 13

The time taken for the concentration to decrease to ~37% (1/e) of the starting value

Question 14

What is the half life?

Answer 14

The time taken for the concentration to halve.

Question 15

What is a logarithm?

Answer 15

It is the exponential function reflected in the 45 degree line y = x.

So if the exponential ( y = b^x)crosses the vertical axis y = 1, the logarithm ( y = log_b(x) ) will cross the horizonal axis at x = 1

Question 16

What is log (AB) equivalent to?

Answer 16

Log (AB) = log A + log B

Question 17

What is log (A/B) equal to?

Answer 17

Log (A/B) = log (A) - log (B)

Question 18

What is the equation for first order kinetics?

Answer 18

Rate at which drug is eliminated is directly proportional to plasma concentration, C

rate of elimination of drug (_{<u>Mass eliminated</u>}) = ßC

^time

Where ß is the rate constant we have seen before.

Question 19

What is the equation for zero-order kinetics?

Answer 19

This is where the elimination mechanism becomes saturated so elimination is independent of C (plasma concentration)

rate of elimination of drug (mass eliminated / time) = γ

Where γ is a constant. Since rate of change is constant, the concentration will decrease linearly rather than exponentially.