Inventory Management Flashcards
(12 cards)
1
Q
Reasons to (not) hold inventory
A
- Why to hold inventory:
- meet predictable variability in demand
- provide safeguard against unpredictable variability in demand
- deal with variability in production or service operations
- take advantage of economies of scale in production/logistics
- Why not:
- costly: storage cost, spoilage cost, opportunity cost of capital, insurance costs
- risky: obsolescence, over-supply
- substitutable: by information or capacity
- hides problems in a sea of inventory
2
Q
Inventory cost components
A
- Purchase costs (per unit), incl. order size discounts
- Ordering costs (per order): paperwork etc.
- Holding costs (per unit per time): warehousing, insurance… ≈ 15-35% of actual unit value per annum
- Shortage/stockout costs (?): missed sales, costs of rush order…
3
Q
Inventory Management: ABC Analysis
A
- Different items require different amount of management’s attention, based on their “value” to the organization:
- A: 20% of items - 80% of value: require frequent monitoring and management via advanced inventory management techniques
- B: 30% of items, 10-20% of value: require less frequent monitoring and less advanced techniques
- C: 50% of items, 5-10% of valie: require infrequent monitoring and easy-to-implement techniques
4
Q
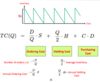
Economic Order Quantity Model
w/ instant lead time
Total Cost
A
- D = Demand rate (number of orders / year)
- Q = Order quantity (units)
- S = Setup costs or cost per order (€/order)
- H = Holding costs per year (€/unit/year); sometimes they are i*C
- C = Cost of purchasing a unit (€/unit)
Objective => minimize total costs so that TC’(Q)=0
Q* = sqrt [(2*D*S) / H]
If there is lead time, reorder as soon as inventory = D*L, also dem demand, der während der lead time herrschen wird
5
Q
EOQ Insights
A
- Order size Q and average inventory (Q/2) are directly related
- trade-off between ordering and holding costs
- The total cost curve is “flat” near the optimum
- EOQ is robust
- EOQ is driven by cost minimization: not always best
6
Q
Continuous Review & Periodic Review
Pros & Cons
A
Continuous review:
- Pros:
- knowledge of an item’s inventory levels at all times
- less inventory needs to be kept at hand
- Cons:
- costs of monitoring inventory levels
Periodic review:
- Pros:
- review periods are known in advance
- allows for better coordination across products and management of ressources
- Cons:
- Requires keeping more inventory on hand to achieve similar service level
7
Q
Continuous Review
Fixed Order Quantity Model: Safety Stock
A
- Order Quantity: get it from EOQ => Q* = sqrt(2DS/H)
- Reorder point ROP = D * L + SS
- Safety stock provides cushion against demand variability
- Shortage occurs when Demand over Lead time > ROP
- Factors that influence Safety Stock
- Demand variability +
- Service level (self chosen) +
- Delivery Lead time +
- SS = z * σL = z * σD * sqrt(L)
- z=NORMSINV(service level)
- σL = σD [std. of daily demand] * sqrt(L)

8
Q
Risk Pooling to reduce Safety Stock
A
- Idea: Supplyng independent demand streams from one location rather than multiple locations requires less safety stock
- Tool: Adding independent and identically distributed random variables:
- Mean Σµi
- Std. Dev.: sqrt( Σ(σi2)
- Example:
- 4 Locations, each daily demand of µ=100, std. dev=50
- 1 centralized location: µ=400, std. dev=sqrt(4*250)=100
- Benefits:
- Cost savings are proportional to the square root of the number of locations pooled!
- Reduces safety stocks: better service for same total inventory level OR same service for a smaller total inventory
9
Q
Periodic Review + Order-up-to-Level
A
- Order up to a predefined level on predefined time intervals
- Exposure period = T [Review Period] + L [Lead Time]
- time between placing order n and receiving order n+1
- I+q is the order-up-to-level
- Safety Stock:
- z * σL+T [Std. dev of demand during exposure period]
- Current Inventory = I
- Target Inventory
= D * (L + T) + z * σL+T - Order Quanity
= D * (L + T) + z * σL+T - I
= Target - Current Inventory - Average Cycle Stock:
- (D * T) / 2
10
Q
Newsvendor Model
A
- Based on marginal analysis: with what probability will the 88th newspaper be sold/not sold and how does that affect profits?
- Buy the Q+1st unit, as long as
- Loss < Gain (or)
- P(D≤Q) * Co < P(D>Q) * Cu (or)
- P(D≤Q) < Cu / (Cu + Co)
- Stop at P(D≤Q*) ≥ Cu / (Cu + Co)
- Service Level = Critical Ratio
- For normal demand:
- Q* = µ + z * σ
- with z = NORMSINV(Cu / (Cu + Co))
11
Q
Newsvendor Application: Revenue Management
A
- Technique to maximize revenue by matiching fixed supply with uncertain demand
- When to use it:
- fixed inventory/capacity which is expensive or impossible to store
- inventory/capacity is committed to a customer before all demand is known
- different customer segments can be price discriminated
- same unit of inventory can satisfy different customer segments
- Calculate Cu and Co
12
Q
Inventory: functional classifications
A
- Cycle inventory: inventory being shipped to different production stages
- Safety inventory: against demand fluctuations & peaks
- Decoupling inventory: buffers against blocks & starvation
- seasonal inventory
- pipeline inventory: products inside production
- speculative inventory: against cost increases for inputs


