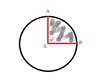
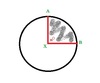
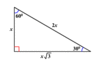
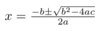Handy Things to Remember

Question 1

An integer is divisible by 2 if…

Answer 1

…its last digit is divisible by 2.

Question 2

An integer is divisible by 3 if…

Answer 2

…its digits add up to a multiple of 3.

Question 3

An integer is divisible by 4 if…

Answer 3

…its last two digits are a multiple of 4.

Question 4

An integer is divisible by 5 if…

Answer 4

…its last digit is 0 or 5.

Question 5

An integer is divisible by 6 if…

Answer 5

…if it is divisible by 2 and 3. (Last digit divisble by 2, Digits add up to a multiple of 3.)

Question 6

An integer is divisible by 9 if…

Answer 6

…its digits add up to a multiple of 9.

Question 7

1/20 –> Percent

Answer 7

5% –> Fraction

Question 8

1/2 –> Percent

Answer 8

50% –> Fraction

Question 9

1/12 –> Percent

Answer 9

8 1/3% –> Fraction

Question 10

3/5 –> Percent

Answer 10

60% –> Fraction

Question 11

1/10 –> Percent

Answer 11

10% –> Fraction

Question 12

5/8 –> Percent

Answer 12

62 1/2% –> Fraction

Question 13

1/8 –> Percent

Answer 13

12 1/2% –> Fraction

Question 14

2/3 –> Percent

Answer 14

66 2/3% –> Fraction

Question 15

1/6 –> Percent

Answer 15

16 2/3% –> Fraction

Question 16

1/5 –> Percent

Answer 16

20% –> Fraction

Question 17

1/4 –> Percent

Answer 17

25% –> Fraction

Question 18

3/10 –> Percent

Answer 18

30% –> Fraction

Question 19

1/3 –> Percent

Answer 19

33 1/3% –> Fraction

Question 20

3/8 –> Percent

Answer 20

37 1/2 % –> Fraction

Question 21

2/5 –> Percent

Answer 21

40% –> Fraction

Question 22

7/10 –> Percent

Answer 22

70% –> Fraction

Question 23

3/4 –> Percent

Answer 23

75% –> Fraction

Question 24

4/5 –> Percent

Answer 24

80% –> Fraction

Question 25

5/6 --\> Percent

Answer 25

83 1/3% --\> Fraction

Question 26

7/8 --\> Percent

Answer 26

87 1/2% --\> Fraction

Question 27

9/10 --\> Percent

Answer 27

90% --\> Fraction

Question 28

11/12 --\> Percent

Answer 28

91 2/3% --\> Fraction

Question 29

Percent Increase

Answer 29

Increase (100%) / Original --- Increase is New-Original.

Question 30

Percent Decrease

Answer 30

Decrease (100%) / Original --- Decrease is New-Original.

Question 31

Factor a^2 - b^2

Answer 31

(a+b)(a-b)

Question 32

Factor a^2 + 2ab + b^2

Answer 32

(a+b)(a+b)

Question 33

Factor a^2 - 2ab + b^2

Answer 33

(a-b)(a+b)

Question 34

Define MEDIAN

Answer 34

the middle term in a group of terms that are arranged in numerical order

Question 35

Define MODE

Answer 35

the term that appears most frequently in a set of numbers

Question 36

Combination Formula

Answer 36

.... where *n* is the number of items in the group as a whole & .... where *k* is the number of items in each sub-group formed

Question 37

Give an example of a Permutation

Answer 37

Ex: for a set containing A, B, and C, the possible permutations for three-letter combinations are: ABC, ACB, BCA, BAC, CAB, CBA

Question 38

How many PERMUTATIONS (arrangements, orders, ways) are possible given set X?

Answer 38

\_ _ \_ _ for the number of spaces in the result becomes (Total)(Total-1)(Total-2)(Total-3) until all of the _ \_ _ \_ spots are taken

Question 39

Probability

Answer 39

Number of desired Outcomes ----------------------------------------------- Number of possible Outcomes

Question 40

Probability of Event NOT Happening

Answer 40

1 - (prob of event happening)

Question 41

Probability of Dependent Events

Answer 41

(probability of 1st event)\*(probability of 2nd event)

Question 42

Area of Parallelogram

Answer 42

Area = base\*height

Question 43

Value of π

Answer 43

3.14

Question 44

Perimeter of a Circle

Answer 44

Circumference -------------------- Diameter

Question 45

Circumference of a Circle

Answer 45

C = 2 π r

Question 46

Define Central Angle of a Circle

Answer 46

the central angle is the angle formed in the center of the circle by two radii (in example, AXB would be the central angle)

Question 47

Define ARC of a circle

Answer 47

the arc is asection of the circumference of the circle (Ex: Arc AB)

Question 48

Arc Length

Answer 48

Arc Length = Circumference \* (n/360)

Question 49

Define Sector of a Circle

Answer 49

a sector is the portion of a circle's interior that is bounded by two radii and an arc (Ex: sector is shaded grey)

Question 50

Area of a Circle

Answer 50

A = π r²

Question 51

Area of a Sector

Answer 51

Area of a Sector = (n/360)\*(Area of Circle) = (n/360)\*π\*r²

Question 52

Define Chord of a Circle

Answer 52

a line segment that joins two points on the circle the longest chord of a circle is its diameter

Question 53

Define Tangent of a Circle

Answer 53

a line that touches only one point on the circumference of the circle a line drawn tangent to a circle is perpendicular to the radius at the point of tangency

Question 54

Slope | (m)

Answer 54

slope = rise/run | (slope = deltaY/deltaX)

Question 55

Equation of a straight line

Answer 55

y = mx + b m: slope=rise/run b: the value of y where x=0 (y-intercept)

Question 56

Volume of a Rectangular Solid (Cube or Box)

Answer 56

Volume = length\*width\*height

Question 57

Surface Area of a Rectangular Solid (Cube or Box)

Answer 57

Surface Area = Sum of Areas of Faces = 2lw+2lh+2wh

Question 58

Volume of a Cylinder

Answer 58

volume of a cylinder = (area of base)(height) = πr²h

Question 59

Lateral Surface of a Cylinder

Answer 59

Lateral Surface is the surface of the cylinder that is NOT the bases. Lateral Surface = (Circumference of Base)\*(Height) =2πrh

Question 60

Total Area of a Cylinder

Answer 60

total Area of a Cylinder = (area of base) + (area of lateral surface) = 2\*πr²+2πrh

Question 61

Give 3 examples of Pythagorean Triples (three whole numbers that satisfy the Pythagorean Theorem)

Answer 61

3, 4, 5 6, 8, 10 5, 12, 13

Question 62

Pythagorean Theorem

Answer 62

c² = a² + b² ...where c =hypotenuse ....where a=leg ...where b=leg

Question 63

Quadratic Equation

Answer 63

Question 64

What are the lengths of the hypotenuse and other leg of a 30-60-90 triangle? (shortest leg is x)

Answer 64

Question 65

What is the length of the hypotenuse in a 45-45-90 traingle?

Answer 65