Half life

Question 1

As an isotope decays, the number of nuclei of that isotope that remain will ___________.

As a consequence of this, the _________ of that isotope will also decrease over time

Answer 1

Decrease

Activity

Question 2

The half-life of an isotope

Answer 2

is the time taken for the activity of that isotope (or the number of original nuclei) to drop to half of its initial value

Every time one half-life passes, the activity (and the number of nuclei) will fall by half

Question 3

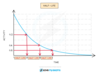
Every time one half-life passes

Answer 3

the activity halves

or

the number of radioactive nuclei half.

Question 4

The activity (and number of nuclei) will never quite drop to zero

(T/F)

Answer 4

True

Question 5

An isotope has an initial activity of 120 Bq.
6 days later it’s activity is 15 Bg.

The number of half-lives that have passed is:

Answer 5

120/2 = 60

60/2 = 30

30/2 = 15

We had to halve 120 three times to get to 15, and so three half-lives have passed.

Therefore each half-life must be:

6 days/3 = 2 days

Question 6

Background Radiation

Answer 6

Background radiation is radiation that is always present in the environment around us

As a consequence, whenever an experiment involving radiation is carried out, some of the radiation that is detected will be background radiation

When carrying out experiments to measure half-life, the presence of background radiation must be taken into account

Question 7

Accounting for background radiation in half-life calculations

Answer 7

Start by measuring background radiation (with no sources present) – this is called your background count

Then carry out your experiment

Subtract the background count from each of your readings, in order to give a corrected count

The corrected count is your best estimate of the radiation emitted from the source, and should be used to measure its half-life